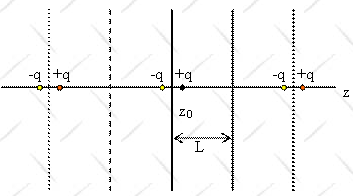The method of images
Infinite conducting plane
Problem:
Two parallel metallic plates (infinite extension) are kept
at potentials Φ = 0 and are located at z = 0 and z = L. A point charge q is at
z0. What is the electrostatic potential between the plates?
Solution:
- Concepts:
Method of images: use infinitely many image charges
- Reasoning:
This problem involves a point charge in front of conducting planes.
- Details of the calculation:
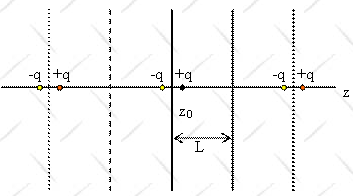
We need image charges -q at z = 2nL - z0, n = integer.
We need image charges +q at z = 2nL + z0, n = non-zero integer.
The potential between the plates is due to the charge q and all the image
charges.
Φ(ρ,z) = [q/(4πε0)]∑n=-∞∞[(ρ2
+ (z-z0-2nL)2)-½ - (ρ2 + (z+z0-2nL)2)-½].
Problem:
A charge q is released
from rest a distance d from an infinite conducting plane. How long will
it take for the charge to strike the plane?
Solution:
- Concepts:
The method of images
- Reasoning:
This problem involves a point charge in front of a conducting plane.
The method of images yields the potential energy of the point charge and the
force on the point charge and Newton's second law yields the equation of
motion. Energy conservation immediately gives us the first integral.
- Details of the calculation:
If the charge is a distance z from the conducting plane, it is a distance 2z
from its image. The force on the charge is Fz = -q2/(16πε0z2),
and its potential energy (the negative of the work necessary to remove the charge from its
position to infinity) is U = -q2/(16πε0z).
When a point charge moves from z = d to z = z', its potential energy changes
by ΔU = U(z') - U(d). A point charge
released from rest at z = d therefore has kinetic energy ½mv2 = [q2/(16πε0)][1/z'
- 1/d] at z'.
|v(z)| = |dz/dt| = [q2/(8mπε0)]½[1/z
- 1/d]½.
T = ∫0Tdt = -[q2/(8mπε0)]-½∫d0dz
[1/z - 1/d]-½
= ((8mπε0d)/q2)½∫0ddz
(z/(d - z))½
= (π/q)(2mπε0d3)½.
[Let z = dsin2θ, dz = 2dsinθcosθdθ,
√z= √(d) sinθ, √(d - z)= √(d) cosθ,
∫0ddz (z/(d - z))½ = 2d∫0π/2
sin2θ dθ = 2dπ/4.]
T is the time it will take for the charge to strike the plane.
Problem:
A particle of mass m and charge e is suspended on a string of length L. At a
distance d (d > L) under the point of suspension there is an infinite plane
conductor. Ignore gravity. Compute the frequency of the pendulum if the
amplitude is sufficiently small such that Hooke's law is valid. (Ignore
radiation losses).
Solution:
- Concepts:
The method of images
- Reasoning:
This problem involves a point charge in front of a conducting plane. The
method of images yields the potential energy of the point charge and the
force on the point charge, and Newton's second law or Lagrange's equations
yield the equation of motion.
- Details of the calculation:
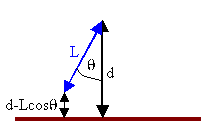
T = ½mv2 = ½mL2(dθ/dt)2, U =
-(1/(16πε0)(q2/(d - Lcosθ)), Lagrangian = T - U.
[If the charge is a distance z from the conducting plane, it is a distance
2z from its image. The force on the charge is Fz = -q2/(16πε0z2),
and its potential energy (the work necessary to move the charge from
infinity to its position) is U = -q2/(16πε0z).]
mL2d2θ/dt2 = -(q2/(16πε0))[Lsinθ/(d
- Lcosθ)2] is the equation of motion.
For small θ we have cosθ ~ 1, sinθ ~ θ.
d2θ/dt2 = -q2θ/(16πε0mL(d - L)2).
We have Hooke's law. The frequency for small oscillation is ω.
ω2 = q2/(16πε0mL(d - L)2).
A different approach:
Using Newton's second law we have Fz = -q2/(16πε0z2)
= -q2/(16πε0(d - Lcosθ)2).
The component in the direction of θ is Fθ = -q2sinθ/(16πε0(d
- Lcosθ)2).
This is the net force, since the component perpendicular to the direction of
θ is canceled by the tension in the string.
F = ma, Fθ = -q2sinθ/(16πε0(d
- Lcosθ)2) = mLd2θ/dt2.
In the small angle approximation we retain only terms to first order in θ,
cosθ ~ 1, sinθ ~ θ.
mLd2θ/dt2 = -q2θ/(16πε0(d - L)2), dθ/dt = -q2θ/(16πε0mL(d
- L)2).
Problem:
Two grounded conducting infinite half-planes meet at an angle of 60o
as shown. A point charge q is located at position (r, 0 < θ < 60o).
The potential in the region between the planes can be found using the method of
images. How many image charges are needed and what are their positions?
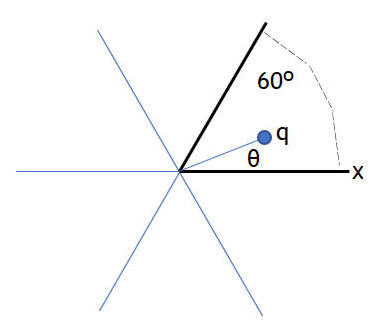
Solution:
- Concepts:
Method of images, the uniqueness theorem
- Reasoning:
Add image charges as shown in the figure. We need 5
image charges. This
configuration will keep the planes at ground potential. The uniqueness
theorem guaranties that this is the only solution.

Let plane 1 be the plane at 0o and plane 2 the plane at 60o.
Proceed from (a) through (f),
| Keep plane 1 at ground potential |
|
Keep plane 2 at ground potential |
| (a) Place -q at (r, -θ) |
|
(b) Place -q at (r, 2*60 - θ) = (r, 120 - θ) |
| (c) Place +q at (r, -2*60 + θ) = (r, 240 + θ) |
|
(d Place +q at (r, 2*60 + θ) = (r, 120 + θ) |
| (e) Place -q at (r, -2*60 -θ) = (r, 240 - θ) |
|
(f) Place +q at (r, -2*60 + θ) = = (r, 240 - θ) |
(e) and (f) place need the same image charge at the same position.
(Note: If the angle between the planes is 360o/n, n even
integer ≥ 4, and a point charge is located at (r, θ) between the planes, then,
if we place an image charge at (r,-θ) and require rotational symmetry for
rotation through angles of 2*360o/n, the resulting charge
distribution keeps the planes at ground potential. We need n-1 image
charges.)
Conducting spheres
Problem:
(a) A point charge q is located a distance d away from the center of a
grounded conducting sphere of radius a. Does the electrostatic force
between the charge and the sphere pull these two objects together or push them
apart. Why?
(b) Now suppose the conducting sphere is not grounded
and carries no net charge. Does the electrostatic force pull the two
objects together or push them apart? Why?
Is the magnitude of the force
greater or less than the force between the charge and the grounded sphere?
Why?
Solution:
- Concepts:
The method of images
- Reasoning:
Consider a charge q at z = d on the z - axis.
Assume a
grounded conducting sphere of radius R is centered on the origin. Then
placing an image charge q' = -qR/d at d' = R2/d on the z-axis
makes the sphere an equipotential with Φ = 0. Add q'' at the center of the
sphere to change the potential or the total charge on the sphere.
- Details of the calculation:
(a) The electrostatic force between the
charge q and the grounded sphere has the same magnitude and direction as the
electrostatic force between the charge q and its image charge q'. It is
attractive.
(b) To keep the net charge on the sphere zero we need two
image charges, q' = -qR/d at d' = R2/d and q'' = qR/d at the
center. The electrostatic force between the charge q and the sphere has the
same magnitude and direction as the electrostatic force between the charge q
and the image charge q' and q''. It is attractive since q' is closer to q
than q''. The magnitude of the force is less than the force between the
charge and the grounded sphere.
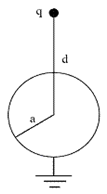
Problem:
Consider a point charge q located a distance d from the center of a grounded,
conducting sphere of radius a < d. Use spherical coordinates and locate
the charge on the z-axis.
(a) Find the potential outside the sphere.
(b) Derive an expression for the induced surface charge density on the
sphere.
(c) Expand the potential in a power series in 1/r for r >> d and keep the
first two terms. What is the significance of these two terms? Find
the electric field to the same order.
Solution:
- Concepts
The
method of images
- Reasoning:
The method of images can be
used to find the potential and field produced by a charge distribution
outside a grounded conducting sphere.
- Details of the calculation:
(a)
Assume that the point charge q is located on the z axis at z = d.
Place an image charge q' = -aq/d on the z-axis at z' = a2/d.
This will keep the sphere at zero potential. The potential in the
region r < a is zero.
The potential in the region r > a is given by
V(r)
= kq/|r - dk| + kq'/|r - z'k| = kq/|r -
dk| - (kaq/d)/|r - (a2/d)k|
= kq/(r2
+ d2 - 2drcosθ)½ - (kaq/d)/ (r2
+ a4/d2 - 2(a2/d)rcosθ)½
=
kq/(r2 + d2 - 2drcosθ)½ - (kq)/(d2r2/a2
+ a2 - 2drcosθ)½.
(b) Surface charge σ = -ε0∂V/∂r|a.
-∂V/∂r = (kq)(r - dcosθ)/(r2 + d2 - 2drcosθ)3/2
- (kq)(d2r/a2 - dcosθ)/(d2r2/a2
+ a2 - 2drcosθ)3/2.
-∂V/∂r|a = (kq)(a - d2/a)/(a2
+ d2 - 2dacosθ)3/2.
σ = ε0(kq)(a- d2/a)/(a2
+ d2 - 2dacosθ)3/2.
(c) V(r)
=
(kq/r)[1/(1
+ d2/ r2 - 2(d/r)cosθ)½
- (a/d)/(1 +
a4/d2r2 - 2(a2/(dr))cosθ)½]
≈ (kq/r)[1
+ (d/r)cosθ) - (a/d)(1 + (a2/(dr))cosθ)]
=
(kq/r)[1 -
(a/d) + [(d/r )- a3/(d2r)]cosθ)]
= (kq/r)[1 - (a/d)] + (kqd/r2)(1-a3/d3)cosθ.
The first term is the potential due to a point charge q[1 - (a/d)] at the
origin.
q[1 - (a/d)] is the sum of q and the induced surface charge on
the sphere.
E = (kqr/r3)[1 - (a/d)] to the same order.
The second term in the potential is due to a dipole
p = qd(1 - a3/d3)k
at the origin.
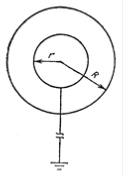
Problem:
Inside a thin-walled metal sphere with radius R = 20 cm there is a metal
sphere with the radius r = 10 cm. The two spheres have a common center. The
inner sphere is connected with a very long wire to the Earth via an opening in
the outer sphere. A charge Q = 10-8 C is placed onto the outer
sphere.
(a) Find an expression for the electrostatic potential everywhere. Neglect the
potential due to the wire. (Hint: Think method of images.)
(b) Find the potential of the outer sphere. Give a numerical answer.
(c) Find the capacitance of the system. Give a numerical answer.
Solution:
- Concepts:
Method of images
- Reasoning:
The inner sphere is a grounded conducting sphere. The potential on the
inner sphere is zero. The potential everywhere outside the inner sphere is
the same as that to a sphere of radius r2/R with uniform surface
charge density and total charge Q' = -Qr/ and a sphere of radius R with
uniform surface charge density and total charge Q.
- Details of the calculation:
(a) V(r < r' < R) = kQ/R + k Q'/r' = kQ(1/R -
r/(Rr')), V(r' > R) = k(Q + Q')r' + k Q'/r' = kQ(1 - r/R)/r'
Here k = 1/( 4πε0).
You can also use the principle of superposition.
Assume there is a charge Q' on the grounded inner sphere.
V(r' > R) = kQ/r' + kQ'/r'
V(r < r' < R) = kQ/R + kQ'/r'
V(r) = kQ/R + kQ'/r = 0 --> Q' = -Qr/R.
(b) V(R) = kQ(1/R - r/R2) = 225 V
(c) C = Q/V = 10-8 C/ (225 V) = 44.4 pF.
Problem:
Compute the force of attraction between a neutral metallic sphere of radius a
and a point charge q positioned a distance r from the center of the sphere,
where r > a.
How does the force of attraction behave as a function of r if r
>> a?
Solution:
- Concepts:
The method of images
- Reasoning:
Orient the coordinate system so that the sphere is
centered at the origin and the point charge q is located at z = r on the
z-axis.
Placing an image charge q' = -qa/r at z' = a2/r on
the z-axis makes the surface of the sphere an equipotential with Φ = 0.
Adding q'' = qa/r at the center of the sphere changes the total charge on
the sphere and makes it neutral while keeping its surface an equipotential.
- Details of the calculation:
The electrostatic force between the
charge q and the grounded sphere has the same magnitude and direction as the
electrostatic force between the charge q and the image charges q' and q''.
It is attractive since q' is closer to q than q''. The magnitude of the
force is less than the force between the charge and the grounded sphere.
|F| = (kq2a/r)[1/(r - a2/r)2 - 1/r2]
= (kq2a/r3)(2a2/r2 - a4/r4)/(1
- a2/r2)2
= kq2(a/r)3(2r2
- a2)/(r2 - a2)2.
Here k = (4πε0).
If r >> a we have |F| ≈ (2kq2a3/r5).
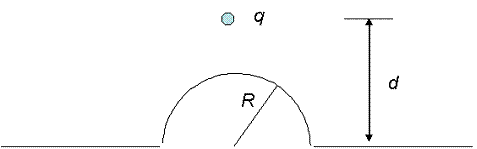
Problem:
A conductor at potential V = 0 has the shape of an infinite plane with a
hemispherical bulge of radius R. A charge q is placed above the center of the
bulge, a distance d from the plane (which means a distance d - R from the top of
the bulge). What is the electrostatic force on the charge?
Solution:
- Concepts:
The method of images
- Reasoning:
The method of images can be used to find the potential and field produced by
a charge distribution outside a grounded conducting sphere and above a
grounded conducting plate. Here we have a combination of these two
geometries.
- Details of the calculation:
Let the z-axis be the symmetry axis. Placing image charges -q at z = -d,
q' = -qR/d at z = R2/d and -q' = qR/d at z = -R2/d on
the z-axis makes the surface of the conductor an equipotential surface. The
field everywhere outside the conductor is the same as that due to q and the
image charges (uniqueness theorem). The force on q therefore is
F = k(-q2/(4d2) + qq'/(d - R2/d)2
- qq'/(d + R2/d)2)k = -kq2(1/(4d2)
+ 4R3d3/(d4 - R4)2)k.
The charge is pulled towards the conductor.
Problem:
An insulated neutral conducting sphere of radius R is centered at the origin.
A point charge q is located on the z-axis at z = 2R.
(a) Find the potential outside the sphere.
(c) For r >> R, expand the potential in a power series in R/r and keep
only terms to up to first order. What is the significance of the terms?
Solution:
- Concepts
The
method of images
- Reasoning:
The method of images can be
used to find the potential and field produced by a charge distribution
outside a conducting sphere.
- Details of the calculation:
(a) For the point charge q is located on the z axis at z =
2R, place an image charge q' = -Rq/(2R) = -q/2 on the z-axis at z' = R2/(2R)
= R/2 and a point charge q/2 at the origin.
This will keep the surface of the sphere an equipotential surface and the sphere
neutral. The potential in the
region r < R is constant.
The potential in the region r > R due to the charge and its image charges is given by
V(r)
= kq/|r - 2Rk| - k(q/2)/|r - (R/2)k| + kq/(2r)
= kq/(r2
+ 4R2 - 4Rrcosθ)½ - (kq/2)/(r2
+ R2/4 - Rrcosθ)½ + ½kq/r
= (kq/r)(1 + 4(R/r)2 - 4(R/r)cosθ)-½ - (kq/2r))(1
+ (R/r)2/4 - (R/r)cosθ)-½ + kq/(2r)
(b) To first order in R/r:
(1 + 4(R/r)2 - 4(R/r)cosθ)-½ = 1 + 2(R/r)cosθ.
(1 + (R/r)2/4 - (R/r)cosθ)-½ = 1 + ½(R/r)cosθ.
V(r) = (kq/r)[1 + (7/4)(R/r)cosθ] = kq/r + k/r2(7qRcosθ/4)
The first term is the potential due to a point charge q at the
origin.
The second term in the potential is due to a dipole
p = 7qR/4 k
at the origin.
Conducting cylinders
Problem:
The ultimate goal of this problem is to determine the capacitance of a
straight telephone wire suspended at a fixed height h above at ground surface. The length of the wire is L, the radius of the wire is a, and the ground
potential is equal to zero.
(a) As a first step, determine the capacitance of a system consisting of two
parallel straight wires. The length of each wire is L, the radius of each wire
is a, and the distance between the wires is 2h. Assume that L >> h >> a. You
can assume that the charges on the upper and lower wires are q and -q,
respectively.
(b) Using the result of part (a) and symmetry arguments, find the
capacitance of the telephone wire described in the beginning of the problem.
Solution:
- Concepts:
Gauss' law, method of images
- Reasoning:
The electric field outside an infinitely long straight wire with charge per
unit length λ points radially away from the wire and has magnitude E = λ/(
2πε0r). (Gauss' law)
The electric field and potential due to a single wire with charge per unit
length λ a distance h from an infinite grounded conducting plane is found using
the method of images.
- Details of the calculation:
(a) Assume two wires of charge density λ and -λ parallel to the y-axis at
x = 0, z = h and x = 0, z = -h, respectively.
The electric field along on the z-axis between the wires is
E = λ/(2πε0(z - h)) - λ/(2πε0(z + h)).
The potential difference between the wires is
ΔΦ = -∫-h+ah-aEdz = [λ/(2πε0)]∫-h+ah-a(1/(z - h) -
1/(z + h))dz
= 2[λ/(2πε0)]∫0h-a(1/(z - h))dz
= -2[λ/(2πε0)]∫2h-aa(1/z')dz' = [λ/(πε0)]ln(2(h
- a)/a),
with z' = h - z.
If h >> a we have
ΔΦ ≈ [Q/(πε0L)]ln(2h/a).
The capacitance is C = Q/ ΔΦ = πε0L/ln(2h/a)
(b) Let the xy plane be the conducting plane and put the wire at x = 0, z = h.
Then the electric field along on the z-axis between the wire and the plane is
E = λ/(2πε0 (z - h)) - λ/(2πε0 (z + h))
= [λ/(2πε0)]ln(2(h - a)/a).
The potential difference between the wire and the plane is
ΔΦ = -∫0h-aEdz = [λ/(2πε0)]ln(2(h - a)/a) ≈ [Q/(πε0L)]ln(2h/a).
This result is also obvious from symmetry.
C = Q/ΔΦ = 2πε0L/ln(2h/a) .