Lagrangian problems, constrained point masses

Problem:

A bead of mass m slides without friction on a circular loop of radius r.  The loop lies in a vertical plane and rotates about a vertical diameter with constant angular velocity ω.

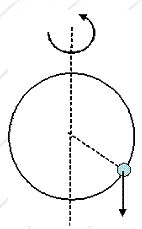
(a)  For angular velocity ω larger than some critical value ω_c, the bead can undergo small oscillations about an equilibrium point θ₀ ≠ 0.  Find ω_c and θ₀(ω).
(b)  Obtain the equations of motion for the small oscillations about θ₀ as a function of ω and find the period of the oscillations.

Solution:

• Concepts:
  Lagrange's equations
• Reasoning:
  Lagrange's equations are the equations of motion.
• Details of the calculation:
  (a)  L = T - U.
  T = ½m(r²(dθ/dt)² + r²sin²θ ω²),  U = -mgr cosθ.
  L = ½m(r²(dθ/dt)² + r²sin²θ ω²) + mgr cosθ.
  Here r and ω are constants. 
  We have only one generalized coordinate. 
  d/dt(∂L/∂(dq_i/dt)) -  ∂L/∂q_i = 0.
  Lagrange's equation yields 
  d²θ/dt² = sinθ cosθ ω² - (g/r) sinθ.
  d²θ/dt²|_θequ = 0 for a stationary point.
  The angles θ = 0, θ = π, or cosθ  = g/(rω²) define stationary points.
  We need g/(rω²) < 1 for the point θ = cos^-1(g/(rω²) to exist.
  The critical frequency ω_c = (g/r)^½.
  (b)  Consider the equilibrium point cosθ₀ = g/(rω²).
  For a stable point we need a restoring force.  Let θ = θ₀ + δ.
  We need d²δ/dt² = -cδ, with c a positive number.
  d²δ/dt² = sin(θ₀ + δ)cos(θ₀ + δ) ω² - (g/r) sin(θ₀ + δ)
  = d(sinθ cosθ ω² - (g/r) sinθ)/dθ|_θ0*δ  (Taylor series expansion)
  = (cos²θ₀ ω² - sin²θ₀ ω² - (g/r) cosθ₀)δ.
  For a stable point we need cos²θ₀ ω² - sin²θ₀ ω² - (g/r) cosθ₀ to be negative.
  But cos²θ₀ ω² - sin²θ₀ ω² - (g/r)cosθ₀ = g²/(r²ω²) - ω² < 0 since g/(rω²) < 1 for the point to exist.
  This point is always stable if it exists.
  The equation of motion for small oscillations about θ₀ is
  d²δ/dt² = -(ω² - g²/(r²ω²))δ.
  The frequency of small oscillations about this point is Ω = (ω² - g²/(r²ω²))^½.
  The period T = 2π/Ω.