Surface tension

Problem:

The surface tension γ is defined as the force along a line of unit length, γ = F/L (N/m).  The force is parallel to the surface and perpendicular to the line.   For an inflated, (1.5 r₀ < r < 2.5 r₀), spherical rubber balloon γ is nearly constant. The surface tension balances the outward force due to the pressure difference between the inside and the outside air.
(a)  For a balloon inflated to a radius r in the range 1.5 r₀ < r < 2.5 r₀ find the pressure difference ΔP = P_i - P_o as a function of r and γ.
(b)  Show that is in a stable equilibrium.

Solution

• Concepts:
  Surface tension
• Reasoning:
  The balloon skin has an inner and an outer surface.  The net force per unit length holding the skin together is 2γ.
• Details of the calculation:
  
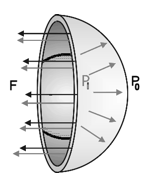
  (a)  Imagine a cut through the balloon as shown.  Consider the right half of the balloon. 
  The net force due to the pressure difference points to the right.  It has magnitude
  F_P = 2πr²∫₀^π/2sinθ dθ ΔP cosθ = 2πr² ΔP ∫₀¹x dx = πr² ΔP.
  The net force due to the surface tension points to the left.  It has magnitude
  F_γ = -2*γ2πr = -4γπr.
  For equilibrium we need F_P + F_γ = 0
  πr² ΔP = 4γπr.  ΔP = 4γ/r.
  
  (b)  In equilibrium we have P_total(r) = -P_atm - 4γ/r + 3nRT/(4πr³) = -a - b/r +  c/r³ = 0, with a, b, and c positive constant.
  P_total(r + Δr) = dP/dr|_rΔr = (b/r² - 3c/r⁴)Δr = (1/r) (b/r + a - a - c/r³ +  c/r³ -  3c/r⁴)Δr 
  = (1/r)(-P_total(r) - a - 2c/r³)Δr = -(1/r)( a + 2c/r³)Δr = -constant*Δr.
  We have a restoring force per unit area, the balloon is stable.