Two spin ½ particles
Problem:
The Heisenberg Hamiltonian representing the "exchange
interaction" between two spins (S1 and
S2)
is given by H = -2f(R)S1∙S2,
where f(R) is the so-called exchange coupling constant and R is the spatial
separation between the two spins. Find the eigenstates and eigenvalues of the
Heisenberg Hamiltonian describing the exchange interaction between two
electrons.
HINT: The total spin operator is S =
S1
+ S2.
Solution:
- Concepts:
The state space of two spin-½
particles
- Reasoning:
We have to diagonalize the
matrix of H in the state space of two spin ½ particles
- Details of the calculation:
S1∙S2 = ½(S2 - S12
- S22). The eigenstates of S1∙S2 are the singlet state and the triplet
states, {|S, Ms>}, S = 0, 1.
In that basis H is diagonal.
For the singlet state we have S1∙S2 |0, 0> = -¾ħ2.
For the triplet states we have S1∙S2 |1, Ms> = ¼ħ2.
The eigenstates of H are |0, 0> with eigenvalue (3/2)f(R)ħ2
and |1, 1>, |1, 0>, |1, -1> with eigenvalue -½f(R)ħ2
(3-fold degenerate).
Problem:
Let Si, i =
1, 2 denote the spin vectors of two spin-½ particles. The interaction is given by
H = U0 (S1·S2 − 3 S1zS2z).
Find the energy eigenstates
and eigenvalues.
Solution:
- Concepts:
The state space of two spin-½
particles
- Reasoning:
We have to diagonalize the
matrix of H in the state space of two spin ½ particles
- Details of the calculation:
The eigenstates of S1·S2 are the singlet and the triplet states.
{|S,Sz> = |0,0>,
|1,1>, |1,0>, |1,-1>}
S1·S2|S,Sz>
= ½(S2 - S12 - S22) |S,Sz>
= ½(S(S+1) - 3/2)ħ2|S,Sz>.
The singlet and the triplet
states are also eigenfunctions of S1zS2z.
S1zS2z
|1,1> = S1zS2z |++> = (ħ2/4)|1,1>.
S1zS2z |1,0> = ½½ S1zS2z
(|+-> + |-+>) = -(ħ2/4)|1,0>.
S1zS2z |1,-1> = S1zS2z |--> = (ħ2/4)|1,-1>
S1zS2z |0,0> = ½½ S1zS2z
(|+-> - |-+>) = -(ħ2/4)|0,0>The eigenstates of H are the
the singlet and the triplet states. In that basis H is diagonal.
H |1,1> = U0(1-3) (ħ2/4)|1,1> = -2U0(ħ2/4)|1,1>
H |1,0> = U0(1+3) (ħ2/4)|1,0> = 4U0(ħ2/4)|1,0>
H |1,-1> = U0(1-3) (ħ2/4|1,-1> = -2U0(ħ2/4)|1,1>
H |0,0> = U0(-3+3) (ħ2/4|0,0>
= 0
Problem:
Two spin ½ particles, 1 and 2, are in the singlet state shown below. Here
|+> and |-> refer to spin up and spin down with respect to the z-axis.
|ψ(1,2)> = ½½(|+>1|->2 - |->1|+>2).
(a) What is the probability of measuring the spin of particle 1 along an
axis u(θ,φ), and finding the particle in the state |+>u?
Assume the spin of particle 1 is measured along the z-axis and found to be ħ/2.
(b) A simultaneous measurement of the spin of particle 2 along the z-axis
would yield which results with which probabilities?
(c) A simultaneous measurement of the spin of particle 2 along the x-axis
would yield which results with which probabilities?
Solution:
- Concepts:
Entangled particles
- Reasoning:
Sz and Siz are incompatible observables for this two-particle
system.
- Details of the calculation:
(a) The probability of measuring the spin of particle 1 along
an axis u(θ,φ), and finding the particle in the state |+>u is
½.
(b) A simultaneous measurement of the spin of particle 2 along the
z-axis would yield which -ħ/2 with probability 1.
(c) A simultaneous measurement of the spin of particle 2 along the
z-axis would yield which -ħ/2 or ħ/2, each with probability 0.5.
Problem:
Consider the state space E = E1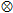 E2
of two non-identical spin ½ particles spanned by the basis vectors {|++>,
|+->, |-+>, |-->}. Use what you know about the common eigenvectors of S2
and Sz, and find the common eigenvectors of S2
and Sx. Express these eigenvectors in terms of the basis
vectors {|++>, |+->, |-+>, |-->}.
E2
of two non-identical spin ½ particles spanned by the basis vectors {|++>,
|+->, |-+>, |-->}. Use what you know about the common eigenvectors of S2
and Sz, and find the common eigenvectors of S2
and Sx. Express these eigenvectors in terms of the basis
vectors {|++>, |+->, |-+>, |-->}.
Solution:
- Concepts:
The state space of two spin ½
particles, change of representation
- Reasoning:
Two eigenbases for the state
space of two spin ½ particles are
{|++>,
|+->, |-+>, |-->},
the common eigenbasis of S1z and S2z
and
{|S,Sz>} = {|1,1>,|1,0>,|1,-1>,|0,0>},
the
common eigenbasis of S2
and Sz. We are to change representation from the eigenbasis of S2
and Sz to the eigenbasis of S2
and Sx.
-
Details of the calculation:
The common eigenfunctions of S2 and Sz
are
|11> = |++>,
|10> = ½½(|+-> + |-+>),
|1-1> =|-->,
|00> = ½½(|+-> - |-+>).
From symmetry we therefore have that the common eigenfunctions of S2
and Sx are
|11>x = |++>x,
|10>x = ½½(|+->x + |-+>x),
|1-1>x = |-->x,
|00>x = ½½(|+->x + |-+>x).
We have
|++>x = ½½(|+> + |->)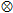 ½½(|+> + |->) = ½(|++> + |+-> + |-+> +
|-->),
½½(|+> + |->) = ½(|++> + |+-> + |-+> +
|-->),
|-->x = ½½(|+> - |->)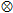 ½½(|+> - |->) = ½(|++> - |+-> - |-+> +
|-->),
½½(|+> - |->) = ½(|++> - |+-> - |-+> +
|-->),
|+->x = ½½(|+> + |->)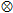 ½½(|+> - |->) = ½(|++> - |+-> + |-+> -
|-->),
½½(|+> - |->) = ½(|++> - |+-> + |-+> -
|-->),
|-+>x = ½½(|+> - |->) ½½(|+> + |->) = ½(|++> + |+-> - |-+> - |-->).
½½(|+> + |->) = ½(|++> + |+-> - |-+> - |-->).
Therefore
|11>x = ½(|++> + |+-> + |-+> + |-->),
|10>x = ½½(|++> - |-->),
|1-1>x = ½(|++> - |+-> - |-+> + |-->),
|00>x = ½½(|+-> - |-+>).
 E2
of two non-identical spin ½ particles spanned by the basis vectors {|++>,
|+->, |-+>, |-->}. Use what you know about the common eigenvectors of S2
and Sz, and find the common eigenvectors of S2
and Sx. Express these eigenvectors in terms of the basis
vectors {|++>, |+->, |-+>, |-->}.
E2
of two non-identical spin ½ particles spanned by the basis vectors {|++>,
|+->, |-+>, |-->}. Use what you know about the common eigenvectors of S2
and Sz, and find the common eigenvectors of S2
and Sx. Express these eigenvectors in terms of the basis
vectors {|++>, |+->, |-+>, |-->}.