Perturbation theory, non-degenerate states

Rigid rotator

Problem:

The equation below defines the eigenvalue problem for a two-dimensional, quantum-mechanical, rigid rotator:
-(ħ²/2I)(∂²/∂θ²)ψ(θ) = Eψ(θ), where ψ(θ + 2π) = ψ(θ).
(a)    What are the energies E_j and wave functions ψ_j(θ) of its lowest three energy eigenstates?
(b)    A perturbation U = β cosθ is added to its Hamiltonian.  Find the effect on the ground state energy, to second order in β.

Solution:

• Concepts:
  The rigid rotator in two dimensions, first and second order perturbation theory.
• Reasoning:
  We are asked to solve the unperturbed problem and find the energy correction for the ground state due to the perturbation to second order.

• Details of the calculation:
  (a)  (∂²/∂θ²)ψ(θ) + (2I/ħ²)Eψ(θ) = 0.
  ψ(θ) = A exp(imθ), E = ħ²m²/(2I).
  where ψ(θ + 2π) = ψ(θ) --> m = 0, ±1, ±2, ...  .
  For m ≠ 0 each energy eigenvalue is two-fold degenerate.  The normalized eigenfunctions corresponding to  E = ħ²m²/(2I) are ψ_±m(θ) = (2π)^-½ exp(±i|m|θ) and linear combinations.  ψ_±m(θ) are eigenfunctions of L_z = (ħ/i)(∂/∂θ) with eigenvalues ±|m|ħ.
  
  (b)  H₀ + U.  U = β cosθ.
  The ground state ψ₀(θ) is not degenerate, E₀⁰ = 0.
  E₁⁰ = <ψ₀|U|ψ₀> = (2π)^-1∫₀^2π β cosθ dθ = 0.
  The first order energy correction is zero.
  
  The second order energy correction for the ground state is
  E₂⁰ = ∑_m≠0 |<ψ_m|U|ψ₀>|²/(E₀⁰ - E₀^m).
  ∫₀^2π exp(imθ) cosθ dθ = 0 unless |m| = 1.
  E₂⁰ = |<ψ₁|U|ψ₀>|²/(E₀⁰ - E₀¹) + |<ψ_-1|U|ψ₀>|²/(E₀⁰ - E₀¹)
  = |<ψ₁|U|ψ₀>|²/(-ħ²/(2I)) + |<ψ_-1|U|ψ₀>|²/(-ħ²/(2I))
  = -(2Iβ²/ħ²)2|(2π)^-1∫₀^2π cos²θ dθ|² = -(Iβ²/ħ²).

Problem:

Suppose the Hamiltonian of a rigid rotator in a magnetic field is of the form
H = AL² + BL_z + CL_y, if terms quadratic in the field are neglected.
Assuming B >> C, use perturbation theory to lowest non-vanishing order to get appropriate energy eigenvalues.

Solution:

• Concepts:
  Stationary perturbation theory
• Reasoning:
  A small correction term is added to a Hamiltonian H₀, whose eigenstates and eigenvalues we can solve for exactly.  We are asked to find the lowest order non-vanishing corrections to the energy eigenvalues.

• Details of the calculation:
  H = AL² + BL_z + CL_y,  B >> C.
  H = H₀ + H', H₀ =  AL² + BL_z.
  The eigenstates of H₀ are {|lm>}.  H₀|lm> = (Al(l+1)ħ² + Bmħ)|lm>
  The eigenvalues are not degenerate.
  The first order corrections are E₁^lm = C<lm|L_y|lm> = -(iC/2)<lm|L₊- L_-|lm> = 0.
  We have to find the second order corrections E₂^lm.
  E₂^lm = ∑_l'm'≠lm C²|<l'm'|L_y|lm>|²/(E₀^lm - E₀^l'm')
  L_y = -(i/2)(L₊- L_-)
  L_±|l,m> = [l(l+1) - m(m±1)]^½ħ|l,m±1>.
  <l'm'|L_±|l,m> = [l(l+1) - m(m±1)]^½ħ δ_l,l' δ_m',m±1.
  E₂^lm = (C²/4)[l(l+1) - m(m+1)]ħ²/(Bħ(m - (m+1))) + (C²/4)[l(l+1) - m(m-1)]ħ²/(Bħ(m - (m-1)))
  = +C²mħ/(2B).
  The energy eigenvalues to second order are Al(l+1)ħ² + Bmħ + C²mħ/(2B).

Infinite well

Problem:

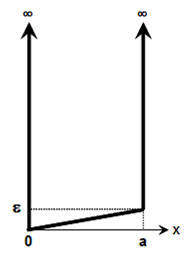
A particle of mass m moves in the potential energy function

U(x) = ∞,  x < 0,  x > a,
U(x) = εx/a,  0 < x < a

where ε is a small perturbation.

Use first-order perturbation theory to find the ground state energy of the particle.

Solution:

• Concepts:
  First order perturbation theory for non-degenerate states
• Reasoning:
  We are asked to solve the unperturbed problem and find the energy correction for the ground state due to the perturbation.
• Details of the calculation:
  H = H₀ + H'.  H₀ is the Hamiltonian of the infinite 1D well, H' = εx/a, 0 < x < a.
  The ground state eigenfunction of H₀ is Φ₀ = (2/a)^½sin(πx/a), the associated eigenvalue is
  E₀ = π²ħ²/(2ma²).
  The first order correction to the ground state energy is
  ΔE₀ = < Φ₀ |H'| Φ₀ > = (2/a)∫₀^a sin²(πx/a)(εx/a)dx = (2ε/π²) )∫₀^π sin²(y) y dy = ε/2.
  Ground state energy to first order:  E = π²ħ²/(2ma²)  + ε/2.

Problem:

A particle of mass m is in an infinite potential well perturbed as shown in the figure.
(a)  Calculate the first-order energy shift of the nth eigenvalue due to the perturbation.
(b)  Write out the first three non vanishing terms for the first-order perturbation expansion of the ground state in terms of the unperturbed eigenfunctions of the infinite well.
(c)  Calculate the second-order energy shift for the ground state.

Solution:

• Concepts:
  First order perturbation theory for non-degenerate states
• Reasoning:
  The ground state of the infinite square well is non-degenerate.

• Details of the calculation:
  (a)  H = H₀ + H'.
  H₀|Φ_n> = E₀ⁿ|Φ_n>.   Φ_n(x) = (2/a)^½sin(nπx/a), E₀ⁿ= n²π²ħ²/(2ma²),  n = 1, 2, 3, ... .
  The eigenvalues of H₀ are not degenerate.
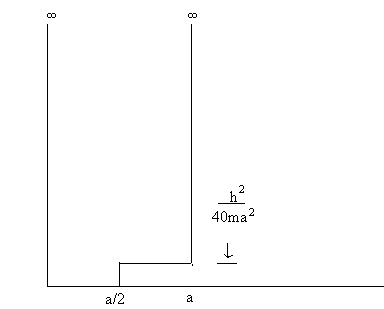
  H'(x) = 0, 0 < x < a/2,  H'(x) = U₀,  a/2 < x < a.  U₀ = h²/(40ma²) = π²ħ²/(10ma²).
  E₁ⁿ = <Φ_n|H'|Φ_n> = (2U₀/a)∫_a/2^a dx sin²(nπx/a) = U₀/2.
  E₁ⁿ = π²ħ²/(20ma²).
  To first order all energies are shifted up by U₀/2.
  
  (b)  First-order perturbation theory yields for the ket
  |ψ₁> = |Φ₁> + ∑_n>1<Φ_n|H'|Φ₁>/(E₀¹ - E₀ⁿ) |Φ_n> ,
  <Φ_n|H'|Φ₁> = (2U₀/a)∫_a/2^a dx sin(πx/a)sin(nπx/a)
  = (2U₀/π)∫_π/2^π dx sin(x)sin(nx)
  = (2U₀/π)[sin((n-1)π/2)/(2n-2) - sin((n+1)π/2)/(2n+2)].
  <Φ_n|H'|Φ₁> = 0 if n = odd.
  <Φ₂|H'|Φ₁> = -4U₀/(3π),  <Φ₄|H'|Φ₁> = 8U₀/(15π).
  E₀¹ - E₀² = -3π²ħ²/(2ma²).  E₀¹ - E₀⁴ = -15π²ħ²/(2ma²).
  |ψ₁> = |Φ₁> + [4U₀/(3π)]/[3π²ħ²/(2ma²)] |Φ₂> - [8U₀/(15π)]/[15π²ħ²/(2ma²)] |Φ₄> + ...
  = |Φ₁> + 8/(90π) |Φ₂> + 16/(2250π)|Φ₄> + ... .

  (c)   E₂¹ = ∑_n>1 |<Φ_n|H'|Φ₂>|²/(E₀¹ - E₀ⁿ)
   = -[4U₀/(3π)]²/[3π²ħ²/(2ma²)] - [8U₀/(15π)]²/[15π²ħ²/(2ma²)] - ...
  = -ħ²/(100ma²)[32/27 + 128/3375 + ...] = second-order energy shift for the ground state.
  The second-order energy shift of the ground state is always negative.

Problem:

A particle of mass m is constrained to move in an infinitely deep, one-dimensional square well extending from -a to +a. 
If this particle is under the influence of a perturbation H' = -Aδ(x), where A is a constant and δ(x) is a delta function at x = 0, calculate the first order corrections to the energy levels. 
What is the condition that A must satisfy if the corrected n* energy level is to have a negative energy?

Solution:

• Concepts:
  First order perturbation theory for non-degenerate states, the infinite square well
• Reasoning:
  The energy level of the 1D infinite square well are non-degenerate.
• Details of the calculation:
  For the unperturbed Hamiltonian we have:
  H = (ħ²/2m)(∂²/∂x²) + U(x),  U(x) = 0 for  |x| < a and U(x) = ∞ for  |x| > a.
  (∂²/∂x²)Φ(x) + (2m/ħ²)EΦ(x) = 0 in the region |x| < a,  Φ(x) = 0 for |x| = a.
  Φ(x) = Cexp(ikx) + Dexp(-ikx),   with E = ħ²k²/(2m).
  Cexp(ika) + Cexp(-ika) = 0,  Cexp(-ika) + Dexp(ika) = 0.
  Two solutions:
  C = D: coska = 0,  ka = nπ/2, n = odd.
  C = -D: sinka = 0,  ka = nπ/2, n = even.
  E_n = n²π²ħ²/(8ma²),  Φ_n(x) = Ncos(nπx/(2a)), n = odd,  Φ_n(x) = Nsin(nπx/(2a)), n = even.
  Normalization:  N² = 1/a.

  Let us calculate the first order energy corrections due to the perturbation.
  n = odd:
  E_n¹ = -AN²∫_-a^a cos²(nπx/(2a))δ(x)dx = -(A/a)
  n = even:
  E_n¹ = -AN²∫_-a^a sin²(nπx/(2a))δ(x)dx = 0.
  E_n* = n²π²ħ²/(8ma²) - A/a if n = odd. We need A > n²π²ħ²/(8ma) for E_n* to be negative.
  E_n* = n²π²ħ²/(8ma²) if n = even.  The perturbation does not change this energy level to first order.