Lasers

A LASER ( Light Amplification by Stimulated Emission of Radiation) is an electromagnetic oscillator which combines light amplification with feedback. The laser uses mirrors to feed the light output from an optical amplifier through a delay (the travel time of the light) back into the amplifier input.

A laser is capable of producing an intense beam of photons having identical scalar and vector properties (frequency, phase, direction and polarization). As a result, a laser beam can be bright, monochromatic, coherent, and unidirectional.

Quantum mechanics predicts that all atomic and molecular systems are characterized by discreet energy levels. These energy levels are the eigenvalues of the Hamiltonian of the system and the corresponding states are its eigenstates. The state corresponding to the lowest possible energy level is termed the ground state and the states corresponding to the other levels are termed excited states. If the energy of the system is measured at any time, the result of the measurement will be one of the energy eigenvalues, and after the measurement the system will be in the corresponding eigenstate.

In a dense medium, such as a solid, liquid, or high pressure gas, frequent collisions between the atoms or molecules cause transitions between energy levels. Optically allowed transitions can also change the energy of the system. An optically allowed transition between energy levels involves the absorption or emission of a photon with frequency f, such that hf = ∆E_if, where ∆E_if is the energy difference between the initial and final energy level and h is Planck’s constant. If the angular momentum quantum number l_i of the initial state and the angular momentum quantum number l_f of the final state differ by 1, i.e. if ∆l = ±1, then a transition involving a photon is very likely. If ∆l ≠ ±1, then a transition involving a photon is much less likely. If no lower lying state satisfying ∆l = ±1 exists, an excited state is called meta-stable. ∆l = ±1 is called a selection rule. If this selection rule is not satisfied, an optical transition is forbidden (unlikely).

An atom or molecule can emit a photon via spontaneous emission or stimulated emission. Atoms and molecules in excited states randomly emit single photons in all directions according to statistical rules via spontaneous emission. In the process of stimulated emission, a photon of energy hf perturbs an excited atom or molecule and causes it to relax to a lower level, emitting a photon of the same frequency, phase, and polarization as the perturbing photon. Stimulated emission is the basis for photon amplification and it is the fundamental mechanism underlying all laser action. The quantum mechanical treatment of stimulated emission is very similar to that of absorption.

Consider the simple case of a two-level system with lower level 1 and upper level 2. Let N₁ be the number density of atoms in level 1 and N₂ be the number density in level 2. Let u(f₁₂) be the energy density per unit frequency interval of the light at frequency f₁₂ = (E₂- E₁)/h.

	The rate of spontaneous emission is independent of u(f₁₂). It is proportional to N₂. R_{spon. emiss.}= A₂₁N₂.
	The rate of stimulated emission depends on u(f₁₂). It is proportional to u(f₁₂)N₂. R_{stim. emiss.}= B₂₁u(f₁₂)N₂.
	The rate absorption depends on u(f₁₂). It is proportional to u(f₁₂)N₁. R_absorb.= B₁₂u(f₁₂)N₁.

The proportional constants A₂₁, B₂₁, and B₁₂ are called the Einstein coefficients.

Simple quantum mechanics predicts B₂₁ = B₁₂ and lets us calculate the value of B₂₁ = B₁₂ using time-dependent perturbation theory. But as long as we treat the electromagnetic field classically, we cannot calculate the probability for spontaneous emission of a photon this way. To calculate A₂₁ we also need to quantize the radiation field.

We can however avoid this problem by making statistical arguments. In a cavity in thermal equilibrium the probabilities that states 1 and 2 are occupied are proportional to the Boltzmann factors exp(-E₁/(kT)) and exp(-E₂/(kT)), respectively, and in equilibrium the probability of up transitions must exactly balance the probability of down transitions.
We have N₁ 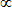exp(-E₁/(kT)), N₂ 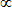exp(-E₂/(kT)),
We therefore need

(A₂₁+ B₂₁u(f₁₂))exp(-E₂/(kT)) = B₁₂u(f₁₂)exp(-E₁/(kT)) = B₂₁u(f₁₂)exp(-E₁/(kT)).
(A₂₁+ B₂₁u(f₁₂)) = B₂₁u(f₁₂)exp((E₂-E₁)/(kT)) = B₂₁u(f₁₂)exp(hf₁₂/(kT)).
A₂₁ = B₂₁u(f₁₂)[exp(hf₁₂/(kT)) - 1].

In a cavity in thermal equilibrium u(f₁₂) is given by Plank's law,
u(f₁₂) = (8πhf³/c³)/[exp(hf₁₂/(kT)) - 1].
We therefore have A₂₁= B₂₁8πhf³/c³.
[Note: I(f,T) = (1/4) u(fT) c = energy radiated per unit time per unit area per unit frequency interval.
I(λ,T) = I(f)|dfν/dλ| = (c/λ²)I(f) = energy radiated per unit time per unit area per unit wavelength interval.]

The rate of absorption and stimulated emission depends on the number of photons present. The rate of spontaneous emission, however, is independent of the number of photons present. Stimulated emission becomes much more likely than spontaneous emission if many photons are present.

The competition between absorption, stimulated emission, and spontaneous emission defines the criteria for laser action.

Consider the simple case of a two-level system with lower level 1 and upper level 2. Let N₁ be the number density of atoms in level 1 and N₂ be the number density in level 2.

In thermal equilibrium N₁> N₂. As a resonant photon is more likely to be absorbed than to stimulate emission. But if N₂> N₁there is the possibility of average overall amplification for an array of photons passing through a volume of atoms of the two-level system. This situation is termed population inversion, since under normal conditions N₁> N₂.

Spontaneous emission depletes N₂, producing unwanted photons with random phases, propagation directions, and polarizations. Because of loss associated with spontaneous emission and other losses associated with the laser cavity, each laser is characterized by a minimum value of N₂- N₁, termed the threshold inversion. Only if N₂- N₁ is greater then the threshold inversion do we see laser action.

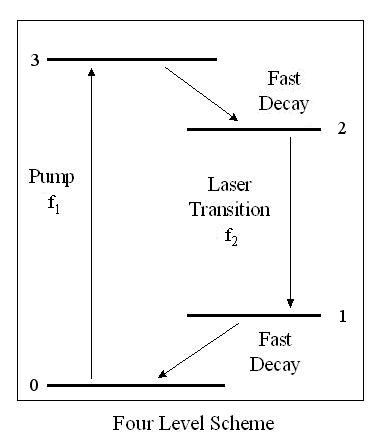
There are several ways of pumping a system to produce a population inversion. It is, however, impossible to optically pump a two-level system, since once N₂= N₁ the induced transition rate equals the absorption rate. Three or four levels are needed to pump the system and produce the population inversion required for laser action. Let us investigate the pumping of a four-level system.

An atom is first excited by optical, electrical, or other means through the “pump transition” from a starting level “0” to a temporary level or group of levels “3”. The atom quickly relaxes to a metastable level “2”. Stimulated emission occurs from level “2” to level “1”. Level “1” quickly decays back to level “0”, so that absorption from level “1” to level ”2” is unlikely. At any given time more of the atoms are at level “2” than at level “1”, there is a “population inversion”. The rate of stimulated emission will exceed the absorption rate resulting in an optical amplifier.

	Solid systems are usually pumped by optical means with intense light. Xenon-filled flashtubes are positioned alongside the lasing medium. A high voltage electric discharge is passed through the flashtubes, causing them to emit an intense flash of white light. This light is partially absorbed by the amplifying medium. A flashlight-pumped laser has a pulsed output.
	Gaseous systems are often pumped by passing an electric discharge through the medium itself. Lasers that are pumped by an electric discharge can produce either a pulsed output or a continuous output depending upon whether the discharge is pulsed or continuous.
	Laser diodes are pumped by passing an electric current through the diode junction.

To sustain laser action it is usually necessary to place the lasing material between the two mirrors of an optical cavity.

Photons are reflected back and forth through the lasing medium, which greatly increases the probability of stimulated emission. Because a coherent beam makes multiple passes through the optical cavity, we observe an interference-induced longitudinal mode structure. Only light whose wavelength satisfies the standing wave condition, mλ = 2L, will be amplified. L is the cavity length and m is a large integer referring to the number of nodes in the standing wave pattern. Out of phase reflections are lost through destructive interference. Spontaneously emitted photons with off-axis velocity components escape from the lasing volume and are not significantly amplified.

The resonant frequencies of a cavity of length L are f_m = c/λ = mc/(2L). The separation between resonance frequencies is ∆f = c/2L. A typical visible wavelength laser resonator might have length L = 30 cm operating at wavelength λ = 600 nm so that m = 1 million. For an L = 30 cm resonator, the spacing between successive resonance frequencies is ∆f = c/2L = 500 MHz while the resonance frequencies are near f_m = c/λ = 500 THz, one million times larger. Non-relativistic quantum mechanics predicts that atomic and molecular systems have stable excited states with discrete, well defined energy eigenvalues. But we observe that all excited state spontaneously decay. The uncertainty principle, ∆E∆t ~ ħ, then requires that the energies of the excited states, and therefore transition frequencies, cannot be known with arbitrary accuracy. We have ∆f∆t ~ 1/(2π). Several of the resonance frequencies f_m may fall into that range ∆f. The spectrum emitted by a laser is a combination of the resonant frequencies f_m that fall into that range ∆f. A representative laser output spectrum is shown below.

Modified laser resonators can suppress all but one of the resonant frequencies, permitting more precise control of the frequency, a desirable feature in scientific and engineering applications

The gain per pass varies greatly between different types of lasers and so do mirror requirements. Pulsed lasers tend to exhibit higher gain per pass than continuous wave (CW) lasers, although the massive population inversion can only be maintained for a small interval of time. A typical helium-neon laser has a single pass gain of the order of 1% and requires high reflectance mirrors (100% and 99%). Lasers that are capable of producing multiple wavelengths from several different lasing transitions require specially optimized mirrors if output at each wavelength is desired.

The radiation field may have nodes and antinodes in the plane perpendicular to the laser axis. The different transverse irradiance patterns are referred to as Transverse Electric and Magnetic (TEM) modes. In an unconfined cavity with cylindrical symmetry, these modes form simple progressions of irradiance functions. Transverse modes are identified by their irradiance distributions and are designated TEM_pq where the subscripts p and q refer to the number of nodes along the two orthogonal axes perpendicular to the laser axis. The irradiance distributions of all the modes are smoothly varying but none has a uniform irradiance distribution.

The lowest order mode, TEM₀₀ has a cylindrical Gaussian irradiance distribution.

This mode experiences the minimum possible diffraction loss, has minimum divergence, and can be focused to the smallest possible spot. For these reasons, it is often imperative that the laser be restricted to operation in this mode. Higher order modes have a larger spread and suffer higher diffraction losses.

Some lasers use an internal tuning element to select various lines. In an argon-ion laser, which has strong output at 488 and 514 nm as well as several other wavelengths, the operational wavelength is selected by a prism within the cavity that is rotated to an angle that provides single-line output.

Various liquid and solid-state lasers have broad bandwidths that cover tens of nanometers. Examples include dye and Ti:sapphire lasers. This has allowed the development of tunable and ultra fast lasers. Creating a tunable CW laser involves including an extra filtering element in the cavity, usually a birefringent filter. This narrows the bandwidth and, by rotating the filter, allows smooth bandwidth tuning.

Lasers can be divided into three main categories, continuous wave (CW), pulsed, and ultra fast. Some materials such as ruby and rare-gas halogen excimers ( ArF, XeCl) sustain laser action for only a brief period. If the pulse duration is sufficiently long (microseconds), laser design is similar to that of a CW laser. However, many pulsed lasers are designed for pulse duration of a few nanoseconds. The light in each pulse cannot make many round-trips in the cavity. Resonant cavity designs used in CW lasers cannot control such a laser. The pulse dies before equilibrium conditions are reached. So, while two mirrors are still used in pulsed lasers for defining the direction of highest gain, they do not act as a resonant cavity. Instead, the usual method of controlling and tuning wavelength is a diffraction grating.

Some pulsed lasers, such as Nd:YAG (neodymium yttrium aluminum garnet) can be operated with a Q-switch, an intra-cavity device that acts as a fast optical gate. Light cannot pass it unless it is activated, usually by a high-voltage pulse. Initially, the switch is closed and energy is allowed to build up in the laser material. Then at the optimum time, the switch is opened and the stored energy is released as a very short pulse. This can shorten the normal pulse duration by several orders of magnitude. The peak power of a pulsed laser is proportional to pulse energy divided by the pulse duration. Q-switching, therefore increases the peak power by several orders of magnitude. The wavelength purity of Q-switched lasers is difficult to control because of the combination of high peak power and short pulse duration. To solve this problem, a low-power, well-controlled oscillator is often put in series with one or more amplifiers.

CW lasers can produce many longitudinal modes. If the cavity is pulsed or oscillated, it is possible to lock these modes together. The resultant interference causes the traveling light waves inside the cavity to collapse into a very short pulse. (We build a wave packet.) Every time this pulse reaches the output coupler, the laser emits a part of this pulse. The pulse repetition rate is determined by the time it takes for the pulse to make one trip around the cavity. The more modes interfere, the shorter is the pulse duration. The pulse duration is inversely proportional to the bandwidth of the laser gain material. The materials commonly used for tunable lasers produce the shortest mode-locked pulses. Popular materials include Ti:sapphire and similar materials. Turnkey commercial Ti:sapphire lasers now deliver pulses as short as 20 fs, with typical repetition rates around 100 MHz and peak powers approaching 1 MW.

Links:

	Interactive Laser Tutorial
	Ti:Sapphire Mode-Locked Lasers