Problem 1:

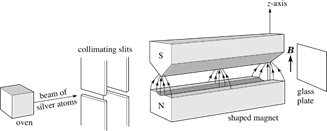
The sketch below illustrates the setup of the Stern-Gerlach experiment that historically confirmed the quantum nature of an atomic-scale system, specifically, the quantization of angular momentum.  In this setup, silver atoms are heated in an oven, creating a beam of atoms that goes through a collimator.  The beam is then subjected to an inhomogeneous magnetic field before colliding with a glass plate.

Answer the following questions about this experiment.
(a)  Why is an inhomogeneous magnetic field needed?
(b)  If the electron spin angular momentum is a classical quantity, what will be the distribution of silver atoms on the plate?
(c)  Based on your knowledge of electron spin, i.e., the quantum mechanical description, how do you predict the distribution of silver atoms on the plate?

Problem 2:

Here we have used the eigenspinors of the operator S_z as our basis.  'A' is a normalization constant.
(a)  If you measure S_z of this particle, what values do you get and what is the probability of each.
(b)  Find <S_z>.
(c)  If you measured S_x instead, what values do you get and what is the probability of each?

Problem 3:

A particle of mass m in an infinite square well of width L starts out in the left half of the well
and is at t = 0 equally likely to be found at any point in that region.  Assume that at t = 0 the particle's wave function is positive-real and constant for 0 < x < L/2, and zero everywhere else.

(a)  What is its initial normalized state ψ(x,0)?
(b)  Expand ψ(x,0) in terms of the eigenfunctions of the Hamiltonian for the infinite square well and determine the expansion coefficients.
(c)  What is the probability that a measurement of the energy at t = 0 would yield the value π²ħ²/(2mL²) in that state?
(d)  Assume that the energy in (c) is measured at time t_m. 
Determine the state of the particle for all t > t_m.

Problem 4:

A one-dimensional potential barrier or square well problem is defined by the Hamiltonian H = (P²/2m) + U(x), with U(x) = ζU₀Θ(ℓ/2 - |x|). Here Θ(z) = 0 for z < 0 and Θ(z) = 1 for z > 0,  ζ = +1 for potential barriers and ζ = -1 for square wells.
(a)  Calculate the transmittance T for E = 1 eV incident electrons facing a potential barrier of U₀ = 2 eV and ℓ = 1 Å.  What is the probability that a 1 eV protons will tunnel through the barrier?
(b)  Infer from (a) the general expression for the transmittance T, and draw T as a function of ℓ for E = 2.25 eV electrons.
(c)  Calculate eigenfunctions and eigenvalues of the square well in the limit U₀ --> ∞.

Problem 5:

Find <x> and ∆x for the nth stationary state of a free particle in one dimension restricted to the interval 0 < x < a.  Show that as n --> ∞ these become the classical values.