Problem 1:

The sketch below illustrates the setup of the Stern-Gerlach experiment that historically confirmed the quantum nature of an atomic-scale system, specifically, the quantization of angular momentum.  In this setup, silver atoms are heated in an oven, creating a beam of atoms that goes through a collimator.  The beam is then subjected to an inhomogeneous magnetic field before colliding with a glass plate.

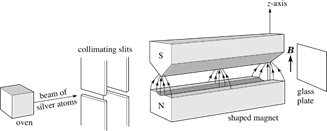
Answer the following questions about this experiment.
(a)  Why is an inhomogeneous magnetic field needed?
(b)  If the electron spin angular momentum is a classical quantity, what will be the distribution of silver atoms on the plate?
(c)  Based on your knowledge of electron spin, i.e., the quantum mechanical description, how do you predict the distribution of silver atoms on the plate?

Solution:

• Concepts:
  The Stern-Gerlach experiment
• Reasoning:
  In an inhomogeneous magnetic field, a magnetic dipole can experience a force and a torque.
• Details of the calculation:
  (a)  The electron has a magnetic moment μ proportional to its spin S, μ =  γS, with γ = -|q_e|/m_e a constant of proportionality.  In an external magnetic field, a magnetic moment can experience a force and a torque.  The force tries to pull an aligned dipole into regions where the magnitude of the magnetic field is larger and push an anti-aligned dipole into regions where magnitude the magnetic field is smaller.  If the magnetic field is pointing into the z-direction, the force on a magnetic dipole in that field is given by F_z = μ_zdB_z/dz. Here μ_z = μ cosθ is the z-component of the magnetic moment.  If B is constant, then dB_z/dz = 0, and there is no force. 
  
  (b)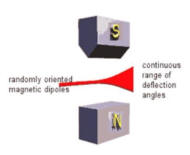  In an inhomogeneous field where dB_z/dz is not zero, the magnetic dipole experiences a force in the z-direction, unless θ = 90^o and μ_z= 0.   Since for a classical quantity μ can point into any direction the force F_z can have any value from -μdB_z/dz to +μdB_z/dz.  If we passed a beam of electrons, which are bound in a system that has no net charge and zero orbital angular momentum, and whose magnetic moments are randomly oriented and cannot change their orientation during the passage through an inhomogeneous magnetic field, different electrons would be deflected by different amounts.
  
  (c)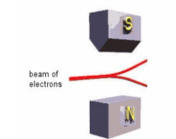  Assume the electrons are moving into the y-direction and pass through a magnet with an inhomogeneous field pointing into the positive z-direction.  In the figure the magnetic field points from the north pole to the south pole.  The magnitude of the field is larger near the south pole, because of the taper of the south pole piece.  The force experienced by a magnetic moment is F_z = μ_zdB_z/dz.  Here dB/dz is positive, since B becomes more positive as z increases.  For a particle with S_z = ħ/2, the z-component of the magnetic moment, μ_z, is negative, and therefore F_z is negative.  A particle with S_z = ħ/2 is deflected downward.   A particle with S_z = -ħ/2 is deflected upward.  The amount of deflection up or down is exactly the same.

Problem 2:

Here we have used the eigenspinors of the operator S_z as our basis.  'A' is a normalization constant.
(a)  If you measure S_z of this particle, what values do you get and what is the probability of each.
(b)  Find <S_z>.
(c)  If you measured S_x instead, what values do you get and what is the probability of each?

Solution:

• Concepts:
  The two dimensional state space of a spin ½ particle, the postulates of Quantum Mechanics
• Reasoning:
  S_z and S_x do not commute.  The eigenstates of S_x are linear combinations of eigenstates of S_z.
  |+>_x = 2^-½ (|+> + |->),  |->_x = 2^-½ (|+> - |->). 
• Details of the calculation:
  (a) The outcome of a measurement of S_z yields one of its eigenvalues, ħ/2 or -ħ/2.
  The probability of obtaining ħ/2 is 5A² and the probability of obtaining -ħ/2 is 4A².
  (5 + 4)A² = 1, A = 1/3,  P(ħ/2) = 5/9,  P(-ħ/2) = 4/9.
  (b)  <S_z> = (5/9)ħ/2 - (4/9)ħ/2 = ħ/18.
  (c)  The outcome of a measurement of S_x yields one of its eigenvalues, ħ/2 or -ħ/2.
  |+> = 2^-½(|+>_x + |->_x),  |-> = 2^-½(|+>_x - |->_x). 
  |χ> = 2^-½(1/3)[(1-2i)(|+>_x + |->_x) + 2(|+>_x - |->_x)] = 2^-½(1/3)[(3-2i)|+>_x + (-1-2i)|->_x].
  P(ħ/2)_x = 13/18,  P(-ħ/2)_x = 5/18.
  <S_x> = (13/18)ħ/2 - (5/18)ħ/2 = 4ħ/9.

Problem 3:

A particle of mass m in an infinite square well of width L starts out in the left half of the well
and is at t = 0 equally likely to be found at any point in that region.  Assume that at t = 0 the particle's wave function is positive-real and constant for 0 < x < L/2, and zero everywhere else.

(a)  What is its initial normalized state ψ(x,0)?
(b)  Expand ψ(x,0) in terms of the eigenfunctions of the Hamiltonian for the infinite square well and determine the expansion coefficients.
(c)  What is the probability that a measurement of the energy at t = 0 would yield the value π²ħ²/(2mL²) in that state?
(d)  Assume that the energy in (c) is measured at time t_m. 
Determine the state of the particle for all t > t_m.

Solution:

• Concepts:
  The infinite square well, postulates of QM
• Reasoning:
  The particle is in a superposition of eigenstates of the infinite well.
• Details of the calculation:
  (a)  ψ(x,0) = (2/L)^½. 0 < x < L/2.
  
  (b)  The normalized eigenfunctions and eigenvalues of H for the infinite square well are
  ψ_n(x) = (2/L)^½sin(nπx/L),  E_n = n²π²ħ²/(2mL²).  
  ψ(x,0) is a superposition of eigenstates of H.  ψ(x,0) = (2/L)^½∑a_n sin(nπx/L).
  a_k = ∫₀^Lψ_k*(x) ψ(x,0)dx = (2/L)^½∫₀^L ψ(x,0) sin(kπx/L) dx = (2/L)∫₀^L/2sin(kπx/L) dx.
  a_k = (L/(kπ))(2/L)∫₀ ^kπ/2sin(y) dy = (2/(kπ))(1 - cos(kπ/2)).
  a_k = 2/(kπ) if k = odd,  a_k = 4/(kπ) if k = 2, 6, 10, ... ,  a_k = 0 if k = 4, 8, 12, ... .
  
  (c)  The probability that a measurement of the energy at t = 0 will yield the value π²ħ²/(2mL²)
  is |a₁|² = 4/π².
  
  (d)  If the measurement at t = t_m yields an eigenvalue E₁, then the state of the particle for all t > t_m is
  Ψ₁(x, t) = (2/L)^½sin(πx/L)exp(-iE₁t/ ħ).

Problem 4:

A one-dimensional potential barrier or square well problem is defined by the Hamiltonian H = (P²/2m) + U(x), with U(x) = ζU₀Θ(ℓ/2 - |x|). Here Θ(z) = 0 for z < 0 and Θ(z) = 1 for z > 0,  ζ = +1 for potential barriers and ζ = -1 for square wells.
(a)  Calculate the transmittance T for E = 1 eV incident electrons facing a potential barrier of U₀ = 2 eV and ℓ = 1 Å.  What is the probability that a 1 eV protons will tunnel through the barrier?
(b)  Infer from (a) the general expression for the transmittance T, and draw T as a function of ℓ for E = 2.25 eV electrons.
(c)  Calculate eigenfunctions and eigenvalues of the square well in the limit U₀ --> ∞.

Solution:

• Concepts:
  This is a "square potential" problem.  We solve HΦ(x) = EΦ(x) in regions where U(x) is constant and apply boundary conditions.
• Reasoning:
  We are given a piecewise constant potential.  We have a potential that has a value of U₀ (barrier) or -U₀ (well) from x = -ℓ/2 to x = ℓ/2 and is zero otherwise.  We are asked to find the transmittance coefficient T.
• Details of the calculation:
  Let x' = x + ℓ/2.  Divide space into 3 regions.
  region 1: x' < 0, region 2: 0 < x' < ℓ, region 3: x' > ℓ.
  (a)  potential barrier, E < U₀.
  The most general solutions in regions 1, 2, and 3 are
  Φ₁(x') = A₁exp(ik₁x') + A₁'exp(-ik₁x'),
  Φ₂(x') = A₂exp(ρ₂x') + A₂'exp(-ρ₂x'),
  Φ₃(x') = A₃exp(ik₁x').
  Here k₁² = (2m/ħ²)E and ρ₂² = (2m/ħ²)(U₀ - E).
  The boundary conditions are that Φ(x') and (∂/∂x')Φ(x') are continuous at x' = 0 and x' = ℓ.
  x' = 0:  A₁ + A₁' = A₂ + A₂' , ik₁A₁ - ik₁A₁' = ρ₂A₂ - ρ₂A₂'.
  x' = ℓ:  A₂exp(ρ₂ℓ) + A₂'exp(-ρ₂ℓ) = A₃exp(ik₁ℓ),
  ρ₂A₂exp(ik₂ℓ) - ρ₂A₂'exp(-ρ₂ℓ) = ik_iA₃exp(ik₁ℓ).
  We need to solve these equations for A₃ in terms of A₁.
  (i)  Solve for A₂ and A₂' in terms of A₃.
  A₂ = ½exp((ik₁ - ρ₂)ℓ)(1 + ik₁/ρ₂)A₃ = C A₃.
  A₂' = ½exp((ik₁ + ρ₂)ℓ)(1 - ik₁/ρ₂)A₃ = C' A₃.
  (ii)  Now solve for A₁ in terms of A₃.
  2A₁ = A₂ + A₂' + (ρ₂/ik₁)(A₂ - A₂') = (C + C' + (ρ₂/ik₁)(C - C'))A₃.
  A₁ = ([(k₁² - ρ₂²))/(2ik₁ρ₂)]sinhρ₂ℓ + coshρ₂ℓ)exp(ik₁a)A₃.
  T = |A₃/A₁|² = 4k₁²ρ₂²/[(k₁² - ρ₂²)²sinh²ρ₂ℓ + 4k₁²ρ₂²cosh²ρ₂ℓ]
  = 4k₁²ρ₂²/[(k₁² + ρ₂²)²sinh²ρ₂ℓ + 4k₁²ρ₂²].
  [cosh²x - sinh²x = 1.]
  
  T = 4E(U₀ - E)/[U₀²sinh²[(2m(U₀ - E)/ħ²)^1/2ℓ] + 4E(U₀ - E)].
  
  If U₀ = 2 eV, E = 1 e V, and ℓ = 1 Å and the particle is an electron then
  T = 1/(1 + sinh²[(2m(U₀ - E)/ħ²)^1/2ℓ] = 1/(1 + sinh²(0.54)) = 0.76.
  If U₀ = 2 eV, E = 1 eV, and ℓ = 1 Å and the particle is an proton then
  T = 3.3*10^-20.
  
  (b)  If we have a barrier of height U₀ and 0 < E < U₀ the general expression for T is
  T = 4E(U₀ - E)/[U₀²sinh²[(2m(U₀ - E)/ħ²)^1/2ℓ] + 4E(U₀ - E)].
  If we have a barrier of height U₀ and E > U₀, or if we have a well (U₀ < 0) and E > 0 the general expression for T is
  T = 4E(E - U₀)/[U₀²sin²[(2m(E - U₀)/ħ²)^1/2ℓ] + 4E(E - U₀)].
  We can consider this second expression the general expression for T valid for all one-dimensional potential barrier or square well scattering problems, if we use the fact that sin²(ix) = -sinh²(x).
  For U₀ = 2 eV, E = 2.25 eV we have
  T = 2.25/[4sin²[(0.5 eV m/ħ²)^1/2ℓ)] + 2.25].
  For an electron (0.5 eV m/ħ²)^1/2 = 0.256/Å.
  
  
  
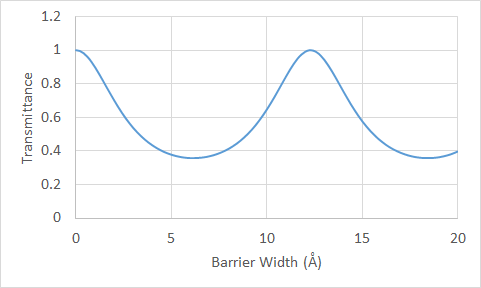
  (c)  We have a symmetric infinite square well.
  H = -(ħ²/2m)(∂²/∂x²) + U(x),  U(x) = 0 for  |x| < ℓ/2 and U(x) = ∞ for  |x| > ℓ.
  Change to the variable x'.
  H = -(ħ²/2m)(∂²/∂x_'²) + U(x'),  U(x') = 0 for 0 < x' < ℓ and U(x') = ∞ for x' < 0 and x' > ℓ.
  (∂²/∂x'²)Φ(x') + (2m/ħ²)EΦ(x') = 0 in the region 0 < x' < ℓ.
  Most general solution:  Φ(x') = Aexp(ikx') + Bexp(-ikx'), with E = ħ²k²/(2m) in the region 0 < x' < ℓ.
  Φ(x') = 0 for x' < 0 and x' > ℓ.
  Boundary conditions:  Φ(x') = 0 at x' = 0 and x' = ℓ.
  Therefore  k = nπ/ℓ and Φ(x') = (2/L)^½sin(nπx'/ℓ).
  Φ_n(x) = (2/L)^½cos(nπx/(2ℓ)), n = odd,  Φ_n(x) = (2/L)^½sin(nπx/(2ℓ)), n = even.
  E_n = n²π²ħ²/(2mℓ²).

Problem 5:

Find <x> and ∆x for the nth stationary state of a free particle in one dimension restricted to the interval 0 < x < a.  Show that as n --> ∞ these become the classical values.

Solution:

• Concepts:
  The eigenfunctions of the infinite square well, the postulates of quantum mechanics
• Reasoning:
  The expression for the mean value of an observable x in the normalized state |ψ> is
  <x> = <ψ|x|ψ>.  The root mean square deviation ∆x is given by 
  ∆x = (<x²> - <x>²)^½.  Here ψ_n(x) = (2/a)^½sin(nπx/a),  E_n = n²π²ħ²/(2ma²).
• Details of the calculation:
  <x>_n = (2/a)∫₀^a x sin²(nπx/a)dx = a/2.
  <x²>_n = (2/a)∫₀^a x² sin²(nπx/a)dx = (2/a)(a/(nπ))³∫₀^nπ x² sin²(x)dx
  = (2a²/(nπ)³)(nπ)³/6 - (2a²/(nπ)³)nπ/4 = a²/3 - ½a²/(nπ)².
  ∆x_n = (a²/12 - ½a²/(nπ)²)^½  --> a/12^½ as n --> ∞.
  
  Classically, the particle is moving back and forth in the well.  It is equally likely to be found at any position.
  The average position x_avg is a/2.
  For x²_avg we have x²_avg = a²/3.  (∫₀^a P(x) x²dx  with P(x) = 1/a.)
  If we define ∆x = (x²_avg - x_avg²)^½ we have ∆x = a/12^½.