Problem 1:
Show that the second Kepler law, the radius vector of a planet covers equal areas in equal times, follows directly from the angular momentum conservation law.
Problem 2:
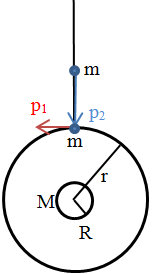A satellite is in a circular orbit of radius r around an airless spherical planet of
radius R. An asteroid of equal mass falls radially towards the
planet, starting at zero velocity from a very large distance. The satellite and
the asteroid collide inelastically and stick together,
moving in a new orbit that just misses the planet's surface. What was the radius
r of the satellite's original circular orbit in terms of R?
Problem 3:
Two particles with the same mass m orbit a massive planet of mass M >> m
and radius R. The orbit of one of the particles is circular, with radius
4R. The other particle's orbits in the same plane, but its orbit is
elliptical with semi-major axis 8R and rmin = 4R. The angular momenta of the two particles point in opposite directions. The particles
collide and stick together, forming a new object.
(a) What is the semi-major axis of the orbit of the new object in terms of R?
(b) Does the new object collide with the planet?
Problem 4:
A particle of mass m moves under the action of a central force whose
potential energy function is U(r) = kr3, k > 0.
(a)
For what energy and angular momentum will the orbit be a circle of radius a
about the origin? What is the period of this circular motion?
(b) If the particle is slightly disturbed from this circular motion, what
will be the period of small radial oscillations about r = a?
Problem 5:
Consider a particle of mass m moving in the xy plane. The potential energy
function is
U(x,y) = (k/2)(x2 + y2), with k a positive constant.
(a) Find the equations of motion.
(b) Are there circular orbits? If yes, do they all have the same period?
(c) Is the total energy conserved?