Problem 1:

A thrill-seeking student jumps off a bridge from the height H = 80.0 m above the water level.  The student is attached to the bridge by an elastic cord so that she reaches zero velocity just as she touches the water.  After some up-and-down bouncing, the student eventually comes to rest h = 20.0 m above the water level.  What is the maximum speed reached by the student during the fall?   Neglect air resistance and use 10.0 m/s² for the acceleration due to gravity.

Solution:

• Concepts:
  Gravitational and elastic potential energy
• Reasoning:
  Let us assume that dissipative forces are negligible during the first descent and that mechanical energy is conserved during the first descent.  Let us also assume that the bungee cord obeys Hooke's law when stretched, with a spring constant k.
  There are three distances we need to consider, the unstretched length of the cord, L, the equilibrium length of the cord with the student attached, d, and the maximum stretched length of the cord H.
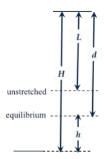• Details of the calculation:
  Hooke's law gives for the equilibrium position
  mg = k(d - L), mg/k = d - L.
  At the end of the first descent v = 0, all the gravitational potential energy has been converted into elastic potential energy.
  mgH = ½k(H - L)², mg/k = d - L = (H - L)²/(2H).
  L² = 2Hd - H² = (2*80*60 - 80²) m² = 3200 m².  L = 56.57 m.
  
  The jumper speed is a maximum when the acceleration is zero, which is at the equilibrium position.  Then, from energy conservation, mgd - ½k(d - L)² = ½mv².
  mgd - ½mg(d - L) = ½mv².  v² = 2gd - gd + gL = g(d + L) = 1165.7 m²/s².
  v_max = 34.14 m/s.

Problem 2:

A particle starts at the origin and moves in the xy-plane with velocity v_x = w + ay, v_y = u.
Let w = 2 m/s, u = 1 m/s, a = 1/s.  Find the trajectory y(x) of the particle.  What is its distance from the origin when x = 6 m?

Solution:

• Concepts:
  Kinematics
• Reasoning:
  We have two coupled first-order differential equations.
• Details of the calculation:
  dy/dt = u,  y = ut.
  dx/dt = w + aut.  x(t) = wt + ½aut²,  x(y) = wy/a + ½ay²/u.
  Trajectory y(x) = -w/a + [(w/a)² + 2xu/a]^½ =  -w/a + (w/a)[1 + 2aux/w²]^½.
  y(x = 6 m) = -2 m + 2*2 m = 2 m.
  Distance from origin d = (x² + y²)^½ = √40 m.

Problem 3:

Consider a r = 0.5 cm radius copper sphere (ρ = 8.96 g/cm³) moving through a fluid with viscosity η = 5 *10^-3 Pa-s in gravity-free space.  At t = 0 it is at r = 0 and has a velocity of v = 10 cm/s n.  (n is a unit vector pointing in some direction.)   Stokes law gives the drag force acting on the sphere in terms of the viscosity of the fluid.
F = -6πηrv.
Write down the equation of motion for the sphere and find r(t) and the distance of the sphere from the origin when it comes to rest.

Solution:

• Concepts:
  Newton's second law, drag
• Reasoning:
  The drag force is proportional to v,  mdv/dt = -μv.
• Details of the calculation:
  v = v₀ exp(-μt/m). 
  μt/m = 6π(5 *10^-3 Pa-s) t/(8.96 *10³ kg/m³ *4π(5*10^-3 m)²/3) = 0.1 t/s.
  v = (0.1 m/s)exp(-0.1 t/s) n.
  r(t) = (0.1 m/s)∫₀^texp(-0.1 t'/s)dt' n = (1 - exp(-0.1 t/s)) m n.
  The sphere comes to rest 1 m from the origin.
  
  or
  
  dv/dt = -(μ/m)v.  (This is a one-dimensional problem.
  dv/dt = (dv/dx)(dx/dt) = (dv/dx)v.  dv/dx = -(μ/m).
  ∫_v0⁰dv = -∫₀^R(μ/m)dx,  v₀ = (μ/m)R
  The distance the car will travel before comes to rest R = mv₀/μ.
  μ/m = 0.1/s.  R = (0.1 m/s)/(0.1/s) = 1 m.

Problem 4:

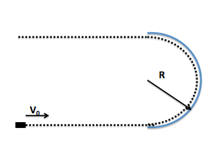An object is sliding on a frictionless surface with velocity V₀.  There is a track on the surface, which turns object backwards as shown by the dashed line in the figure.  If the radius of the track is R and the coefficient of kinetic friction between object and track is μ, how long will it take for the object to make a 180 degree turn?
The size of the object is negligible compared to the radius of the track.

Solution:

• Concepts:
  Circular motion, Newton's 2nd law, friction
• Reasoning:
  For the particle of mass m to move with speed v on the inside of the circular track, the track has to exert a force mv²/R towards the center of the circle.  This is a normal force.
  The frictional force with magnitude f = μN = μ mv²/R will slow the particle down.
• Details of the calculation:
  mdv/dt = -μmv²/R,  dv/v² = -(μ/R)dt.
  Integrate:  ∫_v0^v(t)dv'/v'² = -(μ/R)t.  1/v₀ - 1/v(t) = -(μ/R)t.   v(t) = v₀/(1 + (μv₀/R)t).
  The distance s(t) traveled in time t is s(t) = ∫₀^tv(t')dt' = v₀∫₀^tdt'/(1 + (μv₀/R)t').
  s(t) = (R/μ) ∫₁^{1 + (μv0/R)t} dt/t' = (R/μ)ln(1 + (μv₀/R)t).
  For s(t) = πR we have πμ = ln(1 + (μv₀/R)t),  exp(πμ) = 1 + (μv₀/R)t.
  t = R(exp(πμ) - 1)/(μv₀).
  Or
  dv/dt = (dv/ds) ds/dt = ½dv²/ds = -μv²/R.
  v²(s) = v₀²exp(-2μs/R),  v(s) = v₀exp(-μs/R),  v_{after 1/2 revolution} = v₀ exp(-πμ).
  v(s) = ds/dt,  dt = ds/v(s) = ds exp(μs/R)/v₀.
  Integrate from 0 to πR to find t(πR) = (exp(πμ) - 1)R/(μv₀).

Problem 5:

A satellite moves on a circular earth orbit that has a radius of 6700 km.  The radius of Earth is 6371 km.  A model airplane is flying on a 15 m guideline in a horizontal circle.  The guideline is parallel to the ground.   Find the speed of the plane such that the plane and the satellite have the same centripetal acceleration.

Solution:

• Concepts:
  Centripetal acceleration
• Reasoning:
  For the satellite the centripetal acceleration is GM_E/r_s² = gr_E²/r_s² = 8.86 m/s².
  For the plane the centripetal acceleration is v²/(15 m).
• Details of the calculation:
  We want v² = 15 m * 8.86 m/s²,  v = 11.5 m/s.