Problem :

A very long, solid insulating cylinder with radius R has a cylindrical hole with radius a bored along its entire length.  The axis of the hole is a distance b from the axis of the cylinder, where a < b < R.  The solid material of the cylinder has a uniform volume charge density ρ.  Find the magnitude and direction of the electric field inside the hole.

Problem 2:

A parallel plate capacitor has square plates of width w and plate separation d.  A square dielectric also of width w and thickness d, with permittivity ε and mass m, is inserted between the plates a distance x into the capacitor and held there.  The plates are connected to a battery with battery voltage V₀.

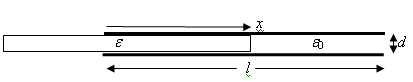
(a)  Derive a formula for the force exerted on the dielectric as a function of x.  Neglect edge effects. 
Assume that the battery stays connected and the dielectric is released from rest at x = w/2.  Describe its subsequent motion.
What is the range of the dielectric's motion, and within that range, what is the dielectric's speed as a function of position x? 
What is the maximum speed v_max of the dielectric?  Express your answers in terms of ε₀, ε, V₀, w, d and m.
(Neglect friction, ohmic heating, radiation.)

(b)  Now assume that the dielectric is released from rest at x = w/2 after the battery has been disconnected.
Derive a formula for the force exerted on the dielectric for w/2 < x < w.  Neglect edge effects. 
What is the maximum speed of the dielectric?  Express your answer in terms of ε₀, ε, V₀, w, d and m.

(c)  Find the ratio v_max(case a) to v_max(case b) in terms of ε₀ and ε.

Problem 3:

Suppose the input voltages V₁, V₂ and V₃ in the circuit shown can assume values of either 0 or 1 (0 means ground).  There are thus 8 possible combinations of input voltages.  List the V_out for each of these possibilities.

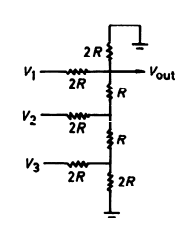
Problem 4:

A dipole p = pk = 4*10^-12 Cm is located at the origin. 
Find the force it exerts on a point charge q = 5*10^-6 C located on the z-axis at z = 10^-4 m.

Problem 5:

(a)  Imagine that the earth were of uniform density and that a tunnel was drilled along a diameter.  If an object were dropped into the tunnel, show that it would oscillate with a period equal to the period of a satellite orbiting the earth just at the surface.
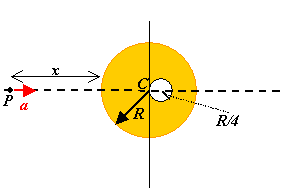
(b)  Find the gravitational acceleration at a point P, a distance x from the surface of a spherical object of radius R.  The object has density ρ.  Inside the object is a spherical cavity of radius R/4.  The center of this cavity is situated a distance R/4 beyond the center of the large sphere C, on the line from P to C.