Problem :

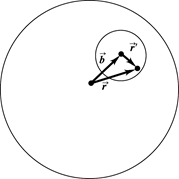
A very long, solid insulating cylinder with radius R has a cylindrical hole with radius a bored along its entire length.  The axis of the hole is a distance b from the axis of the cylinder, where a < b < R.  The solid material of the cylinder has a uniform volume charge density ρ.  Find the magnitude and direction of the electric field inside the hole.

Solution:

• Concepts:
  Gauss' law, the principle of superposition
• Reasoning:
  We can view the cylinder with the hole as a superposition of a cylinder with radius R having a uniform charge density ρ and another cylinder with radius a, located in the hole space, having a uniform charge density -ρ.  The field due to each of these cylindrical charge distributions can be found from Gauss' law.
  E = E₁(cylinder of radius R) + E₂(cylinder of radius a). 
• Details of the calculation:
  Let the axis of the large cylinder be the z-axis and let (r, θ, z) denote cylindrical coordinates.
  The electric field at a position r inside the hole due to the large cylinder is E₁  = ρr/(2ε₀).
  The electric field at a position r inside the hole due to the small cylinder is E₂  = -ρ(r - b)/(2ε₀).
  Therefore the electric field inside the hole is E = E₁ + E₂ = ρb/(2ε₀).
  The field inside the hole is constant and points into the direction of the vector b.

Problem 2:

A parallel plate capacitor has square plates of width w and plate separation d.  A square dielectric also of width w and thickness d, with permittivity ε and mass m, is inserted between the plates a distance x into the capacitor and held there.  The plates are connected to a battery with battery voltage V₀.

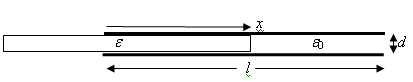
(a)  Derive a formula for the force exerted on the dielectric as a function of x.  Neglect edge effects. 
Assume that the battery stays connected and the dielectric is released from rest at x = w/2.  Describe its subsequent motion.
What is the range of the dielectric's motion, and within that range, what is the dielectric's speed as a function of position x? 
What is the maximum speed v_max of the dielectric?  Express your answers in terms of ε₀, ε, V₀, w, d and m.
(Neglect friction, ohmic heating, radiation.)

(b)  Now assume that the dielectric is released from rest at x = w/2 after the battery has been disconnected.
Derive a formula for the force exerted on the dielectric for w/2 < x < w.  Neglect edge effects. 
What is the maximum speed of the dielectric?  Express your answer in terms of ε₀, ε, V₀, w, d and m.

(c)  Find the ratio v_max(case a) to v_max(case b) in terms of ε₀ and ε.

Solution:

• Concepts:
  Capacitance, energy and work
• Reasoning:
  F_x = -F_mech = -dW_mech/dx.  We  find the work done by external agents as a dielectric is inserted into a capacitor and the capacitance changes.
• Details of the calculation:
  Capacitance, energy and work
  (a)  Capacitance as a function of x:  C = εwx/d  + ε₀w(w - x)/d,  0 < x < w.
  C = εw(2w - x)/d  + ε₀w(x - w)/d,  w < x < 2w.
  C = ε₀w²/d, x < 0 and x > 2w.
  Total energy store in the capacitor:  U = ½CV₀².
  As the position of the dielectric changes, the voltage across the capacitor plates stays the same.
  dW_mech + dW_bat = dU.  dW_bat = dQV₀ = dCV₀².  dU = ½dCV₀². 
  Therefore dW_mech = -½dCV₀². 
  F_x = -dW_mech/dx = ½V₀²dC/dx = ½V₀²(ε - ε₀)w/d  for 0 < x < w.
  The dielectric is pulled towards the right, into the capacitor for 0 < x < w.
  Similarly, the dielectric is pulled towards the left, into the capacitor for w < x < 2w.
  The force has the same constant magnitude.
  
  The dielectric is released from rest at x = w/2.
  The dielectric will oscillate about the equilibrium position x = w.  The motion is periodic, but not simple harmonic.  The restoring force has constant magnitude and points towards the right for w/2 < x < w and towards the left for w < x < 3w/2.
  
  Work - kinetic energy theorem:  ½mv² = F_x(x - w/2), 0 < x < w.
  ½mv_max² = F_xw/2.
  ½mv² = ½mv_max² - F_x(x - w), 0 < x < w.
  The maximum speed of the dielectric is v_max = [½V₀²(ε - ε₀)w²/(md)]^½.
  The speed as a function of position is v = [V₀²(ε - ε₀)w (x - w/2)/(md)]^½ for  w/2 < x < w,
  v = [v_max² - V₀²(ε - ε₀)w (x - w)/(md)]^½ = [V₀²(ε - ε₀)w (3w/2 - x)/(md)]^½  for  w < x < 3w/2.
  
  (b)  Now, as the position of the dielectric changes, the charge on the capacitor plates stays the same, Q₀ = C_w/2V₀ = ½V₀(ε + ε₀)w²/d.
  Total energy store in the capacitor:  U = ½Q₀²/C.
  dW_mech = dU = -½dC Q₀²/C².
  F_x = -dW_mech/dx = ½(Q₀²/C²) dC/dx = ½(Q₀²/C²) (ε - ε₀)w/d  for 0 < x < w.
  The dielectric is pulled towards the right, into the capacitor for 0 < x < w.
  Similarly, the dielectric is pulled towards the left, into the capacitor for w < x < 2w.
  But the force now does not have constant magnitude, since C depends on the position x.
  
  The dielectric is released from rest at x = w/2.
  ½mv_max² = ½Q₀²(1/C_W/2 - 1/C_W) = ½V₀²C_W/2(1 - C_W/2/C_W).
  C_W/2(1 - C_W/2 /C_W) = (½(ε + ε₀)w²/d)(1 - (½(ε + ε₀)w²/d)/(εw²/d))
  = ((ε + ε₀)w²/d)(ε - ε₀)/ε = ¼(w²/d)(ε² - ε₀²)/ε.
  v_max = [¼V₀² (ε² - ε₀²/ ε) w²/(md)]^½.
  
  (c)  v_max²(case a) /v_max²(case b) = 2ε(ε - ε₀)/(ε² - ε₀²) = 2ε/(ε + ε₀).

Problem 3:

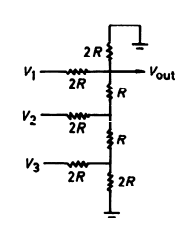
Suppose the input voltages V₁, V₂ and V₃ in the circuit shown can assume values of either 0 or 1 (0 means ground).  There are thus 8 possible combinations of input voltages.  List the V_out for each of these possibilities.

Solution:

• Concepts:
  Kirchhoff's rules
• Reasoning:
  We can find the currents I₁, I₂, I₃ and I₄ using Kirchhoff's rules.  The junction rule states that the sum of the currents entering a junction must equal the sum of the currents leaving that junction.  The loop rule states that the sum of the potential differences around any closed circuit loop must be zero.
• Details of the calculation:
  Redraw the circuit.
  
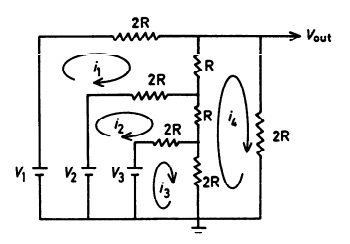
  V₃ - 2R(I₃ - I₂) - 2R(I₃ - I₄) = 0.
  (V₂ - V₃ ) - 2R(I₂ - I₁) - R(I₂ - I₄) - 2R(I₂ - I₃) = 0.
  (V₁-  V₂) - 2RI₁ - R(I₁ -  I₄)] - 2R(I₁ - I₂) = 0.
  2R(I₄ - I₃) + R(I₄ - I₂) + R(I₄ - I₁) + 2RI₄ = 0.
  V_out = 2I₄R.
  We want to solve this system of linear equations for I₄R.
  We obtain I₄R = (4V₁ + 2V₂ + V₃)/24.
  Therefore V_out = (4V₁ + 2V₂ + V₃)/12.
  V_out for the 8 possible combinations of input voltages is given in the table below.
  
  

  V1	V2	V3	Vout	---	V1	V2	V3	Vout
  0	0	0	0		1	0	0	1/3
  0	0	1	1/12		1	0	1	5/12
  0	1	0	1/6		1	1	0	½
  0	1	1	1/4		1	1	1	7/12

Problem 4:

A dipole p = pk = 4*10^-12 Cm is located at the origin. 
Find the force it exerts on a point charge q = 5*10^-6 C located on the z-axis at z = 10^-4 m.

Solution:

• Concepts:
  The dipole field,  E(r) = [1/(4πε₀)](1/r³)[3(p∙r)r/r² - p], F = qE.
• Reasoning:
  E = (p/(4πε₀r³))[2cosθ e_r + sinθ e_θ].
  On the z-axis E = (p/(2πε₀r³))cosθ e_r = (p/(2πε₀r³))k
• Details of the calculation:
  F = 5*10^-6*4*10^-12*1.8*10¹⁰/10^-12 N.
  F = 3.6*10⁵ N k.

Problem 5:

(a)  Imagine that the earth were of uniform density and that a tunnel was drilled along a diameter.  If an object were dropped into the tunnel, show that it would oscillate with a period equal to the period of a satellite orbiting the earth just at the surface.
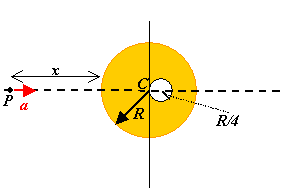
(b)  Find the gravitational acceleration at a point P, a distance x from the surface of a spherical object of radius R.  The object has density ρ.  Inside the object is a spherical cavity of radius R/4.  The center of this cavity is situated a distance R/4 beyond the center of the large sphere C, on the line from P to C.

Solution:

• Concepts:
  Newton's law of gravity, Newton's 2^nd law, the principle of superposition, uniform circular motion
• Reasoning:
  In part (a) the acceleration of the of the objects is due to the gravitational force.  The gravitational force on a object in the tunnel, a distance r from the center is in the -r direction and its magnitude is found using "Gauss' law". 
  [(∇∙F_g/m) = -4πGρ, for a spherical mass distribution. 
  4πr²(F_g/m) = 4πGM_inside, (F_g/m) = GM_inside/r². 
  Here F_g/m is the gravitational force per unit mass.]
  For part (b) we can use the principle of superposition. We compute the acceleration due to a sphere with radius R without a hole and subtract the acceleration due to a sphere with radius R/4 at the location of the hole.
• Details of the calculation:
  (a)  For the object of mass m in the tunnel a distance r from the center of the earth, the magnitude of the gravitational force is
  F = (4/3)Gmπρr, with ρ = 3M/(4πR³). 
  M and R are the mass and radius of the earth, respectively.  The force points towards the center.
  For an object moving in the tunnel we therefore have F = -kr, k = (4/3)Gmπρ.
  The force on the object obeys Hooke's law.  The object will oscillate with angular frequency ω = (k/m)^½ = (4Gπρ/3)^½.
  Its period is T = 2π/ω = (3π/(Gρ))^½ = 2π(R³/(GM))^½.
  For a satellite orbiting just above the surface we have
  GMm_s/R² = m_sv²/R = m_sRω²,  ω² = GM/R³, T = 2π/ω = 2π(R³/(GM))^½.
  The object in the tunnel and the satellite have the same period.
  
  (b)  a = Gρ(4/3)πR³/(x + R)² - Gρ(4/3)π(R/4)³/(x + 5R/4)².
  a = Gρ(4/3)πR³[(x + R)^-2 - (8x + 10R)^-2].
  The direction of a is towards the center of the sphere.