Problem 1:

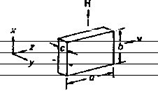An uncharged metal block has the form of a rectangular parallelepiped with sides a, b, c.  The block moves with velocity v in a magnetic field of intensity H as shown in the figure.  What is the electric field intensity in the block and what is the electric charge density in and on the block?

Problem 2:

Earth's magnetic field ends abruptly on the sunward side at approximately the point where the magnetic energy density has dropped to the same value as the kinetic energy density in the solar wind.  Near Earth, the solar wind contains about 5 protons and 5 electrons per cm³ and flows at 400 km/s.  Treating Earth's magnetic field as that of a dipole with dipole moment 8*10²² J/T, estimate the distance to the point above the equator where the field ends.

Problem 3:

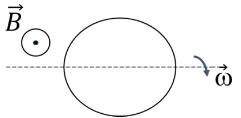A conducting loop of radius R is rotating around an axis in the plane of the loop, initially at an angular frequency ω₀.  A uniform static magnetic field B is applied perpendicular to the rotation axis.
(a)  What is the initial kinetic energy of the loop?
(b)  Calculate the rate of kinetic energy dissipation, assuming it all goes into Joule heating f the loop resistance.
(c)  In the limit that the change in energy per cycle is small, derive the differential equation that describes the time dependence of the angular velocity .  How long will it take for ω to fall to 1/e of its initial value?

Hint:  In the above limit you can replace the instantaneous rate of energy dissipation by its average value over a cycle.

Problem 4:

Consider a particle of charge q and mass m in the presence of a constant, uniform magnetic field B = B₀ k, and of a uniform electric field of amplitude E₀, rotating with
frequency ω in the (x,y) plane, either in the clockwise or in counterclockwise direction.
Let E = E₀cos(ωt) i ± E₀sin(ωt) j.
(a)  Write down the equation of motion for the particle and solve for the Cartesian velocity components v_i(t) in terms of  B₀, E₀, and ω if ω ≠ ω_c = qB₀/m (the cyclotron frequency).
Show that, if ω = ω_c, a resonance is observed for the appropriate sign of ω.
Hint:  Let ζ = v_x + iv_y, and solve for ζ(t).
(b)  Solve for the Cartesian velocity components v_i(t) at resonance.
(c)  Now assume the presence of a frictional force f = -mγv, where v is the velocity of the particle.  Find the general solution for ζ(t) for clockwise rotation of the electric field, and find the steady state solution (t >> 0)  when ω = ω_c.

Problem 5:

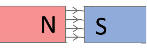The circular pole pieces of radius r = 4 mm of two permanent magnets are separated by a distance of 0.5 mm as shown in the figure. 
The magnetic field B is nearly uniform between the pole pieces, with
B = 0.8 T, and nearly zero everywhere else.  Calculate the force between the pole pieces.