Problem 1:

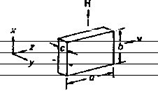An uncharged metal block has the form of a rectangular parallelepiped with sides a, b, c.  The block moves with velocity v in a magnetic field of intensity H as shown in the figure.  What is the electric field intensity in the block and what is the electric charge density in and on the block?

Solution:

• Concepts:
  Motional emf
• Reasoning:
  The magnetic force on the electrons inside the block is F_m = -q_ev × B = -q_evB j = -q_evμ₀H j. 
  Here q_e = 1.6*10^-19 C.
• Details of the calculation:
  Since in equilibrium the net force on the electrons inside the block is zero, we need -q_eE = q_evμ₀H j,  E = -vμ₀H j.
  The volume charge density inside the block is ρ = ε₀ ∇·E = 0,
  and the surface charge density is σ = +ε₀ vμ₀H on the front xy- plane of the block
  and σ = -ε₀ vμ₀H on the back xy- plane of the block (from Gauss' law).

Problem 2:

Earth's magnetic field ends abruptly on the sunward side at approximately the point where the magnetic energy density has dropped to the same value as the kinetic energy density in the solar wind.  Near Earth, the solar wind contains about 5 protons and 5 electrons per cm³ and flows at 400 km/s.  Treating Earth's magnetic field as that of a dipole with dipole moment 8*10²² J/T, estimate the distance to the point above the equator where the field ends.

Solution:

• Concepts:
  Magnetic energy density u_M = (2μ₀)^-1B², 
  Magnetic dipole field B(r) = (μ₀/(4π))(3(m·r)/r⁵ - m/r³) for a dipole at the origin.
• Reasoning:
  Dipole field above the equator:  B(r) = -μ₀m/(4πr³).
  Magnetic energy density above the equator:  u_M = μ₀m²/(32π²r⁶) = 2.55*10³⁷/r⁶ J/m³, with r in m.
  Here r is the distance measured from the center of Earth.
• Details of the calculation:
  The solar wind contains 5*10⁶ protons/m³ and 5*10⁶ protons/m³ moving with speed v = 4*10⁵ m/s.
  The kinetic energy density of these particles is u_K =  ½(5*10⁶)(m_p + m_e)v² = 6.69*10^-10 J/m³.
  We want 2.55*10³⁷/r⁶ = 6.69*10^-10,  r = 5.8*10⁷ m = 5.8*10⁴ km, about 10 earth radii.

Problem 3:

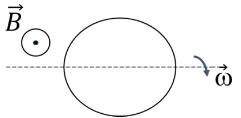A conducting loop of radius R is rotating around an axis in the plane of the loop, initially at an angular frequency ω₀.  A uniform static magnetic field B is applied perpendicular to the rotation axis.
(a)  What is the initial kinetic energy of the loop?
(b)  Calculate the rate of kinetic energy dissipation, assuming it all goes into Joule heating of the loop resistance.
(c)  In the limit that the change in energy per cycle is small, derive the differential equation that describes the time dependence of the angular velocity.  How long will it take for ω to fall to 1/e of its initial value?

Hint:  In the above limit you can replace the instantaneous rate of energy dissipation by its average value over a cycle.

Solution:

• Concepts:
  The induce emf ε = -∂F/∂t,  Ohms law, energy conservation
• Reasoning:
  A changing magnetic flux produces an emf in the ring.  The emf causes a current to flow which produces Joule heating.  Kinetic energy is converted to thermal energy.
• Details of the calculation:
  (a)  The initial kinetic energy of the loop is E = ½Iω₀², I = ½ma², E = ma²ω₀²/4.
  
  (b)  The magnetic flux through the ring is Bπa²cosθ.  The angle is changing, dθ/dt = ω.
  The induced emf due to this changing flux is -Bπa²sinθ ω.
  The current flowing in the ring is given by I = Bπa²sinθ ω/R (neglecting the self-inductance of the loop).
  The amount of kinetic energy converted into thermal energy per unit time is
  P = B²π²a⁴sin²θ ω²/R.
  
  (c)  Energy loss per cycle (assuming ω changes negligibly):
  ∫₀^T Pdt = ∫₀^2π (P/ω)dθ = π³ω B²a⁴/R = ΔE.
  Initial average energy loss per cycle due to Joule heating:  π³ω₀ B²a⁴/R.
  <P> = ΔE/T = ΔE/(2π/ω) = π²B²a⁴ω²/(2R) = -dE/dt.
  dE/dt = f(ω).  We want dE/dt = f(E), so we can solve the differential equation.
  E = ½Iω², I = ½ma², E = ma²ω²/4, ω² = 4E/ma²,  dE/dt = -E2B²π²a²/(Rm).
  E = E₀exp[-2B²π²a²t/(Rm)],  E is proportional to ω².
  ω = ω ₀exp[-B²π²a²t/(Rm)].  
  t_(1/e) = Rm/(B²π²a²) is the time it takes for the frequency of the rotation to decrease to 1/e of its initial value. 

Problem 4:

Consider a particle of charge q and mass m in the presence of a constant, uniform magnetic field B = B₀ k, and of a uniform electric field of amplitude E₀, rotating with
frequency ω in the (x,y) plane, either in the clockwise or in counterclockwise direction.
Let E = E₀cos(ωt) i ± E₀sin(ωt) j.
(a)  Write down the equation of motion for the particle and solve for the Cartesian velocity components v_i(t) in terms of  B₀, E₀, and ω if ω ≠ ω_c = qB₀/m (the cyclotron frequency).
Show that, if ω = ω_c, a resonance is observed for the appropriate sign of ω.
Hint:  Let ζ = v_x + iv_y, and solve for ζ(t).
(b)  Solve for the Cartesian velocity components v_i(t) at resonance.
(c)  Now assume the presence of a frictional force f = -mγv, where v is the velocity of the particle.  Find the general solution for ζ(t) for clockwise rotation of the electric field, and find the steady state solution (t >> 0)  when ω = ω_c.

Solution:

• Concepts:
  The Lorentz force, F = q(E + v × B) = mdv/dt
• Reasoning:
  dv_z/dt = 0, v_z = constant.  We have to solve coupled differential equations for v_x and v_y using the hint, ζ = v_x + iv_y.
• Details of the calculation:
  (a)  dv_x/dt = qv_yB₀/m + (qE₀/m)cos(ωt),
  dv_y/dt = -qv_xB₀/m ± (qE₀/m)sin(ωt),
  Let ζ  = v_x + iv_y,
  dζ/dt = -iqB₀ζ/m + (qE₀/m)exp(±iωt),  or
  dζ/dt + iω_cζ = (qE₀/m)exp(±iωt),.
  We find the solution to the inhomogeneous equation dζ/dt + iω_cζ = (qE₀/m)exp(±iωt)
  and add the solution of the homogeneous equation dζ/dt + iω_cζ = 0.
  
  inhomogeneous solution:
  Try ζ = ζ₀exp(±iωt),  ±iωζ₀ + iω_cζ₀ = (qE₀/m)  ζ₀ = -i(qE₀/m)/(ω_c ± ω).
  homogeneous solution:
  ζ = Aexp(-iω_ct), A = arbitrary complex constant = |A|exp(iφ).
  general solution:
  ζ = Aexp(-iω_ct) - i(qE₀/m)exp(±iωt)/(ω_c ± ω).
  v_x(t) = |A|cos(ω_ct + φ) ± (qE₀/m) sin(ωt)/(ω_c ± ω).
  v_y(t) = -|A|sin(ω_ct + φ) - (qE₀/m) cos(ωt)/(ω_c ± ω).
  
  We have a resonance (the expression is undefined) at ω = ω_c and the field rotates clockwise.
  i.e. E = E₀cos(ωt) i - E₀sin(ωt) j.
  
  (b)  At resonance dζ/dt + iω_cζ = (qE₀/m)exp(-iω_ct).
  Try a solution with a time-dependent amplitude, ζ = C(t)exp(-iω_ct).
  dC/dt - Ciω_c + iω_cC = qE₀/m.  C(t) = qE₀t/m + C₀.  C₀ = arbitrary complex constant.
  v = (qE₀t/m + C₀)exp(-iω_ct). 
  v_x(t) = (qE₀t/m) cos(ω_ct) + |C₀|cos(ω_ct + φ).
  v_x(t) = -(qE₀t/m) sin(ω_ct) - |C₀|sin(ω_ct + φ).
  
  (c) The equations of motion now are
  dv_x/dt = qv_yB₀/m - γv_x + (qE₀/m)cos(ωt),
  dv_y/dt = -qv_xB₀/m - γv_y - (qE₀/m)sin(ωt),
  dζ/dt + iω_cζ + γζ = (qE₀/m)exp(-iωt).
  
  inhomogeneous solution:
  Try ζ = ζ₀exp(-iωt),  ±iωζ₀ + iω_cζ₀ + γζ₀ = (qE₀/m)  ζ₀ = -i(qE₀/m)/(ω_c - ω -iγ).
  homogeneous solution:
  ζ = Aexp(-iω_ct -γt), A = arbitrary complex constant = |A|exp(iφ).
  general solution:
  ζ = Aexp(-iω_ct -γt) - i(qE₀/m)exp(-iωt)/(ω_c - ω -iγ).
  The first term decays exponentially.
  The steady state solution for ζ at resonance is ζ = (qE₀/(γm)) exp(-iω_ct).

Problem 5:

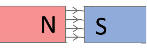The circular pole pieces of radius r = 4 mm of two permanent magnets are separated by a distance of 0.5 mm as shown in the figure. 
The magnetic field B is nearly uniform between the pole pieces, with
B = 0.8 T, and nearly zero everywhere else.  Calculate the force between the pole pieces.

Solution:

• Concepts:
  Energy stored in the magnetic field:
• Reasoning:
  F = -dU/dx.
• Details of the calculation:
• The magnetic field is confined to the region between the pole pieces.  The energy stored in the field is
  U = (2μ₀)^-1∫_{all space}B²dV in SI units. 
  Here U = (2μ₀)^-1B²πr²x, where x denotes the distance between the pole pieces. 
  F = -dU/dx = -(2μ₀)^-1B²πr² = 12.8 N. 
  The minus sign indicates that the force is attractive.