Problem 1:

(a)  When a gas isothermally expands against a fixed external pressure, would the expansion be reversible or irreversible?  Explain your answer.
(b)  Will the entropy of an ideal gas increase, decrease of remain fixed during the expansion?  Explain your answer.
(c)  Suppose the external pressure is zero, what would be the entropy change of the gas, its surroundings, and for the universe?  If appropriate, express your answer in terms of temperature and volume.

Problem 2:

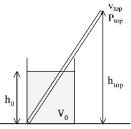A glass is filled to height h₀ with a volume of water V₀ with density ρ.  A straw with uniform cross sectional area A is used to drink the water by creating a pressure at the top of the straw (P_top) that is less than atmospheric pressure (P_Atm).  The top of the straw is a distance h_top above the bottom of the glass.

(a)  Determine the pressure (P_top) at the top of the straw as a function of the height (h) of the water in the glass so that the velocity of water coming out of the top of the straw remains constant at a value of v_top.
(b)  How much work is done by the person in order to drink all the water in the glass with the constant velocity v_top?
(c)  How much time does it take to drink all the water in the glass with the constant velocity v_top?

Ignore the viscosity of the water!

Problem 3:

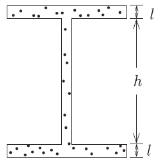N atoms, each of mass m, of an ideal monatomic gas occupy a volume consisting of two identical chambers connected by a narrow tube, as illustrated on the right.  There is a gravitational field with acceleration g directed downward in the figure.  The gas is in thermal equilibrium at temperature T.

The height h of the upper chamber above the lower chamber is much greater than the height l of either chamber.  The volume of the tube is negligible compared with that of the chambers. 
Assume that mgl << k_BT, but not that there is any particular relation between mgh and k_BT.  Treat the system classically and assume the atoms as having no internal degrees of freedom.
(a)  Calculate the number of atoms in the upper chamber in terms of N, m, g, h, and T.
(b)  Calculate the total energy (kinetic and potential) of the system in terms of the same parameters.
(c)  From the total energy obtain an expression for the specific heat of the system as a function of the temperature.

Problem 4:

(a)  One mole of ideal gas with constant heat capacity C_V is placed inside a cylinder.  Inside the cylinder there is a piston which can move without friction along the vertical axis.  Pressure P₁ is applied to the piston and the gas temperature is T₁. 
At some point, P₁ is abruptly changed to P₂ (e.g. by adding or removing a weight from the piston).  As a result, the gas volume changes adiabatically.  Find the temperature T₂ and the volume V₂ after the thermodynamic equilibrium has been reached in terms of C_V, P₁, T₁, and P₂.  Use the relation between heat capacities C_V and C_P to simplify the formulas.
Definition of C_V:  dU = C_VdT, C_P = C_V + R

(b)  After the thermodynamic equilibrium has been established in part (a), the pressure is abruptly reset to its original value P₁.  Compute final values of the temperature T_f and the volume V_f after the thermodynamic equilibrium has been reached again. 
Compute the difference in temperatures (T_f  - T₁) and show that it is quadratic in (P₂ - P₁).
Comment on the sign of the temperature difference.

Problem 5:

Two party balloons have a volume of 0.03 m³ each.  One is filled with air at 1.1 atmospheric pressure and its mass, including skin is 40.6 g.  The other is filled with helium at 1.1 atmospheric pressure and its mass, including skin, is 6.8 g.  The density of the air is 1.2 kg/m³.
A science museum has built an "elevator" which consists of a chamber with scales at the top and the bottom that can read the forces pushing against or pulling on the floor or the ceiling of the cart.  At the push of a button, the cart accelerates at a rate of 2 m/s² upward for 2 s, and then it decelerate at the same rate until it stops at its maximum height.
The air balloon is suspended from the ceiling with a string of negligible mass, and the helium balloon is fixed to the floor with the same type of string.
(a)  What is the maximum height reached by the bottom of the cart?
(b)  What is the tension in the strings when the cart is at rest, and in which direction do the strings pull on each balloon?
(c)  What is the tension in the strings while the car is accelerating upward, and in which direction do the strings pull on each balloon?

Let g = 10 m/s².  Assume the density of the air is constant in the chamber.