Problem 1:

(a)  A telescope with a primary mirror with a diameter of 20 m records 314 photons/second from a star.  Assume that no photons are lost in our atmosphere and that the telescope plus detector system is 1% efficient.  What is the flux of photons/s/m²?
(b)  What is the energy flux in W/m² if the effective wavelength is 500 nm?
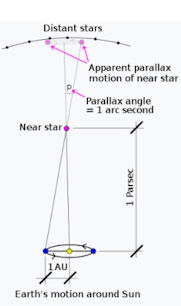
(c)  You observe this star for a year and see that it inscribes a small circle with diameter 1/3600 degrees relative to the fainter and much more distant stars.  The circular motion repeats with exactly one year period.  What is the distance to this star?  The Earth is 150 million km from the Sun.
(d)  How much energy does this star emit in all directions per second into the waveband that was detected (in part a)?

Solution:

• Concepts:
  Flux, triangulation
• Reasoning:
  The circular motion is due to parallax. The baseline is provided by Earth's annual trip around the Sun.
• Details of the calculation:
  (a)  Photon flux = 100*314/(π*100) photons/s/m² = 100 photons/s/m².
  
  (b)  Energy flux = (100 photons/s/m²)*hc/λ = 248 eV/s/m² = 4*10^-17 W/m².
  
  (c)  1 arcsec = (1/3600) deg = (1/3600)(2π/360) rad.
  parallax angle θ = ½ arcsec = 2.42*10^-6.
  tanθ ≈ θ = 1 AU/d,  d = 412526 AU = 6.2*10¹⁶ m.
  
  (d) Total E/s = (4*10^-17 W/m²)(4πd²) = 1.9*10¹⁸ W.

Problem 2:

A research reactor produces fluxes of neutrons to undertake measurements of the structure of materials.  The neutrons are emitted from an aperture in the reactor wall.  The beam contains a wide mixture of neutrons with different energies.  Bragg reflections by a crystal can be used to separate the a monoenergetic beam from the mixture in a similar way to how a diffraction grating separates colors of light into a monochromatic beam.
(a)  Suppose that Beryllium crystals with a Bragg plane spacing d = 1.80 Angstroms (1.8*10^-10 m) are used to separate neutrons with an energy of 0.010 eV.  What is the de Broglie wavelength λ in Angstrom of a neutron with an energy of 0.010 eV?  Note that the neutrons are nonrelativistic.

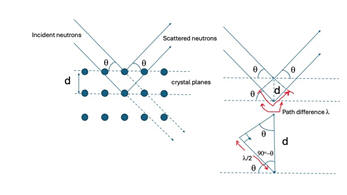
(b)  Calculate the angle 2θ between the incident beam and scattered beam directions for this wavelength of neutrons based on the Bragg condition that the path difference for scattered neutrons is λ between successive crystal planes.

Solution:

• Concepts:
  The deBroglie wavelength, Bragg diffraction
• Reasoning:
  The Bragg condition for constructive interference is nλ = 2d sinθ, where θ is the angle between the incident wave vector and the scattering planes.
• Details of the calculation:
  (a)  E = p²/2m = (h/λ)²/2m.
  λ² = h²/(2mE) = (hc)²/(2mc²E)
  = (1240 eV nm)²/(2*9.396*10⁸ eV*0.01 eV)
  = 8.18*10^-2 nm².
  λ = 2.86 Angstrom.
  
  (b)  λ = 2d sinθ for for first-order constructive interference.  θ is the angle between the incident wave vector and the scattering planes.
  θ = sin^-1(λ/(2d)) = sin^-1(2.86/3.6) = 0.9183 rad.
  2θ = 105^o.

Problem 3:

For a classroom demonstration, you pass the beam from a HeNe laser (λ = 633 nm) through two identical, closely-spaced slits and observe the interference pattern on a wall 5 m away and perpendicular to the plane containing the slits.  In the darkened classroom, you can clearly observe up to 10 interference maxima on both sides of the central maximum, but the 4^th and 8^th maxima are missing on either side.  The spacing between adjacent maxima on the wall is 1.1 cm.
(a)  What is the spacing between the slits?
(b)  What is the slit width?

Solution:

• Concepts:
  Diffraction and interference
• Reasoning:
  Double slit interference can be observed at angles where single slit diffraction provides non-zero intensity.
• Details of the calculation:
  (a)  Double slit interference: dsinθ = mλ.  Small angle approximation: 
  sinθ = tanθ = θ = m*1.1*10^-2/5.
  d = (6.33*10^-7 m)/(2.2*10^-3) = 0.288 mm is the slit separation.
  
  (b)  The interference maximum at 4*1.1 cm is missing since it falls on the first minimum in the diffraction pattern.  For this first minimum wsinθ = λ,  sinθ = 4.4*10^-2/5.  Therefore w = 71.9 μm is the slit width.

Problem 4:

In one dimension, a particle of mass m has potential energy U(x) = V cos(αx) - Fx, with V a positive constant.  Determine the frequency of small oscillations.  What relationship between V, α, and F makes oscillatory motion possible?

Solution:

• Concepts:
  Small oscillations
• Reasoning:
  We are looking for minima in the potential energy function.
• Details of the calculation:
  For an extremum we need dU(x)/dx = 0 --> -Vαsin(αx) - F = 0,  sin(αx) = -F/(Vα).
  We need -1 < F/(Vα) < 1 for an extremum to exist.  We need F² < (Vα)².
  d²U/dx²|_extremum = -Vα²cos(αx)|_extremum = ±Vα²(1 - F²/(Vα)²)^½.
  We need d²U/dx²|_extremum > 0, so cos(αx₀) = -(1 - F²/(Vα)²)^½, where x₀ + n2π are the positions of the minima of U(x).
  F(x) = -dU(x)/dx.
  dU(x)/dx|_x0 = 0.  dU(x)/dx|_x0+q = dU(x)/dx|_x0 + d²U/dx²|_x0q = Vα²(1 - F²/(Vα)²)^½q to first order in q.
  Near any minimum F(x₀ + q) = -Vα²(1 - F²/(Vα)²)^½q to first order in q.
  We have a Hooke's law restoring force,  F(x₀ + q) = -kq.
  The particle will oscillate with frequency f = ω/(2π), where ω² = (Vα²/m)(1 - F²/(Vα)²)^½.

Problem 5:

A 3-kg sphere dropped through air has a terminal speed of 25 m/s.  Assume that the drag force is proportional to the velocity.  The sphere is attached to a spring of force constant 400 N/m and starts to oscillate with an initial amplitude of 20 cm.
(a)  When will the amplitude be 10 cm?
(b)  How much energy will have been lost when the amplitude is 10 cm?

Solution:

• Concepts:
  The damped harmonic oscillator
• Reasoning:
  The equation of motion is F = -kx - cdx/dt.  Given the terminal speed we can solve mg = cv_terminal for c.
• Details of the calculation:
  (a)  When considering masses oscillating on vertical springs in a uniform gravitational field, the gravitational force does not appear in the equations of motions if we write these equations in terms of the displacements from the equilibrium positions in the gravitational field.
  The equation of motion for the mass is d²x/dt² = -ω₀²x - (c/m)dx/dt.
  Here ω₀² = k/m = 133.33 s^-2 and c = mg/v_terminal = 1.1772 kg/s.
  Let x = Aexp(iΩt).  (The real part is implied.)  Then
  -Ω² + 2k/m + iΩc/m = 0,  Ω = ic/(2m) ± (ω₀² - c²/(2m)²)^½.
  The position as a function of time is given by
  x = A exp(-ct/(2m))cos(ωt + φ), with ω = (ω₀² - c²/(2m)²)^½.
  ω₀ = 11.547/s  >> c/(2m) = 0.196/s, so the period of the oscillation is T ≈ 2π/ω₀ = 0.542 s is much smaller than the inverse of the damping coefficient 1/γ = 2m/c = 5.1 s.  We have under-damped motion.
  
  The initial amplitude is A = 20 cm at t = 0 so φ = 0.
  Solve exp(-ct/(2m)) = ½ for the time t when the amplitude is 10 cm.
  exp(-ct/(2m)) = ½,  ct/(2m) = ln(2),  t = 2mln(2)/c = 3.53 s.

  (b) The total energy of the mass is E = ½kA².  The initial energy is 8 J.
  When the amplitude has been reduced to ½ the initial amplitude, 3/4 of the initial energy or 6 J has been lost.