Problem 1:

Coordinate system S' is rotating with a time-dependent angular velocity ω relative to a fixed coordinate system S.  The relationship between the acceleration a of a point in S and the acceleration a' of a point in S' is given by

a = a' + dω/dt × r + 2ω × v' + ω × (ω × r).

(a)  Indicate what fictitious forces each of the last three terms correspond to.
(b)  A particle is projected vertically upward with velocity v₀ on Earth's surface at a northern latitude λ. Where will the particle land with respect to its launch location?
For this problem, assume the launch height is small compared to Earth's radius R.
State any other assumptions or approximations you make explicitly.

Problem 2:

An object of rotational inertia I is initially at rest.  A torque is then applied causing the object to begin rotating.  The torque is applied for only one-quarter of a revolution, during which time its magnitude is given by τ = A cosθ, where A is a constant and θ is the angle through which the object has rotated.  What is the final angular speed of the object?

Problem 3:

Why are there tides?  Describe the mechanism in a few sentences, including what circumstances lead to maximally high and low tides.

Problem 4:

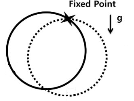A hoop of mass m and radius R = 1.25 m can oscillate in the vertical plane about a fixed point.
(a)  What is the equation of motion for this physical pendulum for small angular displacements θ from equilibrium?
(b)  What is the angular frequency ω for small oscillations?
(c)  If the pendulum is released from rest at time t = 0 at θ = 3^o, solve for θ(t).
Let g = 10 m/s².

Problem 5:

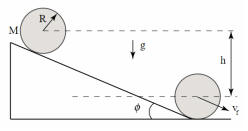
A uniform disk with mass M and radius R starts from rest and moves down an inclined at an angle from the horizontal φ.  The center of the disk has dropped a vertical distance h when it reaches the bottom of the incline.  The incline and the horizontal floor are perfectly rough and the disk rolls without slipping on the incline and on the floor.  What is the velocity of the CM of the disk on the horizontal floor?