Problem 1:

Coordinate system S' is rotating with a time-dependent angular velocity ω relative to a fixed coordinate system S.  The relationship between the acceleration a of a point in S and the acceleration a' of a point in S' is given by

a = a' + dω/dt × r + 2ω × v' + ω × (ω × r).

(a)  Indicate what fictitious forces each of the last three terms correspond to.
(b)  A particle is projected vertically upward with velocity v₀ on Earth's surface at a northern latitude λ. Where will the particle land with respect to its launch location?
For this problem, assume the launch height is small compared to Earth's radius R.
State any other assumptions or approximations you make explicitly.

Solution:

• Concepts:
  Motion in a non-inertial frame
• Reasoning:
  For part (b), choose your coordinate system as shown in the figure.
  
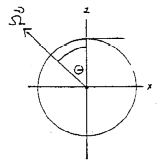
  Assume an observer at mid latitudes λ = 90^o - θ.
• Details of the calculation:
  (a)  a' = a - dω/dt × r - 2ω × v' - ω × (ω × r).
  -mdω/dt = fictitious force due to non-uniform rotation of frame
  -2mω × v' = Coriolis force
  -ω × (ω × r) = centrifugal force
  
  (b)  Choose your coordinate system as shown in the figure.
  Assume an observer in the rotating frame at mid latitudes λ = 90^o - θ.
  The equations of motion in a rotating coordinate system contain fictitious forces.
  mdv'/dt = F_inertial  - 2mω × v' - mω × (ω × r).
  mdv'/dt = mg_eff - 2mω × v'.
  g_eff = g + O(ω²).  Neglect terms of order ω² and air resistance and consider only small vertical heights.
  ω =  ω_xi + ω_zk,  ω_y = 0.
  We know that the deflection will be very small.  The particle will be above the ground for a small time interval, and v_x and v_y will be very small.
  Therefore
  (ω × v')_x = ω_yv'_z - ω_zv'_y ≈ 0,
  (ω × v')_y = ω_zv'_x - ω_xv'_z ≈ - ω_xv'_z ,
  (ω × v')_z = ω_xv'_y - ω_yv'_x ≈ 0.
  
  dv'_z/dt = -g_eff ,  dv'_y/dt = 2ω_xv'_z.
  v'_z(t) = v₀ - g_efft, v'_y(t) = 2ω_x∫₀^t(v₀ - g_efft')dt'
  = -2ω sinθ v₀t + ω sinθ g_efft².
  
  y(t) = ∫₀^tv'_y(t')dt' = -ω sinθ v₀t² + ω sinθ g_efft³/3.
  t = 2v₀/g_eff.
  
  For the deflection we therefore have
  y(v₀) = -4ω sinθ v₀³/g_eff² + 8ω sinθ v₀³/(3g_eff²) = -(4/3)ω sinθ v₀³/g_eff²,
  or
  y(v₀) = -(4/3)ω cosλ v₀³/g_eff².
  The object is deflected westward.

Problem 2:

An object of rotational inertia I is initially at rest.  A torque is then applied causing the object to begin rotating.  The torque is applied for only one-quarter of a revolution, during which time its magnitude is given by τ = A cosθ, where A is a constant and θ is the angle through which the object has rotated.  What is the final angular speed of the object?

Solution:

• Concepts:
  Rotational motion, work-kinetic energy theorem, or solving a differential equation
• Reasoning:
  τ = Id²θ/dt²,  d²θ/dt² = (A/I)cosθ.
• Details of the calculation:
  Work-kinetic energy theorem:
  ½Iω_f² = ∫₀^π/2 τ dθ = A∫₀^π/2 cosθ dθ = A
  ω_f = (2A/I)^½.
  
  Or, solving a differential equation;
  (dθ/dt) d²θ/dt² = (dθ/dt) (A/I)cosθ.
  Let dθ/dt = x.
  x dx/dt = ½dx²/dt= (dθ/dt) (A/I)cosθ.
  Integrate:
  ½∫(dx²/dt) dt = ½∫dx² = (A/I)∫ (dθ/dt) cosθ dt = (A/I)∫ dθ cosθ.
  ½x² = ½(dθ/dt) ² = (A/I) sinθ + constant.
  dθ/dt = ((2A/I) sinθ + C)^½, with C determined by the initial conditions.
  ω₀² = 0 --> C = 0.
  After ¼ revolution, θ = π/2, ω_f = (2A/I)^½.

Problem 3:

Why are there tides?  Describe the mechanism in a few sentences, including what circumstances lead to maximally high and low tides.

Solution:

• Concepts:
  Tides in the Sun, Earth, Moon system
• Reasoning:
  Let us look at the tides on Earth from a reference frame fixed on Earth, with its origin at the CM of Earth and its positive z-axis passing through the North Pole.  This reference frame is an accelerating frame.  Earth rotates about the z-axis, and its CM accelerates as Earth orbits the center of mass of the Earth-Moon system, that system orbits the sun, ... .  In an accelerating frame fictitious force appear. 
  The centrifugal force due to the spinning of Earth about the z-axis causes the shape of Earth to be an oblate spheroid.
  Earth and Moon attract each other and both orbit the CM of the Earth-Moon system. The Moon is about one Earth diameter closer to the near side of the Earth than the far side.  This results in a stronger gravitational force on the near side compared to the far side.
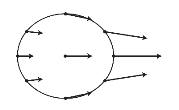
  The diagram below illustrates the magnitude of the gravitational force the Moon exerts on Earth as seen from an inertial frame.  The Moon is to the right of Earth.
  
   
  Now let us look at the forces as seen from the accelerating frame with its z-axis passing through the CM of Earth.
  Neglecting this spin for a moment and only considering the acceleration of the CM of Earth, we can add -dm a_CM to the gravitational force acting on each mass element dm, and leave all other forces (normal, elastic, ... ) unchanged.
  The diagram below illustrates the magnitude of the sum of the gravitational force and the fictitious force as seen from the accelerating frame.
  
  
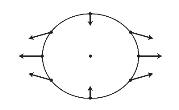
  If Earth, Moon, and Sun are aligned, these fictitious forces are stronger, resulting in high tides.  When Moon and Sun are at right angles seen from Earth the tidal forces are smallest, resulting in neap tides.  The main body of the Earth is made of rock, which is stiff and resists large deformation by tides.
  The Earth rotates faster than the Moon orbits the Earth.  Friction between the ocean and the seabed as the Earth turns out from underneath the ocean tidal bulges drags the ocean bulges in the eastward direction of the Earth's rotation resulting in the ocean tides leading the Moon by several degrees.

Problem 4:

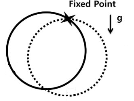A hoop of mass m and radius R = 1.25 m can oscillate in the vertical plane about a fixed point.
(a)  What is the equation of motion for this physical pendulum for small angular displacements θ from equilibrium?
(b)  What is the angular frequency ω for small oscillations?
(c)  If the pendulum is released from rest at time t = 0 at θ = 3^o, solve for θ(t).
Let g = 10 m/s².

Solution:

• Concepts:
  The physical pendulum, the parallel axis theorem, small oscillations
• Reasoning:
  We have a physical pendulum subject to a constant downward force F = mg.
  We have pure rotation about a suspension point.  τ = Id²θ/dt² =  -mg l'sinθ.
  Here l' is the distance from the suspension point to the CM and I is the moment of inertia about the suspension point.
• Details of the calculation:
  (a)  Equation of motion:  d²θ/dt² = -(mgl'/I)θ.
  
  (b)  ω² = mgl'/I.
  Using the parallel axis theorem we have for the moment of inertia of the hoop about the suspension point I = mR² + mR² = 2mR². 
  The distance l' = R.  Therefore d²θ/dt² = -(g/2R)θ = (4/s²)θ.
  ω² = 4/s², ω = 2/s.
  (c)  The most general solution of the equation of motion is
  θ(t) = A cos(ωt) + Bsin(ωt),  dθ(t)/dt = −Aωsin(ωt) + Bωcos(ωt).
  
  (c)  The initial conditions are θ(0) = 3^o = A  dθ/dt|₀ = 0 = Bω --> B = 0. 
  θ(t) = 3^ocos(2t).

Problem 5:

A uniform disk with mass M and radius R starts from rest and moves down an inclined at an angle from the horizontal φ.  The center of the disk has dropped a vertical distance h when it reaches the bottom of the incline.  The incline and the horizontal floor are perfectly rough and the disk rolls without slipping on the incline and on the floor.  What is the velocity of the CM of the disk on the horizontal floor?

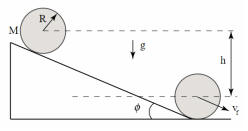
Solution:

• Concepts:
  ΔP = FΔt,  ΔL = τΔt,  relationship between linear and angular momentum for rolling motion
• Reasoning:
  The disk makes an inelastic collision with the floor.  Its momentum and angular momentum change.  The changes in momentum and angular momentum are not independent of each other but are connected by the fact that the same force provides the linear and angular impulse.
• Details of the calculation:
  Energy conservation yields the speed of the disk when it contacts the floor.
  Mgh = ½Iω² + ½Mv² = (3/4)Mv²/R. v = (4gh/3)^½.
  Choose your coordinate system such that the x-axis points to the right and the y-axis points up.
  
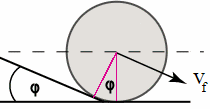
  The disk has momentum p = mv cosφ i - mv sinφ j and angular momentum L = -½MR²v/R k = -½MRv k.
  As the disk contacts the ground an impulse reduces the y-component of its momentum to zero and changes the x-component of its momentum to
  p' = Mv' = Mv cosφ + F_xΔt. 
  The force F_x also results in a torque which changes the magnitude of the disks angular momentum to 
  L' = Iω^' = ½MRv - F_xRΔt = ½MRv - RMv'+ RMv cosφ.
  For rolling we need ω' = v'/R.
  ½MRv' = ½MRv - RMv'+ RMv cosφ.
  ½v' = ½v - v'+ v cosφ.
  (3/2)v' = (½ + cosφ)v.
  v' = (2/3)(½ + cosφ)v.
  v' =  (16gh/27)^½(½ + cosφ).