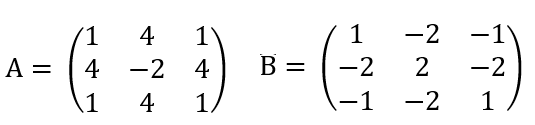
(a) Argue why both matrices are diagonalizable. No calculation allowed.
(b) Find the eigenvalues and eigenvectors of A.
(c) Find [A,B].
(d) The similarity transformation B' = Q-1BQ diagonalizes matrix B. Find Q and Q-1.
Consider the matrices A and B.
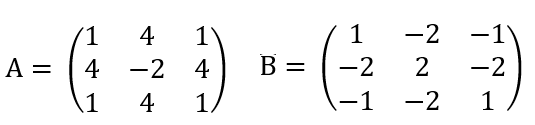
(a) Argue why both matrices are diagonalizable. No calculation allowed.
(b) Find the eigenvalues and eigenvectors of A.
(c) Find [A,B].
(d) The similarity transformation B' = Q-1BQ
diagonalizes matrix B. Find Q and Q-1.
You prepare an ensemble of identical experiments at t = 0. You measure the values of a physical observable A at time t = t1 > 0 in all of the experiments of the ensemble. At time t2 > t1 you measure observable A again in all of the experiments, i.e. you follow the measurement at t = t1 in each experiment with a subsequent measurement of A in each experiment at t = t2.
True or False: (Explain your choice in one or two sentences.)
(a) The values you obtain for A across all of the experiments at time t = t1
are the same given you have made all of the measurements at the same time t = t1.
(b) The value you obtain for A in each experiment at t = t2 may be
different relative to the value obtained for A in the same experiment at t = t1.
(c) The expectation value of A is the same at both t = t1 and t = t2.
(d) The values you obtain for A in any of the experiments at either t = t1
or t = t2 must be one of the eigenvalues of its associated Hermitian
operator.
At t = t3 > t2 you measure a second physical observable
B across all of the experiments, i.e. you follow the measurement of A at t = t2
in each experiment with a measurement of B at t = t3 in each
experiment. The commutator of the two Hermitian operators associated with A and
B satisfies [A, B] ≠ 0.
True or False:
(e) The values you obtained for A at t = t2 in any of these
experiments will remain the same at t = t3 given you are measuring a
different physical quantity B, not A.
Now you reset all of the experiments to what they were at t = 0, i.e., you
start all over again with the same initial set of identical experiments. At
time t = t4, you measure A again in all of your experiments.
Moreover, the commutator of the operator associated with A and the Hamiltonian
operator satisfies
[H, A] =0 .
True or False:
(f) The value you obtain for A in each experiment at t = t4 is the
same as the value you obtained for A in the corresponding experiment at t = t1.
(g) The expectation values of A at t = t and t = t4 are the same.
Consider a two-state system governed by the Hamiltonian H with energy eigenstates |E1> and |E2>,
where H|E1> = E1|E1> and H|E2> = E2
|E2>. Consider also two other states,
|x> = (|E1> + |E2>)/√2, and |y> = (|E1>
- |E2>)/√2.
At time t = 0 the system is in state |x>. At what
subsequent times is the probability of finding the system in state |y> the
largest, and what is that probability?
Consider the one-dimensional time-independent Schroedinger equation for some
arbitrary potential U(x). Prove that if a solution ψ(x) has the property that
ψ(x) → 0 as x → ±∞, then the solution must be non-degenerate and therefore real,
apart from a possible overall phase factor.
Hint: Show that the contrary assumption leads to a contradiction.
A particle in one dimension is in a stationary state.
(a) Show that for any time-independent operator Q, the
expectation or mean value <Q> is independent of time.
(b) Show that the mean value of the particles momentum, <p>, is zero.