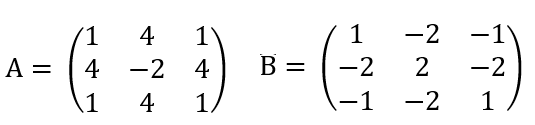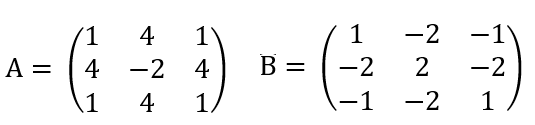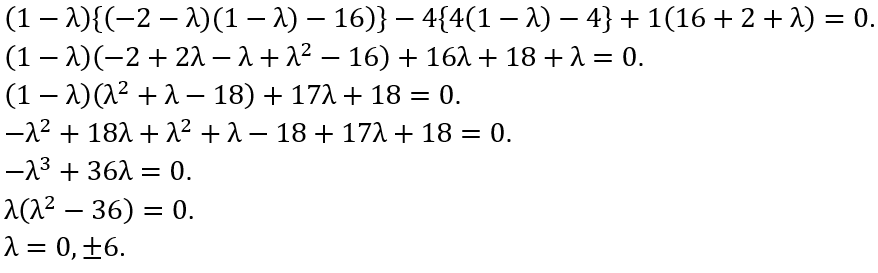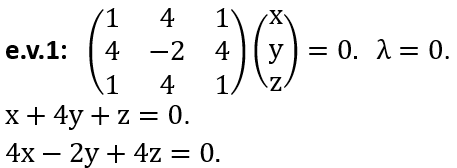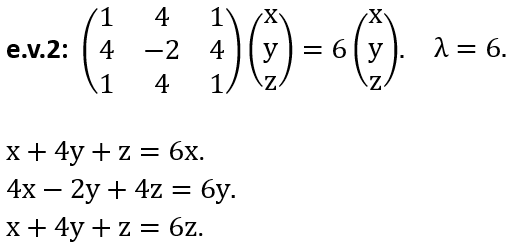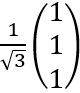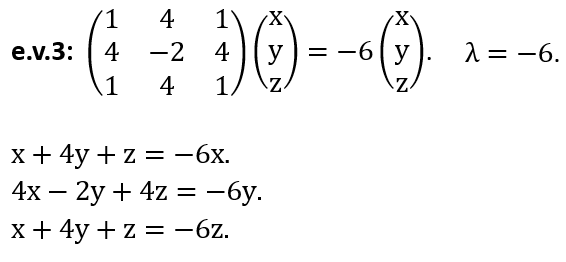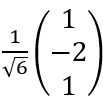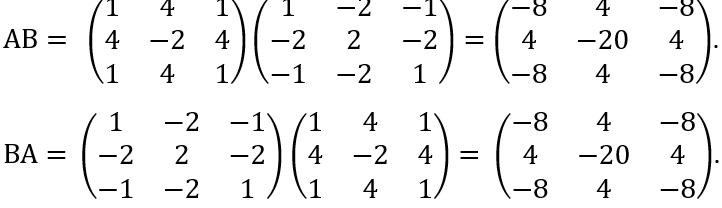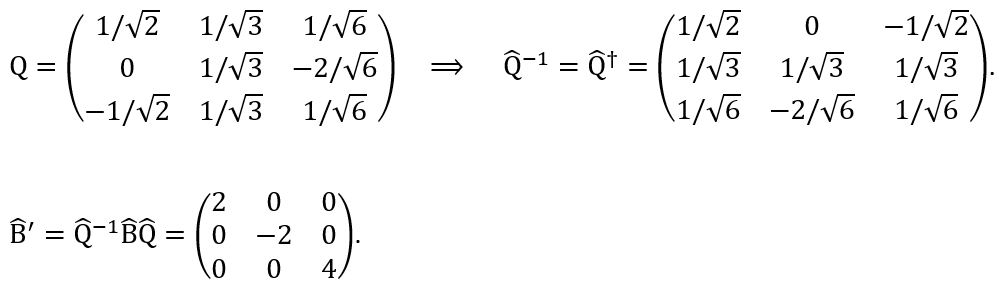Problem 1:

Consider the matrices A and B.

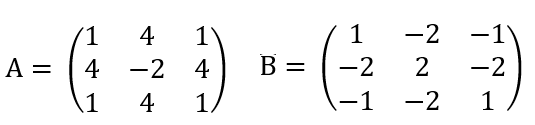
(a)  Argue why both matrices are diagonalizable.  No calculation allowed.
(b)  Find the eigenvalues and eigenvectors of A.
(c)  Find [A,B].
(d)  The similarity transformation B' = Q^-1BQ diagonalizes matrix B.  Find Q and Q^-1.

Solution:

• Concepts:
  Matrix algebra
• Reasoning:
  This is a linear algebra problem.
• Details of the calculation:
  (a)  Both matrices are Hermitian and thus diagonalizable.
  (b)
  
  
  
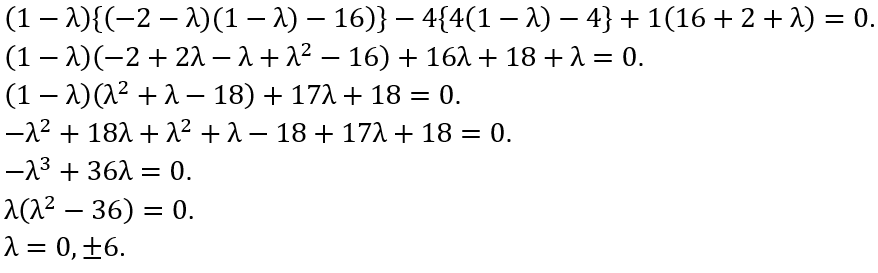
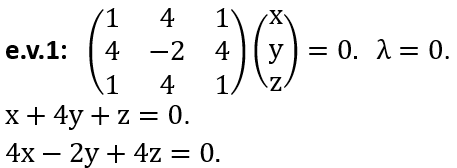
  The third equation is redundant.     
  Multiply first equation by a factor of 4 and subtract:
  --> y = 0.  Then x = -z.
  eigenvector 1:
   
  
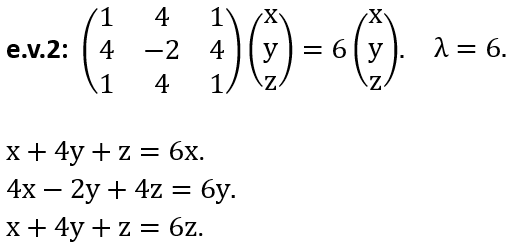
  Solving this system of equations yields x = y = z.
  eigenvector 2:
  
  
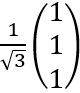
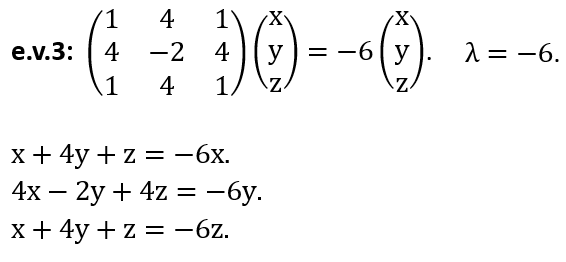
  Solving this system of equations yields x = z, y = -2x.
  eigenvector 3:
  
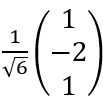
  Note that all three vectors are orthogonal, as they should since  are non-degenerate.
  (c)
  
  
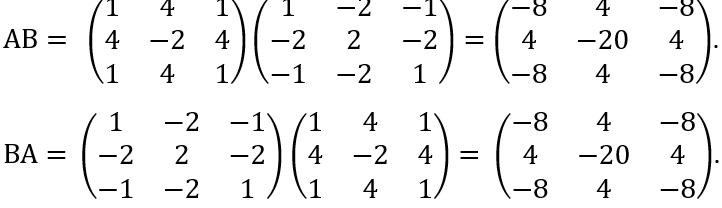
  Therefore [A,B] = 0.
  (d)  Since A and B commute, they have a common set of eigenvectors.  Hence the eigenvectors of A form the columns of the matrix Q that diagonalizes matrix B.  In other words, B' = Q^-1BQ is a diagonal matrix with the eigenvalues of B appearing on the diagonal.
  If we use the normalized eigenvectors of A, then Q will be unitary, which makes it easy to Q^-1 = Q^†.
  
  

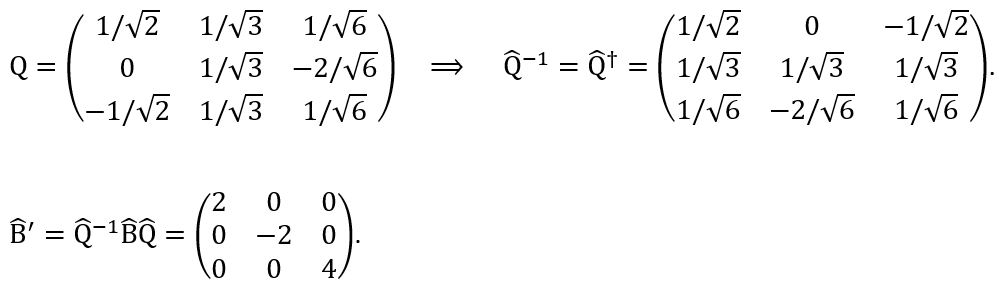
Problem 2:

You prepare an ensemble of identical experiments at t = 0.  You measure the values of a physical observable A at time t = t₁ > 0 in all of the experiments of the ensemble.  At time t₂ > t₁ you measure observable A again in all of the experiments, i.e. you follow the measurement at t = t₁ in each experiment with a subsequent measurement of A in each experiment at t = t₂.

True or False: (Explain your choice in one or two sentences.)
(a)  The values you obtain for A across all of the experiments at time t = t₁ are the same given you have made all of the measurements at the same time t = t₁.
(b)  The value you obtain for A in each experiment at t = t₂ may be different relative to the value obtained for A in the same experiment at t = t₁.
(c)  The expectation value of A is the same at both t = t₁ and t = t₂.
(d)  The values you obtain for A in any of the experiments at either t = t₁ or t = t₂ must be one of the eigenvalues of its associated Hermitian operator.

At t = t₃ > t₂ you measure a second physical observable B across all of the experiments, i.e. you follow the measurement of A at t = t₂ in each experiment with a measurement of B at t = t₃ in each experiment.  The commutator of the two Hermitian operators associated with A and B satisfies [A, B] ≠ 0.

True or False:
(e)  The values you obtained for A at t = t₂ in any of these experiments will remain the same at t = t₃ given you are measuring a different physical quantity B, not A.

Now you reset all of the experiments to what they were at t = 0, i.e., you start all over again with the same initial set of identical experiments.  At time t = t₄, you measure A again in all of your experiments.  Moreover, the commutator of the operator associated with A and the Hamiltonian operator satisfies
[H, A] = 0 .

True or False:
(f)  The value you obtain for A in each experiment at t = t₄ is the same as the value you obtained for A in the corresponding experiment at t = t₁.
(g)  The expectation values of A at t = t and t = t₄ are the same.

Solution:

• Concepts:
  Fundamental assumptions of quantum mechanics
• Reasoning:
  The expectation value of an observable A is constant in time if A and H commute.
• Details of the calculation:
  (a)  False.  The experiment may have been prepared in a superposition of eigenstates of A.
  (b)  True.   A may not commute with H.
  (c)  False.  In the Schroedinger picture d/dt)<Ψ(t)|A|Ψ(t)> = (iħ)^-1<Ψ(t)|[A, H]|Ψ(t)>, if A does not explicitly depend on time.  If [A,H] ≠ 0, then the expectation value of A is time-dependent.
  (d)  True.  A measurement of an observable always yields an eigenvalue of the observable.
  (e)  False.  A measurement of B leaves the system in an eigenstates of B.  A and B do not have a common eigenbasis.
  (f)   False.  The experiment may have been prepared in a superposition of eigenstates of A.
  (g)  True.  See (c).   If [A,H] = 0, then the expectation value of A is time-independent.

Problem 3:

Consider a two-state system governed by the Hamiltonian H with energy eigenstates |E₁> and |E₂>,  where H|E₁> = E₁|E₁> and H|E₂> = E₂ |E₂>.  Consider also two other states,
|x> = (|E₁> + |E₂>)/√2,  and  |y> = (|E₁> - |E₂>)/√2.
At time t = 0 the system is in state |x>.  At what subsequent times is the probability of finding the system in state |y> the largest, and what is that probability?

Solution:

• Concepts:
  The evolution operator, postulates of QM
• Reasoning:
  The evolution operator is a unitary operator defined through |ψ(t)> = U(t,t₀)|ψ(t₀)>.
  If H does not explicitly depend on time, then U(t,t₀) = exp(-(i/ħ)H(t-t₀)).
  If |ψ(t₀)> is an eigenfunction of H with eigenvalue E,
  then |ψ(t)> =  exp(-(i/ħ)E(t-t₀))|ψ(t₀)>.
• Details of the calculation:
  |ψ(0)>  = |x> = (|E₁> + |E₂>)/√2, |ψ(t)> = exp(-iHt/ħ)|ψ(0)>
  = (exp(-iE₁t/ħ)|E₁> + exp(-iE₂t/ħ)|E₂>)/√2.
  <y|ψ(t)> = (<E₁|ψ(t)>  - <E₂|ψ(t)>) /√2 = (exp(-iE₁t/ħ) - exp(-iE₂t/ħ))/2
  = exp(-iE₁t/ħ) (1 - exp(-i(E₂ - E₁)t/ħ))/2.
  Probability of finding the system in state |y>:
  P_y = |<y|ψ(t)>|² = (1 - exp(-i(E₂ - E₁)t/ħ))(1 - exp(+i(E₂ - E₁)t/ħ))/4
  = ½(1 - cos((E₂ - E₁)t/ħ)).
  The probability P_y is maximized when cos((E₂ - E₁)t/ħ) = -1, or when t = nπħ/( E₂ - E₁), n = 1, 3, 5, ... .
  Then P_y = 1.

Problem 4:

Consider the one-dimensional time-independent Schroedinger equation for some arbitrary potential U(x).  Prove that if a solution ψ(x) has the property that ψ(x) → 0 as x → ±∞, then the solution must be non-degenerate and therefore real, apart from a possible overall phase factor.
Hint:  Show that the contrary assumption leads to a contradiction.

Solution:

• Concepts:
  The time-independent Schroedinger equation
• Reasoning:
  If ψ(x) is a solution of the time-independent Schroedinger equation, i.e. of the eigenfunction of the Hamiltonian H(x),
  then ∂²ψ(x)/∂x² - (2m/ħ²)(E - U(x))ψ(x) = 0.
• Details of the calculation:
  Assume two solutions ψ₁(x) and ψ₂(x) exist.
  The Schroedinger equation tells us that
  ∂²ψ₁(x)/∂x² - (2m/ħ²)(E - U(x))ψ₁(x) = 0,
  ∂²ψ₂(x)/∂x² - (2m/ħ²)(E - U(x))ψ₂(x) = 0.
  Multiplying the first equation by ψ₂(x) and the second equation by ψ₁(x) and subtracting we obtain
  ψ₂(x)∂²ψ₁(x)/∂x² - ψ₁(x)∂²ψ₂(x)/∂x² = 0.
  ∂/∂x(ψ₂(x) ∂ψ₁(x)/∂x - ψ₁(x) ∂ψ₂(x)/∂x) = ψ₂(x) ∂²ψ₁(x)/∂x² - ψ₁(x)∂²ψ₂(x)/∂x² = 0.
  Therefore ψ₂(x) ∂ψ₁(x)/∂x - ψ₁(x) ∂ψ₂(x)/∂x = C - constant.  (Integration)
  For bound states the wave function must vanish as x --> ± ∞.  Therefore C = 0.
  ψ₂(x) ∂ψ₁(x)/∂x - ψ₁(x) ∂ψ₂(x)/∂x = 0.
  (1/ψ₁(x)) ∂ψ₁(x)/∂x = (1/ψ₂(x)) ∂ψ₂(x)/∂x.
  ∂/∂x(lnψ₁(x)) = ∂/∂x(lnψ₂(x)).
  This integrates to lnψ₁(x) = lnψ₂(x) + c,  ψ₂(x)  = γ ψ₁(x), where γ = e^c.
  ψ₂(x) can differ from ψ₁(x) only by a multiplicative constant.
  If ψ₁(x) and ψ₂(x) are normalized, then |γ|² = 1, γ = e^iδ, where δ is a real number.  If we choose δ = 0, then c = 1 and ψ is a real function.

Problem 5:

A particle in one dimension is in a stationary state.
(a)  Show that for any time-independent operator Q, the expectation or mean value <Q> is independent of time.
(b)  Show that the mean value of the particles momentum, <p>, is zero.

Solution

• Concepts:
  The postulates of QM
• Reasoning:
  Hψ(x.t) = iħ∂ψ(x,t)/∂t.  Separation of variable is possible.  ψ(x,t) = ψ(x)exp(-iEt/ħ).
  For a stationary state Hψ(x) = Eψ(x).  ψ(x) can be chosen to be real.  ψ(±∞) = 0.
  [ψ(x) is the solution to a real differential equation.
  For any solution one can construct a second solution by taking the complex conjugate ψ*(x).
  ψ(x) and ψ*(x) are either linearly dependent and differ at most by a phase, or they are linearly independent, in which case you can construct two independent real-valued solutions ½(ψ(x) + ψ*(x)) = Re(ψ) and ½(ψ(x) - ψ*(x)) = Im(ψ).] 
• Details of the calculation:
  (a)  <Q(t)> = ∫_-∞^∞ dx ψ*(x,t) Q ψ(x,t) = ∫_-∞^∞ dx ψ(x)exp(iEt/ħ) Q ψ(x)exp(-iEt/ħ)
  = ∫_-∞^∞ dx ψ(x)Q ψ(x) = <Q(0)>, since Q does not act on time.
  (b)  <p> = -iħ∫_-∞^∞ dx ψ*(x) ∂ψ(x)/∂x = -iħ∫_-∞^∞ dx ψ(x) ∂ψ(x)/∂x, since ψ(x) is real.
  <p> = -½iħ∫_-∞^∞ dx ∂ψ²(x)/∂x = -½iħψ²(x)|_-∞^∞ = 0.
  or
  [H, x] = -iħp/m
  <ψ|p|ψ> ∝ <ψ|[H, x]|ψ> = <ψ|(Hx - xH) |ψ> =  E<ψ|x|ψ> - E<ψ|x|ψ> = 0.