Problem 1:

Use the Euler-Lagrange equation to show that the shortest path between two points on a plane is a straight line.

Problem 2: 

A particle of mass m moves in one dimension under the influence of a force F(x,t) = (k/x²)exp(-t/τ), where k and τ are positive constants.  Compute the Lagrangian and the Hamiltonian, and discuss whether energy is conserved in this system.

Problem 3:

(a)  Show that two Lagrangians L₁ and L_2, which differ only by the total derivative of a function of q and t, i.e.  L₂ = L₁ + df(q,t)/dt, describe the same motion for q.
(b)  Find the Lagrangian and Hamiltonian of a pendulum consisting of a mass m attached to a massless rigid rod AB of length l free to move in a vertical plane.  The end A of the rod is forced to move vertically, so that its displacement from the fixed point O is a given function of time γ(t).  Gravity acts vertically downward.
(c)  Show that the vertical acceleration of the point A, d²γ(t)/dt², has the same effect on the equation of motion as a time varying gravitational field.

Problem 4:

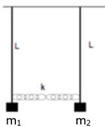
Two pendulums of length L have masses m₁ and m₂ attached to their ends.  They are also connected to each other by a spring with spring constant k, as shown in the figure.  The equilibrium positions of the pendulums are vertical as shown.  Assume small displacements.
(a)  Write down the Lagrangian for the system
(b)  Find the normal mode frequencies for the system.
(c)  At t = 0, pendulum 1 is displaced by an angle θ₁, while pendulum 2
is at its vertical position. 
Find the subsequent angular position of each as a function of time.

Problem 5:

A point mass m₁ is constrained to move on a horizontal wire without friction. 
A second point mass m₂ is attached to it by a rod of negligible mass and length L.

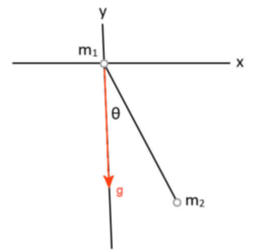
(a)  Write down the Lagrangian of the system and find the equations of motion.
(b)  Are there any equilibrium points for the rotational motion?  Are they stable?  If yes, find the frequency of small oscillations about the equilibrium points.
(c)  Now assume the wire is not horizontal, but makes an angle φ with the horizontal direction.  Find the equations of motion.  Are there any equilibrium points for the rotational motion?  Are they stable?  If yes, find the frequency of small oscillations about the equilibrium points.