More Problems

Problem 1:

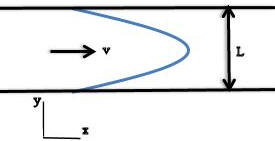
A boat is moving across a river of constant with L.  The boat moves with constant velocity v₁ relative to the water and perpendicular to the current.  The current is parallel to the the river bank and the speed v of the water of depends on the distance from the river bank as v = v₀sin(πy/L), where v₀ is a constant.  Find the velocity vector v(x,y) of the boat with respect to the river bank and the trajectory y(x) of the boat.  Use the orientation of the coordinate axes shown below.

Solution:

• Concepts:
  Kinematics
• Reasoning:
  We have two coupled first-order differential equations.
• Details of the calculation:
  v_y = dy/dt = v₁,  y = v₁t.
  v_x = dx/dt = v₀sin(πy/L) = v₀sin(πv₁t/L).
  v(x,y) = v₀sin(πy/L) i + v₁ j.
  x(y) = ∫₀^y(dx/dy')dy' = ∫₀^y(dx/dt)(dt/dy')dy' = (1/v₁)∫₀^yv_xdy' = (v₀/v₁)∫₀^ysin(πy'/L) dy'.
  x(y) = [Lv₀/(πv₁)][1 - cos(πy/L)].
  Trajectory y(x) =  (L/π)cos^-1[1 - πv₁x/(Lv₀)].

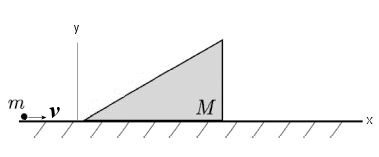
Problem 2:

A wedge of mass M rests on a frictionless horizontal surface.  A point mass m < M moves without friction with velocity v i and approaches the wedge.
(a)  What is the final velocity of the point mass in terms of the given quantities?
(b)  What is the maximum height the point mass will reach before reversing direction?
 

Solution:

• Concepts:
  Energy and momentum conservation
• Reasoning:
  Often collision problems are easiest solved by going to the CM frame.  Assume m is a point particle.
• Details of the calculation:
  (a)  In the CM frame the initial and the final momentum is zero.
  In the CM frame initially m(v + V) + MV = 0
  mv + mV = -MV, V = -mv/(m + M)
  V = -Vi is the velocity of the CM, the CM moves in the -x direction.
  In the CM frame the final velocity of the point mass is -(v + V)i.
  In the lab frame it is -(v + V)i - Vi = i(-v + 2mv/(m + M)) = -i v(M - m)/(M + m).
  
  (b)  In the CM frame:
  Energy conservation:
  &frac12;m(v + V)² + &frac12;MV² = mgh.
  &frac12;m(v - mv/(m + M))² + &frac12;M(mv/(m + M))² = mgh.
  m(Mv/(m + M))² + M(mv/(m + M))² = 2mgh.
  mM²v²/(m + M)² + Mm²v²/(m + M)² = 2mgh.
  M²v²/(m + M)² + Mmv²/(m + M)² = 2gh.
  h  = &frac12;(v²/g)(M²  + mM)/(M + m)²
  = &frac12;(v²/g)(M² + 2mM + m² - mM -m²)/(M + m)²
  = &frac12;(v²/g)(1 - m/(M + m)).
  h  = &frac12;(v²/g)M/(M + m) is the maximum height the point mass will reach.
  
  Or
  In the lab frame:
  mv = (M + m)u  (momentum conservation)
  &frac12;mv² = &frac12;(M + m)u² + mgh. (energy conservation)
  v² = mv²/(M + m) + 2gh.
  Mv²/(M + m) = 2gh.  h  = &frac12;(v²/g)M/(M + m).

Problem 3:

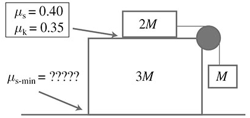
A system of three blocks of different masses is placed on a horizontal floor.  Blocks 2M and M are connected by a light string that passes over a frictionless pulley mounted on block 3M.  Block 2M is initially held in place. Find the minimum value of the coefficient of static friction between block 3M and the floor that allows block 3M to remain at rest after block 2M is released.  The coefficients of friction between the block of mass 3M and that of mass 2M are given.

Solution:

• Concepts:
  Newton's second law, free body diagrams
• Reasoning:
  Draw a free body diagram for each of the blocks and make sure all forces are included.
• Details of the calculation:
  Assume the frictional force f₃ is large enough so that block 3M stays at rest.
  Let the y-axis point up and the x-axis point to the right.
  
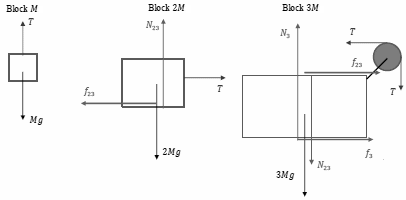
  Block M:  T - Mg = Ma_1y.
  Block 2M:  T - f₂₃ = 2Ma_2x.  N₂₃ - 2Mg = 0.  N₂₃ = 2Mg.
  Block 3M:  f₂₃ + f₃ - T = 0.  N₃ - 3Mg - N₂₃ - T = 0.
  Since blocks M and 2M are connected, -a_1y = a_2x = a.
  
  Can block 2M stay at rest, i.e. can the whole system stay art rest? 
  If block 2M stays at rest, then a = 0, T = Mg = f₂₃.
  But f₂₃ < μ_sN₂₃ = μ_s2Mg = 0.8 Mg, so block 2M cannot stay at rest.
  If block 2M does not stay at rest we have f₂₃ = μ_kN₂₃ = μ_k2Mg.
  Our equations then can be rewritten as
  T - Mg = -Ma. 
  T - μ_k2Mg = 2Ma.
  μ_k2Mg + f₃ - T = 0.
  N₃ - 3Mg - 2Mg - T = 0.
  We have 4 equations for 4 unknowns, T, a, f₃, and N₃.
  We want to solve for f₃ and N₃.
  Eliminating a and T we find
  f₃ = (2/3 - 4μ_k/3)Mg = 0.2 Mg,
  N₃ = (5 + 2/3 + 2μ_k/3) Mg = 5.9 Mg.
  We need the coefficient of static friction between block 3M and the floor μ_3s ≥ f₃/N₃ = 0.0339.