Review Problems

Problem 1:

Problem 2:

A ball of mass m = 5 kg, radius R = 10 cm, and uniform density is at rest on a perfectly rough "moving walkway" that has been stopped for repair.  At t = 0, the walkway starts accelerating with acceleration a = 0.2 m/s.  Find the acceleration of the ball in the "moving walkway" frame and in the frame of the stationary floor.

Solution:

• Concepts:
  Motion in a non-inertial frame
• Reasoning:
  In an accelerating frame fictitious forces appear.  The net force in such a frame is
  F = F_inertial - ma, where a is the acceleration of the frame.
• Details of the calculation:
  Let the acceleration of the walkway point in the x-direction.
  In the walkway frame, the net force on the ball is F = -ma + f = ma' where f is the force of static friction preventing the contact point from slipping and a' is the acceleration of the CM of the ball.  Friction exerts a torque on the ball, |τ| = fR = I|α| = I|a'|/R.
  If the z-axis points up this torque points in the -y direction.
  I = (2/5)mR²,  f = -Ia'/R² = -(2/5)ma', since f points in the positive and a' in the negative x-direction.
  -ma = ma' + (2/5)ma'.  a' = -5a/7.
  The acceleration of the ball in the frame of the stationary floor is a'' = a + a' = 2a/7.

Problem 3:

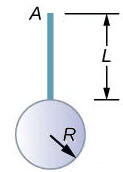
A disk of radius a and mass M is connected to a rod of mass m and length L as shown.  Both the rod and the disk have uniform density.  Find the moment of inertia of the compound object about an axis though the end labeled A and perpendicular to the plane of the disk.

Solution:

• Concepts:
  The moment of inertia, the parallel axis theorem
• Reasoning:
  The parallel-axis theorem states that if I_CM is the moment-of-inertia of an object about an axis through its center of mass, then I, the moment of inertia about any axis parallel to that first one is given by I = I_CM + md², where m is the object's mass and d is the perpendicular distance between the two axes.
• Details of the calculation:
  The moment of inertia of the rod about point A is (1/3)mL².
  The moment of inertia of the disk about its center is ½MR².
  Using the parallel axis theorem we have for the moment of inertia of the compound object about point A
  I = (1/3)mL² + ½MR² + M(L + R)² = (1/3)mL² + (3/2)MR² + ML² + 2MRL.

Problem 4:

Find the eigenvalues and normalized eigenvectors of this Hamiltonian.

Solution:

• Concepts:
  The eigenvalues and eigenvectors of a Hermitian operator
• Reasoning:
  We are given the matrix of the Hermitian operator H in some basis.  To find the eigenvalues E we set the determinant of the matrix (H - EI) equal to zero and solve for E.  To find the corresponding eigenvectors {|Ψ>}, we substitute each eigenvalue E back into the equation (H - EI)|Ψ> and solve for the expansion coefficients of |Ψ> in the given basis.
• Details of the calculation:
  (a)  The eigenvalues are λE₀, where
  

  	1 - λ	2i
  	-2i	6 - λ

    = 0.

  
  
  

  (1 - λ)(6 - λ) - 4 = 0,  λ² - 7λ + 2  = 0,  λ₁ = (7 + (41)^½)/2,  λ₂ = (7 - (41)^½)/2.
  The normalized eigenvector corresponding to λ₁ is |1'> = a|1> + b|2>,
  where a(1 - λ₁) + i2b = 0, and |a|² + |b|² = 1.
  -a(1 - (7 + (41)^½)/2)/(2i) = b,  b = -i2.85a,
  |a|²(1 + 2.85²) = 1, |a|² = 0.11, |b|² = 0.89,  |1'> = 0.33|1> - i0.94|2>.
  
  The normalized eigenvector corresponding to λ₂ is |2'> = c|1> + d|2>,
  where c(1 - λ₂) + i2c = 0, and |c|² + |d|² = 1.
  -c(1 - (7 - (41)^½)/2)/(2i) = d,  d = i0.35a,
  |c|²(1 + 0.35²) = 1, |c|² = 0.89, |d|² = 0.11,  |1'> = 0.94|1> + i0.33|2>.

Problem 5:

Consider a pendulum consisting of a mass m attached to one end of a massless string of length ℓ.  The other end of the string is fixed to the uppermost point of a fixed vertical disk of radius R.  Assume that πR < ℓ. 

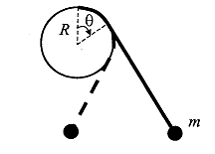
(a)  Find the equation of motion in terms of the angle θ as shown in the figure.
(b)  What is the equilibrium angle θ₀?
(c)  Find the frequency of small oscillations about the equilibrium angle.

Solution:

• Concepts:
  Lagrangian mechanics
• Reasoning:
  All forces except the forces of constraint are derivable from a potential.  The Lagrangian formalism is well suited for such a system.
• Details of the calculations:
  (a)  The Cartesian coordinates of m are
  x = R sinθ + (ℓ - Rθ) cosθ,  y = -R(1 - cosθ) - (ℓ - Rθ) sinθ.
  Therefore
  dx/dt = -(ℓ - Rθ) sinθ dθ/dt,  dy/dt = -(ℓ - Rθ) cosθ dθ/dt.
  L = T - U = ½m[(dx/dt)² + (dy/dt)²] - mgy.
  L = ½m(ℓ - Rθ)²(dθ/dt)² + mgR(1- cosθ) + mg(ℓ - Rθ) sinθ.
  equation of motion:  d/dt(∂L/∂(dθ/dt)) -  ∂L/∂θ = 0.
  ∂L/∂(dθ/dt) = m(ℓ - Rθ)²(dθ/dt),
  d/dt(∂L/∂(dθ/dt)) = -2m(ℓ - Rθ) R(dθ/dt)² + m(ℓ - Rθ)²d²θ/dt².
  ∂L/∂θ = -m(ℓ - Rθ)[R(dθ/dt)² - gcosθ].
  Lagrange's equation becomes 
  (ℓ - Rθ) d²θ/dt² - R(dθ/dt)² - gcosθ = 0.
  
  (b)  dθ/dt = d²θ/dt² = 0  -->  cosθ = 0,  θ = π/2.
  
  (c)  Let θ = π/2 + ε,  ε << 1,  then cosθ = -sinε ~ -ε.
  Keeping only terms to first order in ε Lagrange's equation becomes
  (ℓ - Rπ/2) d²ε/dt² + gε = 0.  d²ε/dt² = -ω²ε.
  ω² = g/(ℓ - Rπ/2).

Problem 6:

Find the commutator between the square of the coordinate x and the momentum p operators i.e. find [x², p].  You can use the trick of thinking of this commutator as acting over a "test function" f(x) if it helps.

Solution:

• Concepts:
  Commutator algebra
• Reasoning:
  [x, p] = iħ.
• Details of the calculation:
  [x², p] = x[x, p] + [x, p]x  = 2iħx.