More Problems

Problem 1:

A rectangular block of dielectric material with permittivity ε is partially inserted between two parallel plane conducting plates.  The plates are square, of side l, and are separated by a distance d, with d << l.  The dielectric is also square, of side l, and has a thickness of almost d.  A potential V₀ is applied across the plates by a battery.

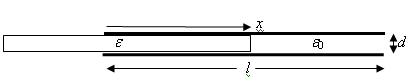
(a)  When the dielectric has a length x inserted between the plates, calculate the force on the dielectric, including its direction.  If x is now changed, does this force depend on x?
(b)  When the dielectric has a length x inserted between the plates, the battery is disconnected.  Calculate the force on the dielectric, including its direction.  If x is now changed, does this force depend on x?

Solution:

• Concepts:
  Capacitance, energy and work
• Reasoning:
  F_x = -dW_mech/dx.  We must find the work done by external agents as a dielectric is inserted into a capacitor and the capacitance changes.
• Details of the calculation:
  (a)  Capacitance as a function of x:  C = εlx/d  + ε₀l(l -x)/d.
  Total energy store in the capacitor:  U = ½CV₀².
  dW_mech + dW_bat = dU.  dW_bat = dQV₀ = dCV₀².  dU = ½dCV₀². 
  dW_mech = -½dCV₀². 
  F_x = -dW_mech/dx = ½V₀²dC/dx = ½V₀²(ε -  ε₀)l/d.
  The dielectric is pulled towards the right, into the capacitor.  The force is independent of x.
  
  (b)  When the battery is disconnected with the dielectric inserted a distance x, the force is
  F_x = ½V₀²(ε -  ε₀)l/d.  At that moment the voltage and the distances are the same as for case (a).
  But when x changes, Q, not V, stays constant.  We therefore write
  F_x = ½(Q₀/C)²(ε -  ε₀)l/d,  where Q₀ = C_initialV₀.
  F_x = ½Q₀²(ε -  ε₀)ld/(εlx  + ε₀l(l -x))².  Since C depends on x,  F_x depends on x.
  
  Note:
  We can also find F_x for part (b) by writing dW_mech = dU,  U = ½Q₀²/C.
  F_x = -dW_mech/dx = -dU/dx = ½(Q₀²/C²)dC/dx = ½(Q₀/C)²(ε -  ε₀)l/d.

Problem 2:

A circuit contains three resistors, two ideal ammeters, and an ideal battery.  The readings of the ammeters are 0.40 A and 0.60 A.  After two of the resistors are swapped with each other, the reading of each ammeter remains the same.  Find the battery current.

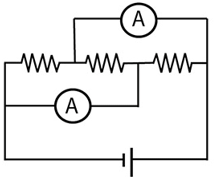
Solution:

• Concepts:
  DC circuits,  Kirchhoff's rules
• Reasoning:
  Ideal ammeters act like wires with no resistance.  Label the points in the circuit that are at the same potential.
• Details of the calculation:
  The figure below shows the junctions that are at the same potential.  We see that we just have 3 resistors in parallel.
  
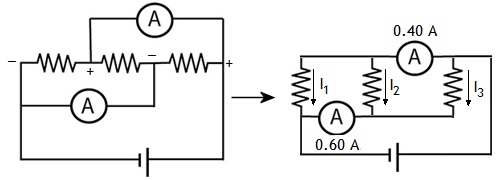
  The voltage across each resistor is the same.  The current through resistor R_i is I_i = V/R_i.  The total current is I = I₁ + I₂ + I₃.
  Without loss of generality, we may arbitrarily assign the given currents to the individual ammeters.
  Apply the junction rule:  I₁ + I₂ = 0.4 A.  I₂ + I₃ = 0.6 A.
  This implies that not all three resistors are identical, since then the two ammeters would have the same reading.
  If R₁ and R₂ are switched, the reading of the upper Ammeter does not change, but the reading of the lower ammeter changes unless R₁ = R₂.
  If R₂ and R₃ are switched, the reading of the lower Ammeter does not change, but the reading of the upper ammeter changes unless R₂ = R₃.
  If R₁ and R₃ are switched, then the readings of both meters change unless R₁ = R₃.  But then both ammeters would have the same reading.
  We therefore conclude that either R₁ = R₂ or R₂ = R₃.
  Case R₁ = R₂:   I₁ = I₂ = 0.2 A.  I₃ = 0.4 A.  I = 0.8 A = battery current.
  Case R₂ = R₃:   I₂ = I₃ = 0.3 A.  I₁ = 0.1 A.  I = 0.7 A = battery current.

Problem 3:

Given the potential function V(x,y,z) = 2x + 4y Volts in free space, find an explicit numerical value for the stored energy in a volume of 1 m³ centered at the origin. Compare this value with other 1 m³ volumes elsewhere.

Solution:

• Concepts:
  Electrostatic energy
• Reasoning:
  E = -∇V,  energy density in the electric field:  u(r) = (ε₀/2)E²(r)
• Details of the calculation:
  E = -∇V = -∂V/∂x i - ∂V/∂y j - ∂V/∂z k = (-2 i + 4 j) V/m.
  E² = (4 + 16) (V/m)² = 20 (V/m)².
  U = (ε₀/2)∫_V E² dV = 10ε₀ (V/m)²*(1 m³) = 8.85 *10^-11 J,
  independent of where the volume is located.