More Problems

Problem 1: 

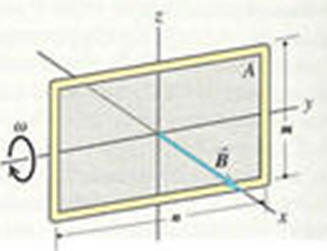The loop in the figure has dimension m times n as shown and resistance R.  In a time interval Δt it is rotated with uniform angular speed ω = π/Δt by 180^o about the y-axis in a uniform magnetic field of magnitude B, oriented along the x-axis as shown.  
(a)  What is the average emf induced in the loop during the rotation?
(b)  How much work was done to rotate the loop.

Solution:

• Concepts:
  Faraday's law, induced emf
• Reasoning:
  The magnetic flux through a filamentary loop is changing with time.  This flux change induces an emf and causes a current to flow.
• Details of the calculation:
  (a)  For filamentary circuits:  emf = -d/dt(∫B∙ndA) = -d/dt(BAcos(ωt)) = ωBAsin(ωt) = ωBm*nsin(ωt).
  <emf> = ωBm*n<sin(ωt)> = (ωBm*n/π)∫₀^π sinx dx = 2ωBm*n/π with ωΔt = π
  Here the average magnitude of the emf therefore is <emf> = 2 B m*n/Δt.
  
  (b)  I = emf/R,  dW/dt = IR = (ωBm*n)²sin²(ωt),
  W = (ωBm*n)²∫₀^Δt sin²(ωt)dt = ω(Bm*n)²∫₀^π sin²(x)dx.
  The work done is W = ωπ(Bm*n)²/2.

Problem 2:

A long solenoid carries a current of I = 2 A.  Its length is L = 10 cm and its cross-sectional is A = 0.25 cm².  The solenoid has N = 15000 turns.  What is the magnetostatic energy stored in the solenoid, and what is its self-inductance?

Solution:

• Concepts:
  The magnetic field inside a solenoid, magnetostatic energy
• Reasoning:
  Approximation:  B = μ₀nI inside the solenoid,  B = 0 outside.
• Details of the calculation:
  U = (2μ₀)∫_{all space}B²dV.
  The integral over all space reduces to an integral over the volume of the solenoid.
  U = (1/(2μ₀))B²V = μ₀n²I²LA/2.
  n = N/L.  U = μ₀N²I²A/(2L) = 0.141 J
  U_L = ½LI²,  L = 2U/I² = 7.07*10^-2 H.

Problem 3:

A particle of charge q and mass m moves in a region containing a uniform electric field
E = Ei pointing in the x-direction and a uniform magnetic field B = Bk pointing in the z-direction.

(a)  Write down the equation of motion for the particle and find the general solutions for the Cartesian velocity components v_i(t) in terms of B, E, q, and m.
Hint:  Let ζ = v_x + iv_y, and solve for ζ(t).
(b)  Solve for the position of the particle as a function of time if the particle is released from rest at t = 0.

Solution:

• Concepts:
  The Lorentz force, F = q(E + v × B) = mdv/dt
• Reasoning:
  dv_z/dt = 0, v_z = constant.  We have to solve coupled differential equations for v_x and v_y using the hint, ζ = v_x + iv_y.
• Details of the calculation:
  (a)  dv_x/dt = qv_yB/m + (qE/m).
  dv_y/dt = -qv_xB/m.
  Let ζ  = v_x + iv_y.
  dv_x/dt + idv_y/dt = dζ/dt = qv_yB/m + (qE/m) - iqv_xB/m
  = -i(iqv_yB/m + qv_xB/m) + (qE/m) = -i(qB/m)ζ + (qE/m).
  dζ/dt + i(qB/m)ζ = (qE/m).
  To a particular solution of the first-order inhomogeneous differential equation,
  dζ/dt + i(qB/m)ζ = (qE/m), we add the solution of the homogeneous differential equation,
  dζ/dt + i(qB/m)ζ = 0, to find the most general solution.
  
  An inhomogeneous solution:
  dζ/dt = 0, ζ = ζ₀,  ζ₀ = -i(E/B).
  homogeneous solution:
  ζ = Aexp(-iωt), A = arbitrary complex constant = |A|exp(iφ),  ω = qB/m.
  general solution:
  ζ = |A|exp(-iωt + φ) - i(qE/m).
  v_x(t) = |A|cos(ωt + φ),
  v_y(t) = -|A|sin(ωt + φ) - (E/B).
  
  (b) v_z(0) = 0 --> v_z(t) = 0_{.
  Vx}(0 = 0, v_y(0) = 0 -- > φ = -π/2, |A| = E/B.
  v_x(t) = (E/B)sin(ωt),  v_y(t) = (E/B)cos(ωt) - (E/B).
  x(t) = ∫₀^t v_x(t')dt' = (mE/(qB²))(1 - cos(qBt/m)),  
  y(t) = ∫₀^t v_y(t')dt' = -Et/B +  (mE/(qB²))(sin(qBt/m).