More Problems

Problem 1:

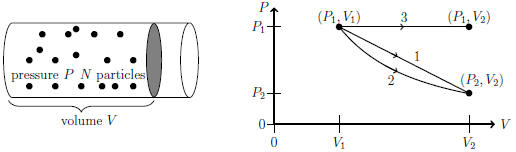
Consider an ideal gas of N particles in a cylinder with a piston so that the volume and pressure may change.  Suppose that each particle has f = 5 quadratic degrees of freedom in its energy.  Consider the three paths in a PV diagram shown.  Suppose ways 1 and 3 are straight lines on the PV diagram and way 2 is an adiabatic process.
(a)  How much work W is done on the gas for ways 1, 2, and 3?
(b)  How much heat Q is transferred to the gas for ways 1, 2, and 3?
(c)  What is the change in internal energy ΔU for ways 1, 2, 3?
(d)  What is the change in entropy ΔS for way 3?

Solution:

• Concepts:
  The ideal gas law PV = NkT,  work done by the system W = ∫PdV,  the first law of thermodynamics, entropy
• Reasoning:
  We are asked to compute ΔU, ΔQ, W, and ΔS for along different path on a PV diagram.
• Details of the calculation:
  (a)  W = -∫PdV = work done on the gas = - area under the curve on a PV diagram.
  First law of thermodynamics:  ΔU = ΔQ + W. 
  way 1:  W = -½(P₁ + P₂)(V₂ - V₁).
  way 2:  W = ΔU = (5/2)Nk(T₂ - T₁) = (5/2)(P₂V₂ - P₁V₁).
  way 3:  W = -P₁(V₂ - V₁).
  
  (b)  ΔQ = ΔU - W.
  way 1:  ΔQ =  (5/2)(P₂V₂ - P₁V₁) + ½(P₁ + P₂)(V₂ - V₁)
  = 3(P₂V₂ - P₁V₁) - ½(P₂V₁ - P₁V₂).
  way 2:  ΔQ = 0.
  way 3:  ΔQ = (5/2)(P₁V₂ - P₁V₁) + P₁(V₂ - V₁)
  = (7/2)P₁(V₂ + V₁).
  
  (c)  ΔU is a physical property of the system.  It depend only on the state of the system, not on the way the system was put into this state.
  ways 1 and 2:  ΔU = (5/2)(P₂V₂ - P₁V₁)
  way 3:  ΔU = (5/2)(P₁V₂ - P₁V₁)
  
  (d)  Change in entropy: ΔS = ∫_i^f dS = ∫_i^f dQ_r/T.
  way 3:  dQ = dU - dW = (5/2)NkdT + NkdT = (7/2)NkdT.
  ΔS = (7/2)Nkln(T₂/T₁).
  ΔS = (7/2)Nkln(V₂/V₁).

Problem 2:

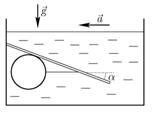A hollow ball with a volume V is held in place in a tank under water by a wire under a sloped plank as shown in the figure.  The water density is ρ and average ball density is ρ/5.  The plank makes an angle α with the horizontal, with tan(α) = 1/3.   What is the tension in the wire if the whole system is accelerating horizontally with acceleration a = g/6.

Solution:

• Concepts:
  Free-body diagrams, buoyancy
• Reasoning:
  Let the x-axis point towards the right and the y-axis point up.  For the net force F on the ball we have F_y = 0, F_x = -ma.
• Details of the calculation:
  The buoyant force acting on the ball is B_t = ρVg j - ρVa i.
  The buoyant force is the force the surrounding water would have to exert on the displaced water to accomplish the state of motion given in the problem in the absence of the object.
  F_y = -mg + B_y - Ncosα = -ρVg/5 + ρVg - Ncosα = 4ρVg/5 - Ncosα = 0.
  N = 4ρVg/(5cosα).
  F_x = -Nsinα + T + B_x = -ma. 
  T = -ρVg/30 + 4ρVg/15 + ρVg/6 = 2ρVg/5.

Problem 3:

A thermodynamic system consists of an ideal monatomic gas confined in a cylinder by a frictionless piston.  What physical process would have to be carried out to change the temperature at constant entropy S₁ from an initial value T₁ to a greater final value T₂ and what physical conditions would have to be satisfied by the all of the container?
Give a quantitative expression for the physical change in terms of T₁ and T₂.

Solution:

• Concepts:
  Entropy, internal energy, gas laws
• Reasoning:
  dS = dQ/T  Keeping the entropy constant implies an adiabatic process.
  PV = Nk_BT,  U = N(3/2) PV,  dU = dQ - dW.
  Increase in internal energy of a system
  = heat put into the system - work done by the system on its surroundings,
• Details of the calculation:
  dS = 0 --> dQ = 0.  Work has to be done on the gas to raise the temperature.  The gas has to be compressed, the volume has to decrease.
  dU = -dW = -PdV.
  But we also have U = (3/2)PV, dU = (3/2)(PdV + VdP). 
  [U = N½m<v²> = N(3/2)kT  = (3/2)PV.]
  Equating our two expressions for dU we have 
  -PdV = (3/2)(PdV + VdP),  (-5/2)PdV = (3/2)VdP,  dP/P + (5/3)dV/V = 0. 
  We can integrate to obtain lnP + (5/3)lnV = lnC, where lnC is the constant of integration.
  This yields PV^5/3 = C = constant.
  P₁/P₂ = (V₂/V₁)^5/3.
  From the ideal gas law P₁/P₂ = (T₁/T₂)(V₂/V₁).
  Therefore (V₂/V₁)^2/3 = (T₁/T₂),  (V₂/V₁) = (T₁/T₂)^3/2.