Problem 1:

With the aid of telescopes, astronomers were able to measure the distances to the nearer planets and asteroids using Earth's diameter as a baseline.  This is how the AU was first established.  To reach for the stars, however, requires a much longer baseline for triangulation and extremely sensitive measurements.  Such a baseline is provided by Earth's annual trip around the Sun.
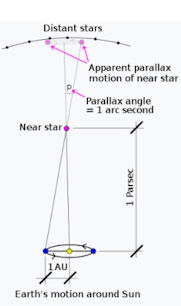
A star that has a parallax of 1 arcsec.  Calculate the distance from the sun to the star.  Calculate the distance in AU.  (The parallax angle is defined by 1 AU, not 2 AU,)

• Concepts:
  Triangulation
• Reasoning:
  The parallax angle is 1 arcsec = (1/3600) deg
  = (1/3600)(2π/360)rad.
• Details of the calculation:
  Paralax angle:  θ = 9.696*10^-6 rad.
  tanθ ≈ θ = 1 AU/d,  d = 206265 AU

Problem 2:

A photon rocket emits a beam of light instead of the hot gas of an ordinary rocket.  How powerful a light source would be needed for a photon rocket with thrust equal to that of a space shuttle (35 MN)?  Compare the power of this photon rocket with humanity's total electric power-generating capability, about 10 TW.

Solution:

• Concepts:
  Momentum conservation, F = dp/dt
• Reasoning:
  The magnitude of the total momentum of all photons emitted per second must be equal to the thrust (in an inertial frame instantaneously moving with the same speed as the shuttle, whose speed v/c << 1).
• Details of the calculation:
  p_total/s = 35*10⁶ N.
  The total photon energy/s is p_totalc/s = (35*10⁶ N)(3*10⁸ m/s) = 1.05*10¹⁶ W ≈ 10⁴ TW.
  The power of this photon rocket would exceed humanity's total electric power-generating capability by a factor of 1000.

Problem 3:

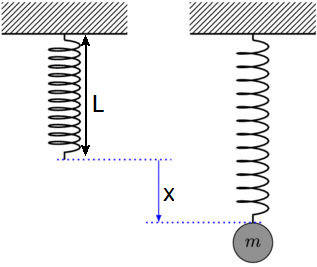
Show that when considering masses oscillating on vertical springs in a uniform gravitational field, the gravitational force does not appear in the equations of motions if we write these equations in terms of the displacements from the equilibrium positions in the gravitational field.

Solution:

• Concepts:
  Simple oscillations
• Reasoning:
  Assume a mass m is oscillating on a vertical spring of equilibrium length L and spring constant k.
• Details of the calculation:
  F = -kx + mg.  md²x/dt² = -kx + mg.
• The equilibrium position of the mass in the gravitation field id x₀ = mg/k.
  Let x' = x - x₀,  x = x' + x₀.
  md²x'/dt² = -kx'.
  This is the equation of motion for a harmonic oscillator.  The gravitational force does not appear in this equation.
  

Problem 4:

Determine the energy and the corresponding temperature T of thermal neutrons with the shortest de Broglie wave that can pass through a polycrystalline graphite, whose lattice constant is d = 0.335 nm.

Solution:

• Concepts:
  Bragg diffraction
• Reasoning:
  
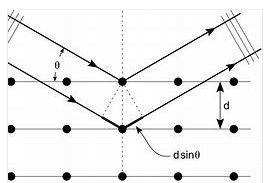
  The Bragg condition for constructive interference is nλ = 2d sinθ, where θ is the angle between the incident wave vector and the scattering planes.
  If the Bragg condition cannot be satisfied for any angle, the neutrons pass through the crystal without deflection.
• Details of the calculation:
  For a polycrystalline sample θ can range from 0 to 90^o.  So if λ > 2d the Bragg condition cannot be satisfied for any angle.
  The largest angle θ for a first order maximum can occur is θ = 90^o. 
  λ_min= 2d.
  Using de Broglie formula we can find the corresponding energy of neutrons.
  E_max = p²/2m = (h/λ)²/2m = h²/(8d²m) = (3/2)kT,
  T = h²/(12d²mk) = (6.62*10^-34)²/(12*(3.25*10^-10)²*1.675*10^-27*1.38*10^-23) K = 15 K.