More Problems

Problem 1:

The Euler-Lagrange differential equation is the fundamental equation of calculus of variations.  A typical problem consists of finding a real function y(x) of a real variable x such that a given function
J(y) = ∫_x1^x2f(x, y(x), y'(x)) dx,
where y'(x) = dy(x)/dx, has a stationary value.  The problem is to determine those functions y(x) which take given values at y₁ = y(x₁) and y₂ = y(x₂) at the end points and make J(y) an extremum.
Derive the Euler-Lagrange differential equation for f,  (∂f/∂y)  - d/dx(∂f/∂y') = 0.

Solution:

• Concepts:
  Euler-Lagrange differential equation
• Reasoning:
  This is a variational calculus problem.
• Details of the calculation:
  Investigate the quantity J(y) = ∫_x1^x2f(x, y(x), y'(x)) dx.
  Consider a small change δy(x) in the function y(x) subject to the condition that the endpoints are unchanged.
  δy(x₁) = δy(x₂) = 0.
  The first order variation in f is δf = (∂f/∂y)δy  + (∂f/∂y')δy', where δy' = (d/dx)δy.
  Therefore the variation in the integral is
  δJ = ∫_x1^x2dx [(∂f/∂y)δy  + (∂f/∂y') (d/dx)δy)]
  
  ∫_x1^x2dx [(∂f/∂y') (d/dx)δy] = (∂f/∂y')δy| _x1^x2 - ∫_x1^x2dx [d/dx(∂f/∂y')δy
  (integration by parts)
  δJ = ∫_x1^x2dx [∂f/∂y) -d/dx(∂f/∂y')]δy.
  For J to assume an extreme value, we need δJ = 0 for arbitrary variations δy.
  We therefore need ∂f/∂y) -d/dx(∂f/∂y') = 0.
  This is the Euler-Lagrange differential equation.

Problem 2:

A point mass m₁ is constrained to move on a horizontal wire without friction.  A second point mass m₂ is attached to it by a rod of negligible mass and length L.

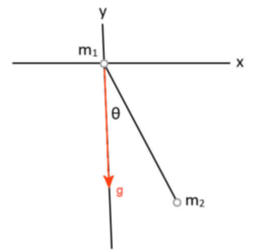
(a)  Describe qualitatively the possible motions of the system.  Assume the motions are confined to the xy-plane.
(b)  Find the Lagrangian of the system using the coordinates X and Y for motion of the CM of the system, and the coordinate θ for rotation about the CM.
(c)  How does the Lagrangian change if the wire is not horizontal, but makes an angle φ with the horizontal direction?

Solution:

• Concepts:
  Lagrangian mechanics
• Reasoning:
  L = T - U.
• Details of the calculation:
  (a)  F_x = 0, the x-component of the velocity of the CM must be constant. 
  Gravity and the normal force from the wire act in the y-direction. 
  We can have rotations about the CM, but they have to satisfy the constraint of keeping m₁ on the wire.
  (b)  Distance d of the CM from m₁:  m₁d = m₂(L - d), d = m₂L/(m₁ + m₂). 
  Let M = m₁ + m₂.
  T = ½M(X'² + Y'²) + ½Iθ'².  Here I = m₁d²+ m₂(L - d)² = m₁d²+ m₂(m₁/m₂)²d² = (m₁/m₂)Md².
  The ' = d/dt denotes the derivative with respect to t.
  Constraint: Y = -d cosθ, Y' = d sinθ θ'.
  T = ½MX'² + ½(Md²sin²θ + I)θ'².   U = -Mgd cosθ.
  L = ½MX'² + ½(Md²sin²θ + I)θ'² + Mgd cosθ.
  
  (c)  If we orient our coordinate system as shown in the figure, then only the expression for the potential energy changes.
  
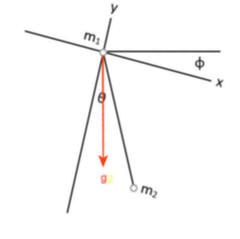
  The X-component of the acceleration of the CM will be constant, a_X = g sinφ, F_X = -∂U/∂X  = Mg sinφ.
  F_Y = -∂U/∂Y  = -Mg cosφ.  U = -Mg sinφ X + Mg cosφ Y = -Mg sinφ X - Mg cosφ d cosθ.
  L = ½MX'² + ½(Md²sin²θ + I)θ'² + Mg sinφ X + Mg cosφ d cosθ.

Problem 3:

A particle of mass m₁ hangs from a rod of negligible mass and length l, whose support point consists of another particle of mass m₂ that moves horizontally subject to two springs of spring constant k each, as shown.
Consider only motion in the plane shown and only small displacements from equilibrium.

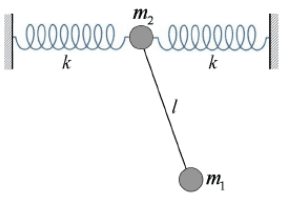
Write down the Lagrangian for this system.

Solution:

• Concepts:
  Lagrangian mechanics
• Reasoning:
  We are asked to find the Lagrangian for the system.
• Details of the calculation:
  L = T - U.  T = ½m₁((dx₁/dt)² +(dy₁/dt)²) +  ½m₂(dx₂/dt)².
  x₁ = x₂ + lsinθ, y₁ = -lcosθ.   dx₁/dt = dx₂/dt + lcosθ dθ/dt, dy₁/dt = lsinθ(dθ/dt).
  T = ½(m₁ + m₂)(dx₂/dt)² + ½m₁l²(dθ/dt)² + m₁(dx₂/dt)lcosθ(dθ/dt),
  U = kx₂² - mglcosθ.
  For small displacements sinθ ~ θ, cosθ ~ 1 - θ²/2.
  Keeping only terms to second order in the Lagrangian we have
  L = ½(m₁ +m₂)(dx₂/dt)² + ½m₁l²(dθ/dt)² + m₁(dx₂/dt)l(dθ/dt) - kx₂² - m₁glθ²/2.
  Let q₁ = lθ, q₂ = x₂.
  L = ½(m₁ +m₂)(dq₂/dt)² + ½m₁(dq₁/dt)² + m₁(dq₂/dt)(dq₁/dt) - kq₂² - (m₁g/l)q₁²/2.