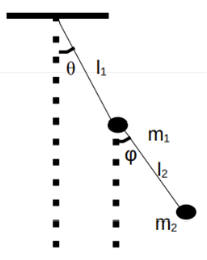
 (a) For the system shown, consider only motion in the vertical plane.
(a) For the system shown, consider only motion in the vertical plane.Write the Lagrangian for small displacements from equilibrium of the system in the form
These are problems from the January 2024 qualifying exam. The address topics discussed in Physics 513. Problems 1 - 2 are from part 1 of the exam and problem 3 -4 are from part 2 of the exam.
Two objects are dropped from the top of a cliff of height H. The second object is dropped when the first has traveled a distance D. At the instant when the first object reaches the bottom, what is the distance of the second object above the ground in terms of H and D?
In reference frame S a firecracker explodes and a second firecracker explodes a distance Δx = c*25 ns away and 52 ns later. In another inertial reference frame S', moving with velocity v i with respect to S, the two explosions are measured to occur a distance d' = c*42 ns apart in space. How much time passes between the explosions in frame S'?
Consider a potential barrier with height U and width a.
(a) Which will tunnel more easily through the potential barrier, an electron or
a proton? Why? Assume each particle's kinetic energy is smaller than U.
(b) If the incoming particle's kinetic energy is 32.0 eV, U = 41 eV, and a =
0.25 nm, find the probability that the particle will tunnel through the barrier,
both for the proton and the electron.
 (a) For the system shown, consider only motion in the vertical plane.
(a) For the system shown, consider only motion in the vertical plane.
Write the Lagrangian for small displacements from equilibrium of the system in
the form
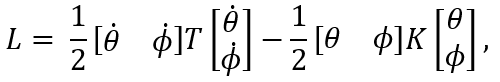
where T and K are matrices. Clearly identify (write out) T and K.
If l1 = l2 = l, and m1 = 21 and m2 =
4 (everything in SI units), find the frequencies
of the normal modes of this system? Briefly describe these modes.