Assignment 9

Problem 1:

In reference frame K a long, straight, neutral wire with a circular cross sectional area A = πr₀² lies centered on the x-axis and carries a current with uniform current density j i. For r > r₀, choose the scalar potential to be Φ = 0 and the vector potential to be A = -[μ₀I/(2π)] ln(r) i.
In a frame K' moving with velocity vi with respect to K, find the ρ', j', Φ' and A' at the point P on the z-axis a distance z = z' > r₀ from the wire.

Solution:

Concepts:
Lorentz transformation of 4-vector current, 4-vector potential and electric and magnetic field
Reasoning:
We are asked to Lorentz transform the 4-vector current density and the 4-vector potential..
Details of the calculation:
In K we have j = ji inside the wire, j = 0 outside the wire, ρ = 0 everywhere.
At point P on the z-axis A = -[μ₀I/(2π)] ln(z) i.
In a frame K' moving with velocity vi with respect to K we have
cρ' = γcρ - γβj = -γβj. j_x' = γj inside the wire,
ρ' = j' = 0 outside the wire.
The wire is not neutral in this frame.
To find Φ' and A' at point P, we can transform the 4-vector potential
Φ'/c = -γβA_x = [γ(v/c) μ₀I/(2π)]ln(z'), A_x' = γA_x, A' = -[μ₀γI/(2π])ln(z') i at point P.

Problem 2:

Determine the E and B fields of an electric dipole moving along the z-axis with a velocity v = v k relative to the laboratory using the Lorentz transformation of the fields. The dipole moment in its rest frame is p = p₀ k.

Solution:

Concepts:
The dipole field, the transformation of E and B from one Lorentz frame to another
Reasoning:
In the rest frame of the dipole, with the dipole at the origin,
E(r,t) = (1/(4πε₀)) (1/r³)[3(p∙r)r/r² - p], B(r,t) = 0.
p = p₀ k. We have axial symmetry about the z-axis.
Let us find the components of the electric field parallel and perpendicular to the z-direction.
3(p∙r)r/r² - p = 3p₀cosθsinθ (ρ/ρ) + 3p₀cos²θ k - p₀ k = 3p₀cosθsinθ (ρ/ρ) + p₀(3cos²θ - 1) k
= 3p₀ρz/(ρ² + z²) (ρ/ρ) + p₀(2z² - ρ²)/(ρ² + z²) k.
E(r,t) = (1/(4πε₀)) (1/(ρ² + z²)^5/2)[3p₀ρz (ρ/ρ) + p₀(2z² - ρ²) k].
Details of the calculation:
The laboratory frame is moving with speed v = -v k with respect to the rest frame of the dipole.
We find the fields in the laboratory frame using
E'_|| = E_||, B'_|| = B_||, E'_⊥ = γ(E + v×B)_⊥, B'_⊥ = γ(B - (v/c²)×E)_⊥.
E'_|| = (1/(4πε₀)) (1/(ρ² + z²)^5/2)p₀(2z² - ρ²) k. B'_|| = 0.
z = γ(z' - vt'), ρ = ρ'.
E'_|| = (1/(4πε₀)) (1/(γ²(z' - vt')² + ρ'²)^5/2)p₀(2γ²(z' - vt')² - ρ'²) k.
E'_⊥ = γE_⊥ = (γ/(4πε₀)) (1/(ρ² + z²)^5/2)3p₀ρz (ρ/ρ)
= (γ²/(4πε₀)) (1/(γ²(z' - vt')² + ρ'²)^5/2)3p₀ρ'(z' - vt') (ρ/ρ).
B'_⊥ = -γ(v/c²)×E = -(v/c²)(γ³/(4πε₀))(1/(γ²(z' - vt')² + ρ'²)^5/2)3p₀ρ'(z' - vt') (φ/φ).

Problem 3:

(a) A fast electron (kinetic energy = 5*10^-17Joule) enters a region of space containing a uniform electric field of magnitude E = -i 1000 V/m. The field is parallel to the electron's motion and in a direction such as to decelerate it. How far does the electron travel before it is brought to rest? Neglect radiation losses.
(b) Now assume that the initial velocity of the electron is v = v₀j, perpendicular to the direction of the electric field. Assume that at t = 0 the electron moves through the origin. Find the speed of the electron, its position, and the work done by the field on the electron as a function of time.
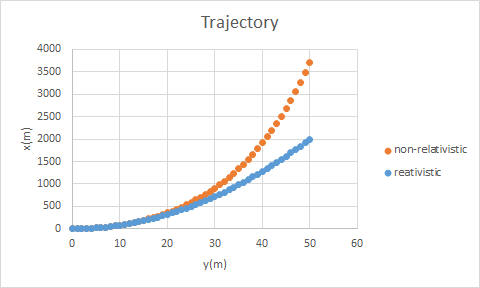
(c) Find the trajectory of the electron, x(y).

Solution:

Concepts:
F = dp/dt, p = γmv.
Reasoning:
The electron is acted on by a constant force. We neglect radiation losses.
Details of the calculation:
(a) E = E i, q = -1.6*10^-19 C.
dp_x/dt = qE, dp_y/dt = dp_z/dt = 0.
p_x(t) = p₀ + qEt, p_y(t) = p_z(t) = 0.
Note: both q and E are negative, so qE is positive.
This part of the problem can be treated non-relativistically,
since 5*10^-17J << mc² = 0.511 MeV * 1.6*10^-13 J/MeV.
v_x = v₀ - qEt/m = 0 --> t = v₀m/(qE).
x = v₀t - ½at² = ½mv₀²/(qE) = (5*10^-17J)/(1.6*10^-19 C * 1000 N/C) = 0.3125 m.

(b) dp_x/dt = qE, dp_y/dt = dp_z/dt = 0.
p_z(t) = 0, p_x(t) = qEt, p_y(t) = p₀.
Energy E_t(t) = (m²c⁴ + p²c²)^½ = (E_t0² + (qcEt)²)^½.
E_t0² = m²c⁴ + p₀²c².
Notation: E_t denotes the total energy, E denotes the field strength.
E_t = γmc², p = γmv, v/c = pc/E_t.
v_x(t)/c = cqEt/(E_t0² + (qcEt)²)^½, v_y(t)/c = cp₀/(E_t0² + (qcEt)²)^½.
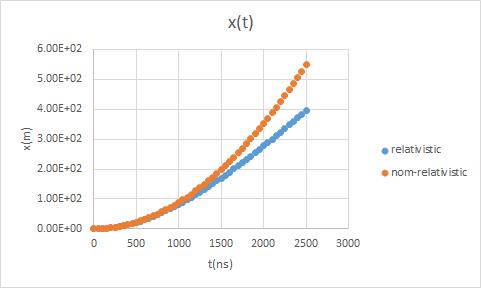
dx/dt = c²qEt/(E_t0² + (qcEt)²)^½, x(t) = (qE)^-1∫₀^qcEt t'dt'/(E_t0² + t'²)^½.
x(t) = (qE)^-1(E_t0² + (qcEt)²)^½ - E_t0/(qE).
dy/dt = c²p₀/(E_t0² + (qcEt)²)^½ = c²p₀E_t0/(1 + (qcEt/E_t0)²)^½.
y(t) = (c²p₀/E_t0)∫₀^u dt'/(1 + t'²)^½, with u = qcEt/E_t0.
y(t) = (p₀c/(qE)) sinh^-1(qcEt/E_t0).
The work done on the electron by the field is
W(t) = (E_t0² + (qcEt)²)^½ - E_t0.
(c) Express t in terms of y(t): sinh(qEy/(p₀c)) = qcEt/E_t0.
x(t) = E_t0(qE)^-1(1 + (qcEt/E_t0)²)^½ - E_t0/(qE).
x(y) = [E_t0/(qE)][(1 + sinh²(qEy/(p₀c)))^½ - 1] = [E_t0/(qE)] [(cosh(qEy/(p₀c)) - 1].
E_t0 = mc² + T = 8.18*10^-14 J, qE = 1.6*10^-16 N, E_t0/(qE) = 511 m,
p₀c = 2.86*10^-15 J, qE/(p₀c) = 0.056/m.
x(y) = 511 m (cosh(y*0.056/m) - 1).

Problem 4:

A reference frame K' is moving with uniform velocity v = vi with respect to reference frame K.
(a) In K, a plane wave with angular frequency ω is traveling in the i direction. What is its frequency in K'?
(b) In K, a plane wave with angular frequency ω is traveling in the j direction. What is its frequency in K'?
(c) In K, a plane wave with angular frequency ω is traveling in a direction making an angle of 45^o with respect to the i direction and the j direction. What is its frequency in K'?

Solution:

Concepts:
The relativistic Doppler shift
Reasoning:
Given the angular frequency ω of a plane wave in reference frame K we are asked to find the angular frequency ω' in reference frame K' moving with constant velocity with respect to K.
Details of the calculation:
(a) ω' = ω[(1 - v/c)/(1 + v/c)]^½ if k and v are parallel to each other.
(b) ω' = γω if k and v are perpendicular to each other. γ = (1 - v²/c²)^-½.
There is a transverse Doppler shift, even if θ = π/2.
(c) ω' = γ(ω - v·k) = γω(1 - vcosθ/c) = γω(1 - v/(√2c)).