More Problems

Problem 1:

A point charge q rests at the origin.  
A natural choice of potentials for this static problem is Φ(r,t) = q/(4πε₀r), A(r,t) = 0.  Find a gauge transformation that results in Φ'(r,t) = 0.
What is A'(r,t)?

Solution:

• Concepts:
  Gauge transformations
• Reasoning:
  A and Φ are not unique.
  A --> A + ∇λ, Φ --> Φ - ∂λ/∂t is called a gauge transformation.
• Details of the calculation:
  Φ' = Φ - ∂λ/∂t = q/(4πε₀r) - ∂λ/∂t = 0.
  Possible λ:  λ = qt/(4πε₀r). 
  ∇λ = e_r [qt/(4πε₀)]∂r^-1/∂r =  -e_r [qt/(4πε₀r²)].
  A' = A + ∇λ =  -e_r [qt/(4πε₀r²)].

Problem 2:

A thin wire of radius b is used to form a circular wire loop of radius a (a >> b) and total resistance R.
The loop is rotating about the z-axis with constant angular velocity ωk in a region with constant magnetic field B = B₀i. 

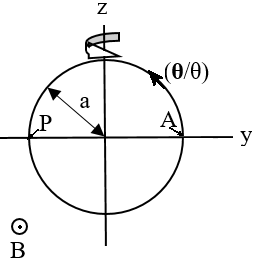
At t = 0 the loop lies in the y-z plane and the points A and P cross the y-axis.
Find the potential difference between points A and P as a function of time.

Solution:

• Concepts:
  Faraday's law, conservative and non-conservative electric fields.
• Reasoning:
  We can find the induced electric field.  That is the only electric field present.  An induced field is non-conservative.
  The potential difference between points A and P is zero for all t.

Problem 3:

A thin wire of radius b is used to form a circular wire loop of radius a (a >> b) and total resistance R.
The loop is rotating about the z-axis with constant angular velocity ωk in a region with constant magnetic field B = B₀i. 
At t = 0 the loop lies in the y-z plane and the point A at the center of the wire crosses the y-axis.

 

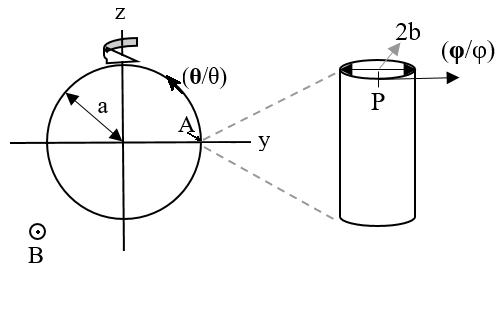
Let the (θ/θ) direction be tangential to the loop and be equal to the positive z direction at point A.
Let the (φ/φ) direction be tangential to the wire and be equal to the direction indicated in the figure.
(a)  Find the current flowing in the loop.  Neglect the self-inductance of the loop.  What is current density J as a function of time?
(b)  Find the thermal energy generated per unit time, averaged over one revolution.
(c)  Write down an expression for the the Poynting vector S on the surface of the wire.
(d)  Use S to find the field energy per unit time flowing into the wire, averaged over one revolution.

Solution:

• Concepts:
  The Poynting vector, energy conservation
• Reasoning:
  The Poynting vector represents the energy flux in the electromagnetic field.  The energy can circulate or flow into an object.  If it flows into an object and is absorbed, energy conservation requires that the field energy is converted into another form of energy.
• Details of the calculation:
  (a)  Flux Φ = B∙A = B₀πa² cos(ωt).  (Define the normal to the area by the right-hand rule.)
  emf = -dΦ/dt = ωB₀πa² sin(ωt),  I = ωB₀πa² sin(ωt)/R.  At t = 0 + dt the current at point A flows in the z-direction.
  The current density in the wire is J = (θ/θ) (a²/b²)ωB₀ sin(ωt)/R.
  
  (b)  P = I²R = ω²B₀²π²a⁴ sin²(ωt)/R.  
  <P> = ½ω²B₀²π²a⁴/R is the thermal energy generated per unit time, averaged over one revolution.
  
  (c)  E_wire = (θ/θ)ωB₀πa² sin(ωt)/(2πa) = ½ωB₀a sin(ωt)(θ/θ). 
  B_wire = μ₀I/(2πb)(φ/φ).  B = B₀ + B_wire.
  S = (1/μ₀)(E×B) = (1/μ₀)(E_wire×B_wire) + (1/μ₀)(E_wire×B₀).
  (E_wire×B_wire) always points towards the center of the wire.
  Let us call this direction the -(ρ/ρ) direction.
  Field energy flowing into the wire per unit time per unit length:
  -∫₀^2πS∙(ρ/ρ) bdφ = (1/μ₀)|E_wire×B_wire|2πb = ω²B₀²π²a⁴ sin²(ωt)/(2πaR).
  [(1/μ₀)(E_wire×B₀) has the same direction all along a circumference of the wire and therefore there is no net flux into the wire due to this term.]
  Total field energy flowing into the wire per unit time
  2πa(1/μ₀)|E_wire×B_wire|2πb = ω²B₀²π²a⁴ sin²(ωt)/R.
  Averaged over one revolution:  ½ω²B₀²π²a⁴/R = <P>.