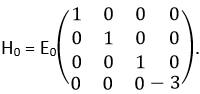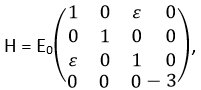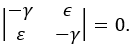Problem 1:
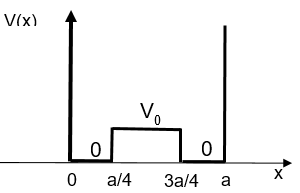
Consider the perturbed 1D infinite well, V(x) = 0 for 0 < x < a/4 and 3/4 < x <a,
V(x) = V0 for a/4 < x < 3a/4,
V(x) infinite everywhere else.
Solve for the energy eigenvalues of excited state with E >> V0 of this perturbed well using the WKB
approximation.
Solution:
- Concepts:
The WKB approximation
- Reasoning:
In one dimension, if V(x) finite everywhere, then the WKB approximation
requires
∫cylcepdx = ∫cylceħkdx
= (n - ½)h, n = 1, 2, ... .
Here V(x) is infinite everywhere except in some finite region and ∫cylcepdx =
∫cylceħkdx
= nh.
- Details of the calculation:
For stationary bound states we want ∫xminxmax
pdx = nh/2, n = 1, 2, ... .
For this problem k2 = k12 =
(2m/ħ2)E for 0 < x < a/4 and 3/4 < x <a,
and k2 = k22 =
(2m/ħ2)(E - V0) for a/4 < x < 2a/4.
∫0a ħkdx = nh/2 = nπħ. ∫0a/4
(2mE)½dx + ∫3a/4a
(2mE)½dx +∫a/43a/4 (2m(E-V0))½dx =
nπħ.
(2mE)½a/2 + (2m(E - V0))½a/2 = nπħ.
E/4 + (E - V0)/4 + (E(E - V0))½/2 = n2π2ħ2/(2ma2)
= n2E0.
E/2 - V0/4 + (E/2)(1 - V0/E)½ ≈ E/2 - V0/4
+ E/2 - V0/4 = E - V0/2 = n2E0.
since E >> V0.
E = n2E0 + V0/2,
keeping only first order terms in the expansion.
Problem 2:
Suppose the potential energy between an electron and a proton had a term U0(a0
/r)2 in addition to usual electrostatic potential energy -e2/r,
where e2 = qe2/(4πε0).
To the first order in U0, where U0 = 0.01 eV, by how much
would the ground state energy of the hydrogen atom be changed?
Solution:
- Concepts:
First order perturbation theory for non-degenerate states
- Reasoning:
The ground state of the hydrogen atom is non-degenerate (neglecting spin). We
add the perturbation H' = U0(a0 /r)2.
- Details of the calculation:
The ground state wave function of the hydrogen atom is ψ(r) = (πa03)-½exp(-r/a0).
The first order correction to the ground state energy is
E01 = [4πU0a02/(πa03)]
∫0∞dr r2 exp(-2r/a0) (1/r2)
= 2U0.
The ground state energy is shifted up by 0.02 eV.
Problem 3:
Consider a system with a 4-dimensionl state space. The Hamiltonian of
the system is H0. The normalized eigenbasis of H0 is
{|1>, |2>, |3>, |4>}. In that basis the matrix of H0 is
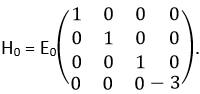
The system is perturbed and the Hamiltonian becomes
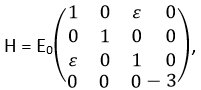
with ε << 1.
Use perturbation theory to find the first order corrections to the eigenvalues
and the zeroth order eigenstates of H.
Solution:
- Concepts:
Stationary perturbation theory for degenerate states
- Reasoning:
The eigenvalue E0 is 3-fold degenerate.
- Details of the calculation:
H = H0 + H'.
The eigenvalues of H0 are E0 with eigenvectors |1>, |2>,
|3> and -3E0 with eigenvector |4>.
The eigenvectors |2> and |4> are also eigenvectors of H with eigenvalue E0
ad -3E0 respectively.
We now diagonalize the perturbation H' in the subspace spanned by |1> and |3>.
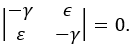
γ2 - ε2 = 0, γ = ±ε. The first order corrections to
the eigenvalues are εE0 and -εE0.
The corresponding eigenstates are 2-½(|1> + |3>) and 2-½(|1>
- |3>) respectively.
Problem 4:
Do a variational calculation. A particle of mass M is subjected to a
potential energy function of the form U = infinite for x < 0 and U = bx
for x > 0
Let the trial wave function be ψ(x) = N x exp(-cx), where "c" is a
variational parameter. Using this function, construct a best estimate of the ground state
energy.
Solution:
- Concepts:
The variational method
- Reasoning:
We are asked to use the
variational method to estimate the ground state energy.
-
Details of the calculation:
H = p2/(2m) + bx, x > 0.
The eigenfunctions of H must be zero at x = 0 and x = ∞. The derivative dψ/dx must be
continuous at all x except x = 0. Any function that
satisfies these boundary conditions can be written as a linear combination
of eigenfunctions of H and is an acceptable trial function giving <H> > E0.
We choose
ψ(x) = N x exp(-cx), N2∫0∞ x2 exp(-2cx) dx = N2/(4c3)
= 1, N2 = 4c3.
Here c is the adjustable parameter.
<H> = N2∫0∞ x exp(-cx)[(-ħ2/2m)(∂2/∂x2)
+ bx]x exp(-cx) dx
= N2(-ħ2/2m)∫0∞
(-2cx + c2x2) exp(-2cx) dx + N2b∫0∞ x3 exp(-2cx)
dx
= N2(ħ2/4mc
- ħ2/8mc
+ 6b/(2c)4).
d<H>/dc = 0 --> c3 = 3bm/(2ħ2).
Insert c back into the equation
for <H>.
E = 1.96 b(2/3)ħ(2/3)/m(1/3).
Exact solutions:
E = 1.85 F(2/3)ħ(2/3)/m(1/3).