More Problems

Problem 1:

Consider a ³He atom (composed of a nucleus of spin 1/2 and an electron).  The electronic angular momentum is J = L + S, where L is the orbital angular momentum of the electron and S is its spin.  The total angular momentum of the atom is F = J + I, where I is the nuclear spin.  The eigenvalues of J² and F² are j(j + 1)ħ² and f(f + 1)ħ² respectively.
(a)  What are the possible values of the quantum numbers j and f for a ³He atom in the ground state?
(b)  What are the possible values of the quantum numbers j and f for an excited ³He atom with one electron is in the 2p and the other in the 4f  state?

Solution:

• Concepts:
  Addition of angular momenta
• Reasoning:
  We are asked to add three angular momenta
• Details of the calculation:
  (a)  For the (1s)² ground state we have L = 0, S = 0, J = L + S = 0.
  F = J + I, i = ½.
  There is only possible values for f, are f = ½.
  (b)   For the excited ³He atom we have l₁ = 1, l₂ = 3.
  The possible values for l are 4, 3, and 2.
  The possible values for s are 0 and 1.
  The possible values for j are j = 5, 4, 3, 2, 1.
  F = J + I, i = ½.
  So the possible values for f are f = 11/2, 9/2, 7/2, 5/2, 3/2, ½.

Problem 2:

Consider a composite system made of two spin ½ particles.  For t < 0 the Hamiltonian does not depend on time and can be taken to be zero.  For t > 0 the Hamiltonian is given by  H = (4C/ħ²)S₁∙S₂, where C is a constant.  Suppose that the system is in the state |+-> for t ≤ 0.  Find, as a function of time, the probability for being in each of the states |++>, |+->, |-+>, and |-->.

Solution:

• Concepts:
  The state space of two spin ½ particles
• Reasoning:
  S₁∙S₂ = ½(S² - S₁² - S₂²).  The eigenstates of S₁∙S₂ are the singlet state and the triplet states, {|S, M_s>}, S = 0, 1.  These are common eigenfunctions of S² and S_z.
• Details of the calculation:
  (a)  The common eigenfunctions of S² and S_z are
  |1,1> = |++>,
  |1,0> = ½^½(|+-> + |-+>),
  |1,-1> = |-->,
  |0, 0> = ½^½(|+-> - |-+>).
  Therefore |+-> = ½^½(|0,0> + |1,0>), |-+> = ½^½(|0,0> - |1,0>).
  For the singlet state we have S₁∙S₂|0,0> = -(3/4)ħ²,  H|0,0> = -3C|0,0>.
  For the triplet states we have S₁∙S₂|1, M_s> = (1/4)ħ²,  H|1, M_s> = C|1, M_s>.
  |ψ(0)> = |+-> = ½^½(|0,0> + |1,0>),
  |ψ(t)> = U(t, 0)|+-> = 2^-½(exp(i3C*t/ħ)|0,0> + exp(-iC*t/ħ)|1,0>).
  P(t) of being in the state |++> = |1 1> = 0.
  P(t) of being in the state |--> = |1 -1> = 0.
  P(t) of being in the state |+-> = |<+-| ψ(t)>|².
  <+-|ψ(t)> = ½(<0,0| + <1,0|)(exp(i3C*t/ħ)|0,0> + exp(-iC*t/ħ)|1,0>)
  = ½(exp(i3C*t/ ħ) + exp(-iC*t/ħ))
  = ½exp(iC*t/ ħ) (exp(i2C*t/ħ) + exp(-i2C*t/ħ)) = exp(iC*t/ħ) cos(2C*t/ħ).
  P(t) of being in the state |+-> = cos²(2C*t/ħ).
  For 2C*t/ħ << 1, P(t) ~ 1.
  P(t) of being in the state |-+> = sin²(2C*t/ħ), since the total probability of being in one of the 4 basis states must be equal to 1.
  For 2C*t/ħ << 1, P(t) ~ (2C*t/ħ)².

Problem 3:

Linearly polarized light of the form E_x(z,t) = E₀e^i(kz-ωt) is incident normally onto a nonmagnetic material which has index of refraction n_R for right-hand circularly polarized light and n_L for left-hand circularly polarized light.
Using Maxwell's equations to calculate the intensity and polarization of the reflected light.

Solution:

• Concepts:
  Maxwell's equations, boundary conditions for the electric and magnetic fields
• Reasoning:
  The intensities of the reflected and transmitted waves are proportional to the squares of the corresponding electric field amplitudes.  We find the ratio of these amplitudes to the incident amplitude by applying boundary conditions.
• Details of the calculation:
  (a)  For a dielectric-dielectric interface Maxwell's equation yield the boundary conditions
  (E₂ - E₁)∙t = 0, and (H₂ - H₁)∙t = 0.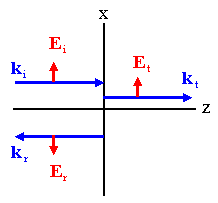

  
  If we set up the problem as shown in the figure above the boundary conditions yield
  (E₂ - E₁)∙t = 0.  (E₂ - E₁)∙(x/x) = 0.  E_i - E_r = E_t.
  (H₂ - H₁)∙t = k_f∙n, (H₂ - H₁)∙(y/y) = 0, there are no free surface currents.
  H₂ = B₂/μ₀.  H₁ = B₁/μ₀.
  B = (1/v)(k/k)×E.  B_i = (y/y)E_i(n₁/c), B_r = (y/y)E_i(n₁/c), B_t = (y/y)E_t(n₂/c).
  (n₁/μ₀)(E_i + E_r)  = (n₂/μ₀)E_t.
  Inserting E_t from above and setting n₁ = 1 and n₂ = n we obtain (E_i + E_r)  = n(E_i - E_r).
  E_r/E_i = (n - 1)/(n + 1) = r₁₂.  r₁₂ is the reflection coefficient.
  The reflectance or ratio of the reflected to the incident intensity is R = <S_r>/<S_i> = |r₁₂|² = [(1 - n)/(1 + n)]².
  
  The total electric field of the incident light has two components. 
  E =  E_R + E_L = (E/2)(i + exp(iπ/2)j) +  (E/2)(i + exp(-iπ/2)j) = (E/2)(i + i j) +  (E/2)(i - i j).
  Since we have normal incidence E_r/E_i = -(1 - n)/(1 + n) holds for each component.
  E_r = -(E/2)(1 - n_R)/(1 + n_R)(i + i j) - (E/2)(1 - n_L)/(1 + n_L)(i - i j).
  |E_r|² = E_r∙E_r* =  (E²/4)[2[(1 - n_R)/(1 + n_R)]² + 2[(1 - n_L)/(1 + n_L)]²].
  (The [(1 - n_R)/(1 + n_R)][(1 - n_L)/(1 + n_L)] terms cancel.)
  R = ½[(1 - n_R)²/(1 + n_R)² + (1 - n_L)²/(1 + n_L)²].