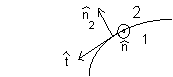 (D2 -
D1)·n2 = σf,
(D2 -
D1)·n2 = σf,(B2 - B1)·n2 = 0,
(E2 - E1)·t = 0,
(H2 - H1)·t = kf∙n.
Consider two angular momentum operators J1 and
J2.
J1 operates in E1 and J2 operates in E2.
Let J = J1 + J2.
J operates in
E = E1⊗
E2.
Since the operators J12, J1z , J22,
and J2z all commute, a basis of common eigenvectors for
E exists. We denote this basis by {|j1,j2;m1,m2>}.
Since the operators J12, J22, J2,
and Jz all commute, a basis of common eigenvectors for
E exists. We denote this basis by {|j1,j2;j,m>}.
We can write the vectors of one basis as linear combinations of the vectors of
the other basis.
|j1,j2;j,m> = ∑m1∑m2 Cjm1m2|j1,j2;m1,m2>,
|j1,j2;m1,m2> = ∑j∑m
Cjm1m2|j1,j2;j,m>.
The Cjm1m2 = <j1,j2;m1,m2|j1,j2;j,m> are called the
Clebsch-Gordon
coefficients.
Properties of the Clebsch-Gordan coefficients
Cjm1m2 = real, Cjm1m2 = 0 unless
m1 + m2 = m and |j1 - j2| ≤ j ≤ |j1
+ j2|.
Cjm1=j1 m2=j2 = 1 (stretched case).
Let Es = Es(1)⊗Es(2)
be the state space of a system of two spin ½ particles.
The tensor product vectors {|++>, |+->, |-+>, |-->} form a basis for
Es.
In the four dimensional state space the operators Siz are product operators.
S1z = S1z(1)
⊗ I(2), S2z = I(1)
⊗ S2z(2),
etc.
The common eigenvectors of S2 = (S1 + S2)2
and Sz = S1z + S2z also form a basis of
Es, which we denote by {|S,Sz>},
where s(s + 1)ħ2
denotes the eigenvalue of S2 and msħ denotes the eigenvalue of Sz.
We have the singlet state
|00> = 2-½(|+-> - |-+>)
and the triplet states
|11> = |++>,
|10> = 2-½(|+-> + |-+>),
|1-1> =|-->.
Review
Maxwell's equations!
In lih materials in regions with ρf =
0 and jf = 0 both
E and
B satisfy the wave
equation.
∇2E - με∂2E/∂t2
= 0, ∇2B - με∂2B/∂t2
= 0.
Plane wave solutions E = E((k/k)∙r)
- vt, B = B(k/k)∙r
- vt) exist.
Here k/k is the unit vector pointing in the direction of
propagation.
We have E ⊥ B,
E
⊥ k, B ⊥
k,
B = (με)½(k/k)×E.
Sinusoidal plane waves are of the form E =
E0ei(k∙r
- ωt). B = B0ei(k∙r
- ωt),
with
k2 = μεω2.
In regions with ρf = 0 and jf
= σcE both
E and
B satisfy the damped wave equation.
∇2E - μσc∂E/∂t
- με∂2E/∂t2
= 0, ∇2B - μσc∂B/∂t
- με∂2B/∂t2
= 0.
Plane wave solutions exist. For sinusoidal plane waves we now have
k2 = iμσcω
+ μεω2
= με(ω)ω2, i.e. k is complex, the wave damps out.
ε(ω) and the
index of refraction
are complex numbers.
The skin depth is defined as the distance it takes to reduce the amplitude by a factor of 1/e.
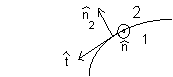 (D2 -
D1)·n2 = σf,
(D2 -
D1)·n2 = σf,
(B2 -
B1)·n2 = 0,
(E2 - E1)·t = 0,
(H2 - H1)·t =
kf∙n.
These are the boundary conditions the electromagnetic fields have to satisfy on any
boundary.
For waves propagating across a dielectric-dielectric boundary or
from a dielectric into a conductor we use that the tangential components of
E
and H are continuous across the boundary.
From these boundary
conditions we derive the law or reflection, θi
= θr, and the law of
refraction, (Snell's law), n1sinθi
= n2sinθt, for a wave propagating from a medium with index of refraction n1 into
a medium with index of refraction n2. Here θi,
θr, and θt are the angles the incident, reflected, and transmitted wave vectors make
with the normal to the boundary.
The plane of incidence is the plane containing the wave vector ki
and the normal to the boundary.
A linear polarized wave has p-polarization if
E lies in the plane of incidence, and it has s-polarization if
E
is perpendicular to the plane of incidence.
The reflection coefficients
for s and p polarization are
r12s
= sin(θ1 - θ2)/sin(θ1 + θ2),
r12p = tan(θ1 - θ2)/tan(θ1 + θ2).
The reflectance is R = |r12|2.
The transmittance is T = 1 - R if there is no absorption (energy conservation).
When θi = θB,
the Brewster angle, then only light with s-polarization is reflected.
We
have tan θB = n2/n1,
θt + θi
= π/2.
If n2 < n1, then for θi > θc = sin-1(n2/n1) we have total internal reflection. The wave vector kt then has a component parallel to the interface which is real and a component perpendicular to the interface which is imaginary.
For good conductors R ≈ 1, T ≈ 0. The wave does not penetrate into the conductor. We then use as the boundary conditions that the tangential component of E and the normal component of B are continuous.
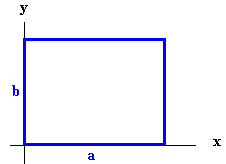 Electromagnetic waves can propagate in a conducting channel.
Electromagnetic waves can propagate in a conducting channel.
If we have a rectangular channel as shown in the figure, with its axis
in the z-direction, than any wave propagating into the z-direction can be viewed
as a superposition of waves with either the electric or the magnetic field
vector lying in the x-y plane. These waves are called transverse electric
(TE) or transverse magnetic (TM) waves.
E and B have to satisfy boundary conditions on the conducting
walls of the channel.
E∙t
= 0 and B∙n
= 0 on the walls.
A TE wave is a superposition of waves of the form
E = E0ysinkxx
exp(i(kzz - ωt)) j + E0xsinkyy
exp(i(kzz - ωt)) i,
with kxa = nπ,
kyb = mπ,
with m and n positive integers. One of these integers, n or m, may be
zero.
These waves are called the TEmn modes of the waveguide.
For each mode there exists a cutoff frequency ωc
= ((nπc/a)2 + (mπc/b)2)½.
Waves with ω
< ωc
do not propagate in the waveguide.
If a > b then the cutoff frequency for
all TE modes is ωc = nπc/a.
Phase velocity: The phase velocity vp is the velocity
with which planes of constant phase move in the z-direction.
vp = ω/kz > c.
Group velocity: The group velocity vg is the velocity
with which the energy moves in the z-direction.
We have vg < c, vpvg = c2.
A TM wave is a superposition of waves of the form
B = B0xsinkxx
exp(i(kzz - ωt)) j + B0ysinkyy
exp(i(kzz- - ωt)) i,
with kxa = nπ,
kyb = mπ,
with m and n nonzero, positive integers.
These waves is called the TMmn modes of the
waveguide.
TM10 or TM01 guided waves do not exist.
They cannot satisfy ∇∙B
= 0.
| Energy density in EM fields: | u = ½ε0E2 + (1/(2μ0))B2 |
| EM waves, energy flux: | S = (1/μ0)E×B |
| Radiation pressure: | P = (1/c)S |
| Polarizers: | Itransmitted = I0cos2θ. |
| Geometrical Optics | |
| Law of refraction: | n1sinθ1 = n2sinθ2 |
| Mirror equation: | 1/xo + 1/xi = 1/f, M = -xi/xo, f = R/2 |
| Lens equation: | 1/xo + 1/xi = 1/f, M =- xi/xo |
| Wave Optics | |
| Double slit, diffraction grating (maxima): | dsinθ = mλ, m = 0, 1, 2, ..., λ ≈ zd/(mL) |
| Single slit, (minima): | wsinθ = mλ, λ ≈ zw/(mL) |
| Resolving power: | θmin = 1.22 λ/D |
| Constructive interference (thin oil film on water): | 2noilt cosθt = (m + ½)λ, m = 0,1,2,... |