Assignment 8
Problem 1:
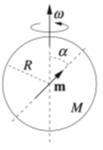 A pulsar is a neutron
star with mass M ≈ 1.4*Msun ≈ 2.8 × 1030 kg and radius R ≈
10 km. The star rotates with angular velocity ω and has a magnetic moment m,
which is, in general, not parallel to the rotation axis.
A pulsar is a neutron
star with mass M ≈ 1.4*Msun ≈ 2.8 × 1030 kg and radius R ≈
10 km. The star rotates with angular velocity ω and has a magnetic moment m,
which is, in general, not parallel to the rotation axis.
(a) Describe the radiation emitted by the pulsar, and find the total radiated
power, assuming that the angle between the magnetic moment and the rotation axis
is α, as in the figure.
(b) Find the "spin-down rate" (i.e. find ω(t)) of the pulsar, assuming that
energy loss is due to radiation only.
Solution:
- Concepts:
Magnetic dipole radiation
- Reasoning:
Treat the neutron star as a rotating magnetic dipole with dipole moment m.
The rotating magnetic dipole will radiate energy at a rate Prad =
<(d2m/dt2)2>/(6πε0c5).
- Details of the calculation:
(a) Let the rotation axis be the z-axis.
m(t) = m0 cosθ k + m0 sinθ (cos(ωt) i +
sin(ωt) j), where θ is tilt angle.
d2m/dt2 = -ω2m0 sinθ [cos(ωt) i +
sin(ωt) j].
<(d2m/dt2)2> = ω4m02sin2θ,
Prad = ω4m02sin2θ/(6πε0c5).
(b) The rotational kinetic energy of the neutron star is ½Iω2
= (1/5)MR2ω2.
Prad(t) = -dE/dt = -(d/dt)((1/5)MR2ω2) =
-(2/5)MR2ω dω/dt.
ω4m02sin2θ/(6πε0c5)
= -(2/5)MR2ω dω/dt.
-5 m02sin2θ dt/(12 MR2πε0c5)
= dω/ω3.
[-5 m02sin2θ /(12 MR2πε0c5)]
∫0t dt = ∫ω(0) ω(t)dω/ω3.
[5 m02sin2θ /(12 MR2πε0c5)]
t = ½(ω(t)-2 - ω(0)-2).
5 m02sin2θ t/(6 MR2πε0c5)
= 1/ω(t)2 - 1/ω(0)2.
1/ω(t)2 = 1/ω(0)2 + 5 m02sin2θ
t/(6 MR2πε0c5).
ω(t)2 = ω(0)2/(1 + 5 m02sin2θ
ω(0)2t/(6 MR2πε0c5)).
ω(t) = ω(0)/(1 + t/τ)½, with
τ = 6 MR2πε0c5/(5 m02sin2θ
ω(0)2).
Problem 2:
A spherical shell of
radius R carrying a uniform surface charge σ is set spinning at angular velocity
ω.
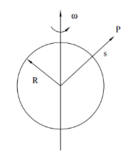
(a) Find the
vector potential A that it produces at point P located at a distance s, s
> R from the center of the sphere.
(b) Find the magnetic field B outside the sphere.
(c) How does the result for A change if r < R?
(d) Find B inside the sphere.
Solution:
- Concepts:
Boundary conditions, the uniqueness theorem
- Reasoning:
One form of the uniqueness theorem for magnetostatics:
If the current density j is specified throughout a volume V and either
A or B are specified at the boundaries of a volume V, then a unique
solution exists for B inside V.
In magnetostatics we choose
∇·A
= 0.
Then ∇2A
= -μ0j, A(r) = (μ0/(4π)) ∫V'dV'
j(r')/|r - r'|. (SI units).
Boundary conditions in magnetostatics:
(B2 - B1)·n2 = 0, (B2
- B1)·t = μ0k·n.
A is continuous across the boundary.
There are different approaches. One is outlined below.
We can solve for the magnetic
field of a rotating spherical shell with uniform surface charge density σ, by
treating the problem as a boundary value problem.
Let the radius of the sphere be R, its center be located at the origin, and let
the sphere rotate with angular velocity ω = ωk.
The rotating surface charge density produces a surface current density, km
= σω x R,
km = σωRsinθ eφ.
For r >> R we will have a dipole potential,
A(r) = (μ0/(4π))m x r/r3, or Aφ
= (μ0/4π)msinθ/r2.
To find the dipole moment m, we consider the rotating sphere to be a
stack of current loops.
For a current loop at θ we have dm = IA = Rσω sinθ Rdθ πR2sin2θ
= R4σωπ sin3θdθ.
Integrating dm from θ = 0 to θ = π we find m = (4/3)R4σωπ, m =
mk.
[∫sin3xdx = -(1/3)cosx (sin2x + 2)]
For r >> R we have Aφ = (μ0/3)R4σωsinθ/r2.
For any r > R assume that A(r) only has φ component.
Assume that the 1/r2 term is the only term in the expansion of A
in powers of 1/rn outside of the sphere, i.e. assume that outside the
sphere the potential is a pure dipole potential.
Since A must be continuous at r = R and A must be finite at the
origin,
assume that Aφ = Csinθr = (μ0/3)Rσωsinθr inside the
sphere and A = Aφeφ.
If this solution fulfills the boundary conditions,
(B2 - B1)·n2 = 0, (B2
- B1)·t = μ0k·n,
we have the only solution.
B = ∇×A,
Bin_r = (1/)rsinθ))∂(sinθAφ_in)/∂θ = (2μ0/3)Rσω
cosθ.
Bin_θ = -(1/r)∂(rAφ_in)/∂r = -(2μ0/3)Rσω sinθ.
Bout_r = (1/(rsinθ))∂(sinθAφ_out)/∂θ = (2μ0/3)R4σω
cosθ/r3.
Bout_θ = -(1/r)∂(rAφ_out)/∂r = (μ0/3)R4σωsinθ/r3.
Now check that the boundary conditions are satisfied.
At r = R the radial component of B is continuous.
Bout_θ - Bin_θ = μ0R4σω sinθ/R3
= μ0km.
The boundary conditions are satisfied and we have the only solution.
The field outside the sphere is a pure dipole field,
B(r,θ,φ) = (μ0/3)R4σω
(2cosθ/r3 er + sinθ/r3 eθ),
and the field inside the sphere is constant, B(r,θ,φ)
= k(μ0/4π)2m/R3.
- Details of the calculation:
(a) Aout (r,θ,φ) = (μ0/3)R4σωsinθ/r2
eφ.
(b) Bout (r,θ,φ) = (μ0/3)R4σω/r3
(2cosθ er + sinθ eθ).
(c)
Ain(r,θ,φ) = (μ0/3)Rσωsinθ r eφ.
(d)
Bin(r) = (μ0/4π)2m/R3
k.
Problem 3:
Assume you have two very small spheres with radius a, made of lih material
with magnetic susceptibility Χm > 0.
The spheres are separated by a distance R >> a .
Assume that the magnetic dipole moment m of each sphere is induced by the
magnetic field due to the magnetic dipole moment of the other sphere.
Choose your coordinate system so that sphere 1 is located at the origin and its
magnetic dipole moment points in the z-direction.
(a) Where do you have to place sphere 2, so that the dipole moments of the
two spheres are aligned?
(b) Where do you have to place sphere 2, so that the dipole moments of the
two spheres are anti-aligned?
Solution:
- Concepts:
The dipole field
- Reasoning:
The magnetic field outside the tiny sphere is a pure dipole field. Since the
spheres are tiny, we can assume that the external magnetic field inside the spheres is
equal to that at the center of the spheres.
For linear, isotropic, homogeneous (lih) magnetic materials we have
M = ΧmH,
B = μ0(H +
M) = μ0(1 + Χm)H = μ0κmH
= μH.
Χm > 0 for paramagnetic materials.
- Details of the calculation:
(a) Assume both spheres have a dipole
moment m = mk. At a distance r, the magnetic field produced by
sphere 1 is
B(r) = (μ0/4π)(3(m·r)r/r5
- m/r3) = (μ0m/(4πr3))[2 cosθ (r/r)
+ sinθ (θ/θ)],
H(r) = (1/4π)(3(m·r)r/r5
- m/r3) = (m/(4πr3))[2 cosθ (r/r)
+ sinθ (θ/θ)].
On the z - axis H(z) = 2m/(4π|z|3).
If sphere 2 is located on the z-axis and the distance between the spheres is d,
then the magnetic field at the location of each sphere is due to the dipole
moment of the other sphere is H(d) = 2m/(4πd3).
We want m = V(3χm/(3 + χm))H(d), with V = 4πa3/3.
Then m = V(3χm/(3 + χm))2m/(4πd3).
Solve for d: d3 = V(6χm/(3 + χm))/(4π).
(b) In the xy plane, a distance ρ from the magnetic field produced by
sphere 1 points in the opposite direction of its magnetic moment.
H(ρ) = -m/(4πρ3) k.
If sphere 2 is located in the xy plane and the distance between the spheres is d,
then the magnetic field at the location of each sphere is due to the dipole
moment of the other sphere is H(d) = -m/(4πd3).
We want m = V(3χm/(3 + χm))H(d), with V = 4πa3/3.
Then m = V(3χm/(3 + χm))m/(4πd3).
(magnitude)
Solve for d: d3 = V(3χm/(3 + χm))/(4π).
Problem 4:
At t = 0 a particle of mass m and charge q moves in the xy-plane in a
circular orbit of radius R in a uniform magnetic field
B = Bk. The particle will loose kinetic energy through
radiation. Assume that the energy loss per revolution is small compared to
the total energy of the particle.
(a) Neglecting radiation, what is the magnitude of the particles momentum
at t = 0? Does your answer depend on the speed of the particle
(relativistic or non-relativistic)?
(b) Now assume the particle is moving non-relativistically. Derive
an expression for the time it takes the particle to loose half of its kinetic
energy.
Solution:
- Concepts:
Motion of a charged particle in a magnetic field, the radiation field of a point charge moving non-relativistically,
the Larmor formula
- Reasoning:
In a magnetic field a moving charged particle is acted on by a force
perpendicular to the direction of its velocity. Therefore this force
changes the direction of the velocity, but not the energy of the particle.
This acceleration results in power loss via radiation. The power
radiated is given by the Larmor formula.
- Details of the calculation:
(a) F = qv×B, dp/dt = γmdv/dt
= γma.
For circular motion a = v2/R pointing towards the center
of the circle.
γmv2/R = qvB, R = γmv/(qB) =
p/(qB). p = RqB.
(b) For a non-relativistic particle mv = rqB, r
= mv/qB.
We assume that the orbital radius r changes very little while
the particle completes one revolution, so that the acceleration is always
given by v2/r.
From the Larmor formula we have
P = (2/3)[q2/(4πε0c3)]a2
= -dE/dt.
a = v2/r = 2E/(mr). Since r = mv/qB,we have
a2 = 2Eq2B2/m3.
dE/dt = -[q4B2/(3π m3ε0c3)]E.
E = E0exp(-t/τ),
where τ = (3πε0c3m3)/(q4B2)
½ = exp(-t/τ), t = τ ln(2) is the time it takes the particle to loose half
of its kinetic energy.
 A pulsar is a neutron
star with mass M ≈ 1.4*Msun ≈ 2.8 × 1030 kg and radius R ≈
10 km. The star rotates with angular velocity ω and has a magnetic moment m,
which is, in general, not parallel to the rotation axis.
A pulsar is a neutron
star with mass M ≈ 1.4*Msun ≈ 2.8 × 1030 kg and radius R ≈
10 km. The star rotates with angular velocity ω and has a magnetic moment m,
which is, in general, not parallel to the rotation axis.