Let E_s(1) denote the two-dimensional state space of particle 1 and E_s(2) the two-dimensional state space of particle 2.  E_s= E_s(1)ÄE_s(2) then is the state space of the system of the two particles. E_s is four-dimensional.

The vectors {|i:+>,|i:->} form a basis for the two-dimensional state space of each particle.  They are eigenvectors of S_iz and S_i². Here i denotes either particle 1 or particle 2.

,

.

The vectors {|++>,|+->,|-+>,|-->}, (where |++>=|1:+>Ä|2:+> etc. ), form a basis for E_s.  Every vector in E_s can be written as a linear combination of these basis vectors.

Let e denote + or -. The we express orthogonality through

,

and completeness through

.

The most general normalized state in E_s is

,

with .

In the four dimensional state space of the two particles S_iz and S_i² are product operators.

  etc.

The matrix of any product operator A(1)ÄB(2) in a basis of tensor product vectors {|i(1)>Ä|j(2)>=|i,j>} is

,

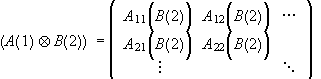where the A_ij are the matrix elements of A in the {|i(1)>} basis of E₁ and is the matrix of B in the {|j(2)>} basis of E₂.

.

The matrix of S_1z in the {|++>,|+->,|-+>,|-->} basis therefore is

.

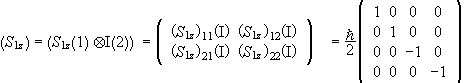The matrix of S_2z is

.

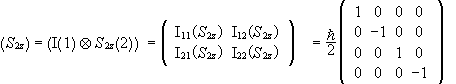The matrix of S₁² and S₂² is

 .

What do we see inspecting these matrices?

The basis vectors {|++>,|+->,|-+>,|-->} are eigenvectors of S_1z, S_2z, S₁², and S₂² in E_s.

The total spin of the two particles is S=S₁+S₂.  What are the eigenvectors of S² and S_z?

.

Any linear combination of basis vectors are eigenvectors of S₁² and S₂².  To find the eigenvectors of S² we therefore have to find the eigenvectors of S₁·S₂.

.

In the {|++>,|+->,|-+>,|-->} basis we have

.

.

.

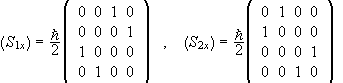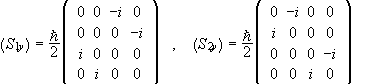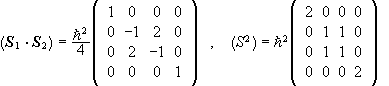What do we see by inspecting these matrices?

The basis vectors are not eigenvectors of S_ix, S_iy, , and S².

The eigenvalues of S² are 0 and . The eigenvalue is 3 fold degenerate.

Any linear combination of the normalized vectors |++>, is an eigenvector with eigenvalue .

The eigenvector with eigenvalue 0 is .

The matrix of S_z=S_1z+S_2z is

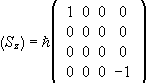
in the {|++>,|+->,|-+>,|-->} basis.

Common eigenvectors of S² and S_z are |++>, and .  Each common eigenvector is uniquely specified by its pair of eigenvalues.  These eigenvectors also form a basis of E_s, which we denote by {|S,S_z>}, where  denotes the eigenvalue of S² and  denotes the eigenvalue of S_z.  We have

,

triplet states

and

.

singlet state

S² and S_z form a C.S.C.O. for the 4-dimensional state space E_s.

Problems:

Consider a pair of non identical particles of spin ½ with angular momenta I₁ an I₂.  Their magnetic moments, m₁=-g₁I₁ and m₂=-g₂I₂ respectively, are subjected to a uniform static magnetic field in the z direction.  The interaction between the particles, which can be written as T(I₁·I₂) is weak compared to the Zeeman interactions.  Ignore kinetic energies.

(a) Write the Hamiltonian for the system.

(b) Work out the matrix form of H, defining the basis states explicitly.

(c) Find the exact expressions for the energies of the eigenstates.

(d) Calculate the state energies using perturbation theory and compare these approximate results with your exact expressions from part c).

• Solution:

  No orbital angular momentum is mentioned, we assume that the total angular momentum of each particle is due to its spin I₁=S₁, I₂=S₂.

  

  .

  • (a)  .
  • (b) In the {|++>,|+->,|-+>,|-->} basis S_1z and S_2z are diagonal, but is not.  In the {|S,S_z>} basis is diagonal but S_1z and S_2z are not. Therefore the matrix of H is not diagonal in either basis.

    Let us choose the {|++>,|+->,|-+>,|-->} basis.  Then

    

    .

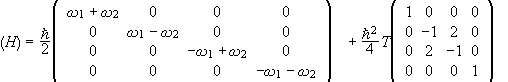
  • (c) .  The l are the eigenvalues of H.  We have (since the matrix is block-diagonal)

    ´

    =0,

    where .  One of the factors in this product has to be zero.

    The eigenvalues are

    ,

    and from

    

    .

  • (d) If H=H₀+W, where W is a small perturbation compared to H, and the set {|f_n>} is a non degenerate eigenbasis of H₀ with eigenvalues {E_n⁰} then to first order in W the eigenvalues of H are {E_n}, where E_n=E_n⁰+<f_n|W|f_n>.

    .

    .

    .

    .

A system of two distinguishable spin ½ particles (S₁ and S₂) are in some triplet state of the total spin, with energy E₀.  Find the energies of the states, as a function of l and d, into which the triplet state is split when the following perturbation is added to the Hamiltonian, V=l(S_1xS_2x+S_1yS_2y)+dS_1zS_2z.

• Solution:

  In the { |S,S_z> }={|1,1>,|1,0>,|1,-1>,|0,0>} basis the matrix of the Hamiltonian H₀ is

  .

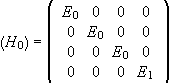  We have H=H₀+V.

  .

  In the { |S,S_z> } the matrix of V is diagonal, since the matrices of are diagonal.

  .

  For the triplet state  .

  For the singlet state  .

  ,

  .

  Therefore we have

    .

  ,

  .

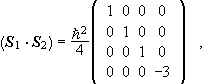
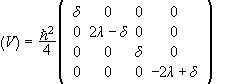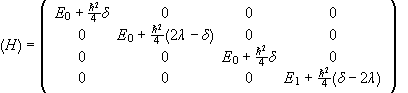  The energies into which the triplet state splits are and . The value is twofold degenerate.