Problem 1:

Define the standard components of a vector operator V as the three operators

.

Using the standard components of the two vector operators V and W we construct the operator

.

where the <1,1;p,q|K,M> are the Clebsch-Gordan coefficients entering into the addition of two angular momenta.

(a)  Show that [V⁽¹⁾ Ä W⁽¹⁾]⁽⁰⁾₀ is  proportional to the scalar product V·W of the two vector operators.

(b)  Show that the three operators [V⁽¹⁾ Ä W⁽¹⁾]⁽¹⁾_M are proportional to the three standard components of the vector operator V´W.

(c)  Express the five components [V⁽¹⁾ Ä W⁽¹⁾]⁽²⁾_M in terms of V_z, V₊=V_x+iV_y, V_-=V_x-iV_y, W_z, W₊=W_x+iW_y, and W_-=W_x-iW_y.

Problem 2:

(a)  Relate, as much as possible, the matrix elements
<n',l',m'|-(2)^-1/2(x+iy)|n,l,m>, <n',l',m'|(2)^-1/2(x-iy)|n,l,m>,
and <n',l',m'|z|n,l,m>, using only the Wigner-Eckart theorem.  Make sure to state under what conditions the matrix elements are non-vanishing.

(b)  Do the same problem using the wavefunctions y(r)=R_nl(r)Y_lm(q,f).

Problem 3:

Consider a neutron interferometer.  

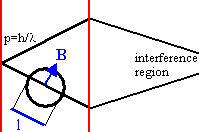
Prove that the difference in the magnetic fields that produces two successive maxima in the counting rates is given by

DB=4phc/(|e|g_nll),

where g_n (= -1.91) is the neutron magnetic moment in units of -eh/(2m_nc).

Problem 4:

The hyperfine splitting in the hydrogen atom can be discussed in terms of a perturbation AI·J, where I and J are the spin angular momentum operators of the proton and electron respectively and A is a constant.  The total angular momentum operator is F=I+J.

(a)  Calculate the expectation value < AI·J >_I,J,F.of the perturbation in the state characterized by the quantum numbers I, J, and F.
(b)  Let DV=B(g_II_z+g_JJ_z). Calculate <DV>_I,J,F.