Consider a spinless particle of mass m in a central potential V(r).  The potential energy of the particle depends only on its distance from the origin.

For a classical particle in a central potential the force  is always directed towards the origin, the torque t=r´F  is zero, and the angular momentum L=r´p is a constant of motion.  The particle's trajectory lies in a plane passing through the origin.  Its velocity v may be decomposed into a component v_r along and a component v_^ perpendicular to in this plane.  The energy of the particle is given by

.

Using p=mv and L=mrv_^ we can write

, 

with

.

To make the transition to quantum mechanics we write down the quantum-mechanical Hamiltonian H of the particle by replacing in the expression for E the classical physical quantities expressed in terms of r and p by their corresponding quantum mechanical operators, symmetrizing suitably, if necessary.  In coordinate representations we have already found expressions for the operators p_x, p_y, p_z, p², and L², but we have not yet found an expression for p_r².

In coordinate representation we write

.

.

See notes!

.

The expression for p_r² in coordinate representation therefore is

.

To find the wave functions y(r,q,f) of the eigenstates of H we have to solve the eigenvalue equation Hy(r)=Ey(r).  For a particle in a central potential the entire angular dependence of H is contained in the L² term.  L² commutes with all components L_i of L.  All other terms of H depend only on r, not on q and f, and commute with L_i.  We have [H,L_i]=0, [H,L²]=0.  The angular momentum L of the particle is therefore a constant of motion.  We can find a common eigenbasis of H, L² and L_z.  We denote the states of this basis by |k,l,m> and the corresponding eigenfunctions by y_klm(r,q,f).  We have

.

We already know the general form of y_klm(r,q,f).  y_klm(r,q,f)=R_kl(r)Y_lm(q,f) is a product of a radial function R_kl(r) and the spherical harmonic Y_lm(q,f).  (See notes!)

Substituting this form of y_klm(r,q,f) into the eigenvalue equation yields the differential equation

for R_kl(r).

Note: Since H commutes with L_± , E cannot depend on m.  E is therefore at least (2l+1) fold degenerate.  Accidental degeneracies may add to this essential degeneracy.

Writing we find a differential equation for u_kl(r), which has a simpler form than the differential equation for R_kl(r).

.

This differential equation has the same form as the one-dimensional Schroedinger equation for a particle moving in an effective potential

.

(Here is one reason why we have spend so much time on 1-d problems.)

But r is not x.  The variable r can take on only non-negative real values.  R(r) has to stay finite at r=0, therefore u(r) has to go to zero at r=0.  Otherwise y(r,q,f) is not an acceptable wave function.  Choosing V_eff(r)=¥ for r<0 lets us treat the radial equation as an ordinary one-dimensional equation while guaranteeing the proper behavior at the origin.

Assume R_kl(r) is sufficiently regular that it may be expanded in powers of r near the origin.

,

where s is the lowest power with a non-zero coefficient.

Therefore

.

Substituting this expression for u_kl(r) into

yields

+ (terms containing powers of r greater than s-1) = 0, if |V(r)|£ C'r^-2 as r®0.

The coefficients of successive powers of r have to vanish independently, since this equation holds for all r.  Letting the coefficient of r^s-1 vanish we obtain -s(s+1)+l(l+1)=0 if |V(r)|< C'r^-2 as r®0, i.e. if |V(r)| does not blow up as fast as r^-2 as r®0.  This is a quadratic equation with two solutions.  It implies that s=l, or that s=-(l+1) if |V(r)|< r^-2 as r®0.  As r®0 the lowest power of r dominates and u_kl(r)®Cr^l+1.  The solution u_kl(r)®Cr^l+1 goes to zero at r=0, but the solution u_kl(r)®Cr^-l blows up at r=0 and is unacceptable.

Near the origin the radial behavior of an acceptable wave function of a particle moving in a central potential is proportional to r^l, (if |V(r)|<C'r^-2). Note: R(r)=u(r)/r.

Consider an isolated system of two interacting spinless particles of masses m₁ and m₂ at positions r₁ and r₂, respectively.  The potential energy of the particles depends only on their relative position r₁-r₂.  In classical mechanics the Lagrangian of such a particle can be written as

,

where M=m₁+m₂ is the total mass of the system, 

is the reduced mass of the system, 

are the coordinates of the center of mass of the system, 

and r=r₁-r₂ are the relative coordinates.  

Using  we define  .  The classical equations of motion are obtained from

.

They are

.

If we take P, R and p, r as the new canonical variables of the system then the Hamiltonian of the system is

.

The first term represents the kinetic energy associated with the motion of the center of mass, which is in uniform rectilinear motion ( ).  The other terms represent the energy associated with the relative motion.  If we choose an inertial frame in which the center of mass is at rest, then  

is the Hamiltonian of a fictitious particle with mass m, whose position is given by the relative coordinate r, moving in an external potential V(r).  The relative motion of two interacting spinless particles reduces to the motion of a single fictitious particle in an external potential.

To make the transition to quantum mechanics we again replace in the expression for H the classical physical quantities expressed in terms of the canonical variables by their corresponding quantum mechanical operators and symmetrize suitably, if necessary.

,

where H, P, p, and r are now operators.  Expressing these operators in terms of p₁, p₂, r₁, and r₂ and using we can show that and that for all i and j.

We therefore have

.

There exists a common eigenbasis of H, H_CM, and H_r.  We may consider the state space E to be the tensor product of E_CM and E_r, E=E_CMÄE_r.  P and R operate only in E_CM and p and r operate only in E_r.  The motion of the center of mass and the relative motion are completely independent of each other.

Let {|c>} be an eigenbasis of H_CM and {|w>} be an eigenbasis of H_r.  Then {|f>=|c>Ä|w>}is an eigenbasis of H in E.

,

.

In coordinate representation we write

,

,

.

We can solve for c(R).

.

The fictitious particle associated with the motion of the center of mass behaves like a free particle and is represented by a plane wave.

We are often only interested in the relative motion, i.e. in the behavior of the two interacting particles in their center of mass frame.  If their mutual interaction depends only on the distance between them, then V(r)=V(r) and the eigenvalue equation becomes

.

Then

.

.

is the radial function of a particle of mass m moving in a central potential.

Problem:

(a) Show that the ground state of the deuteron possesses zero orbital angular momentum (l=0).  Since this is true for any central potential, you may not need the detailed nature of the square well potential.

(b) Assume that l=0 and estimate the value of V₀ under the additional condition that the value of the binding energy is much smaller than V₀.

• Solution:

  (a)  The Hamiltonian of the relative motion of the two particles is

  .

  .

  Let y_l be the lowest energy eigenfunction of H with orbital angular momentum quantum number l.

   .

  Let y_l+1 be the lowest energy eigenfunction of H with orbital angular momentum quantum number l+1.

   .

  .

  

  since y_l is assumed to be the lowest energy eigenfunction of the Hamiltonian .

  Therefore and the ground state possesses zero orbital angular momentum.

  (b)  The relative motion of the two particles is described in the same way as the motion of a fictitious particle of reduced mass m in a central potential.  Therefore we have

  .

  .

  The problem is reduced to a one dimensional "square well problem" with E<0.

  

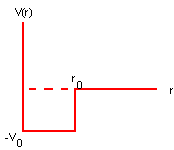
  Let

  .

  Let r<r₀ define region 1 and r>r₀ define region 2.  The coordinate r is never negative.  Therefore

  .

  .

  The boundary conditions are

  .

  .

  ,

  in regions where .

  If we plot we find solutions at the intersections of the two curves in regions where , i.e. .

  For only one solution to exist we need .

  (See QM1, homework 2, problem 3, solutions.)

  If |E|<<|V₀| then .  Then .

  .

  (Rule of thumb: To estimate the range of a force, divide by the mass of the particle that carries it times c, ).

  See notes!